5028
Improving the performance of integrated RF-B0 shim array using 3D coil elements with orthogonal loops1Laboratory of FMRI Technology (LOFT), Mark & Mary Stevens Neuroimaging and Informatics Institute, Keck School of Medicine, University of Southern California, Los Angeles, CA, United States
Synopsis
B0 field inhomogeneity can reduce the effectiveness of RF pulses, result in signal voids, blurring and image artefacts. Integrated RF-shim coil arrays1,2,3 offer capabilities for concurrent parallel RF reception and local B0 shimming, and is superior to spherical harmonic shimming. However, its performance may still be limited due to the lack of flexibility for shimming and limited depth penetration for signal reception. We simulated the B0 shimming performance of a range of integrated RF-shim coil arrays with 3D orthogonal loop coil geometry, and compared the results with standard 2D loop coil arrays.
Introduction
Integrated RF-shim coil arrays1,2,3 offer capabilities for concurrent parallel RF reception and local B0 shimming, also known as AC/DC array technology. However, existing AC/DC arrays have drawbacks of inflexible shim field patterns, limited shim strength and degrees of freedom (the total number of shim loops). In addition, AC/DC arrays with standard 2D loop coil geometry have limited depth penetration for signal reception, especially for a large number of small coils. 3D coil elements with orthogonal X and Y-loops have been proposed to improve SNR and depth penetration as well as spatial sensitivity (g-factor) distribution compared to standard 2D loop coils4,5,6. The 3D coil topology may also provide new degrees of freedom with orthogonal shim arrays that improve B0 shim efficiency compared to existing AC/DC arrays, as demonstrated by Lin F-H et.al7 in which separate orthogonal shimming and reception coil arrays are applied. The goal of this study was to simulate B0 shimming performance of a range of AC/DC arrays with 3D orthogonal loop coil geometry, and to compare the results with standard 2D loop coil arrays.Methods
Simulation was performed using opensource Matlab package and geometries of 2D loop coil arrays with 8, 16, 32, 39(32ch + 7 face loops), 64 and 128 channels3. We designed 2 AC/DC arrays with 3D orthogonal loop geometries: 1) existing 2D loop coil arrays plus vertical rectangular loops aligned paralleled to B0 field8; and 2) existing 2D loop coil arrays plus vertical rectangular loops aligned ±45-degree with the B0 field and also perpendicular to neighboring rectangular loops. These two geometries based on 8, 16 and 32 channel coil arrays are shown in Fig.1. In order to satisfy the requirement of mutual decoupling between orthogonal loops as well as between neighboring loops, we rotated vertical rectangular loops 90 degrees every time on the next coil to decouple the neighbor coils as much as possible while maintaining the 45-degree angle in the meantime. It is expected that rectangular loops paralleled to B0 field are favorable for signal reception but weaker for shimming.Shimming simulation of two 3D and standard 2D geometries of 8-128ch arrays was performed using six B0 field maps acquired on healthy subjects at 3T with 2nd-order spherical harmonic shims applied over the entire brain (ΔTE=2.54ms, 62 slices, 2mm slice thickness, 2.4mm in-plane, FOV=24x24cm)3. In order to calculate the field distribution, each coil including 2D loop coils and orthogonal loops was first approximated with 64 equal length small segments. Then, by applying spatial matrix transform and changing the corresponding angle parameters, these approximated coils were placed into the correct positions around the brain. At last, Biot-Savart solver9 was applied to calculate the overall compensation field strength generated by the coil array in the space.
The optimization process with coil subarray selection using genetic algorithm was performed3. The standard deviation (SD) of simulated B0 field with optimized shimming averaged across 6 subjects was used as the metric comparing shimming performance of 3D and 2D coil arrays.
Results
Fig.2 shows the coil subarray selection results of 8ch, 16ch case where 25% and 50% of the coils are selected, and the shimming results of B0 field maps are shown in Fig.3. The shimming results (SD of B0 field averaged across6 subjects) are shown in Fig.4 with bar plots. It can be observed from Fig.4 that the 3D coil geometry with 45-degree rectangular orthogonal loops yielded better shimming performance in most of the coil arrays tested. The higher performance of 3D arrays compared to 2D arrays is expected since each 3D coil element is composed of 2 separate coils orthogonal to each other while each 2D coil element only contains one coil. Therefore, we also compared the performance between 2D arrays and corresponding 3D arrays with half of the 2D coil number selected for shimming. For 8ch and 16ch arrays, the result of 3D arrays with 45-degree neighbor orthogonal geometry is still superior to that of 2D arrays even with only half of the coils selected. For arrays with coil number higher than 16, 3D arrays have no clear advantage in shimming performance compared to 2D arrays.Discussion & Conclusion
In general, our design of 3D arrays with 45-degree rectangular loops is superior to that with orthogonal loops parallel to B0 field in shimming performance. The reason is that the former design provides more flexibility in signal reception and shimming compared to the latter design, and the alternating ±45-degree design minimizes the potential coupling between neighboring coils. However, when the coil number is larger than 32, we can only get small to little benefits for B0 shimming using 3D arrays in comparison with 2D arrays. Our results suggest practical implementation of 3D coil elements for integrated RF-shim coil arrays may be limited to 8ch and 16ch arrays. Although not evaluated in this study, 3D coil arrays may have benefits in SNR, depth penetration and g-factor over 2D arrays.Acknowledgements
We thank the authors of the opensource Matlab package for simulation (http://rflab.martinos.org/index.php?title=Multi-coil_B0_shimming).
References
1. Han H, Song AW, Truong TK. Integrated parallel reception, excitation, and shimming (iPRES). Magn Reson Med. 2013 Jul;70(1):241-7. doi: 10.1002/mrm.24766. Epub 2013 Apr 29. PMID: 23629974; PMCID: PMC3800110.
2. Truong TK, Darnell D, Song AW. Integrated RF/shim coil array for parallel reception and localized B0 shimming in the human brain. Neuroimage. 2014 Dec; 103:235-240. doi: 10.1016/j.neuroimage.2014.09.052. Epub 2014 Sep 28. PMID: 25270602; PMCID: PMC4312247.
3. Stockmann JP, Witzel T, Keil B, Polimeni JR, Mareyam A, LaPierre C, Setsompop K, Wald LL. A 32-channel combined RF and B0 shim array for 3T brain imaging. Magn Reson Med. 2016 Jan;75(1):441-51. doi: 10.1002/mrm.25587. Epub 2015 Feb 17. PMID: 25689977; PMCID: PMC4771493.
4. Wang ZJ. Improving SNR of RF coils using composite coil elements. NMR Biomed. 2009 Nov;22(9):952-9. doi: 10.1002/nbm.1410. PMID: 19582771.
5. Mueller M, Lanz T, Breuer F, Griswold M, Jakob P. Three-Dimensional Quadrature Array Coil Elements for Improved Parallel Magnetic Resonance Imaging Performance At 1.5 Tesla. Concepts in Magnetic Resonance Part a 2011;38A(2):61-73.
6. Maunder A, Fallone BG, Daneshmand M, De Zanche N. Experimental verification of SNR and parallel imaging improvements using composite arrays. NMR Biomed. 2015 Feb;28(2):141-53. doi: 10.1002/nbm.3230. Epub 2014 Nov 11. PMID: 25388793.
7. Zhou J, Stockmann JP, Arango N, Witzel T, Scheffler K, Wald LL, Lin FH. An orthogonal shim coil for 3T brain imaging. Magn Reson Med. 2020 Apr;83(4):1499-1511. doi: 10.1002/mrm.28010. Epub 2019 Oct 21. PMID: 31631391; PMCID: PMC7360482.
8. Avdievich NI, Giapitzakis IA, Pfrommer A, Borbath T, Henning A. Combination of surface and 'vertical' loop elements improves receive performance of a human head transceiver array at 9.4 T. NMR Biomed. 2018 Feb;31(2). doi: 10.1002/nbm.3878. Epub 2017 Dec 15. PMID: 29244225.
9. Lin F-H. http://maki.bme.ntu.edu.tw/Figures
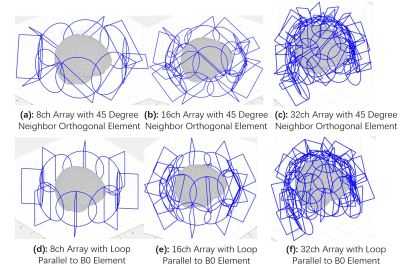
Fig.1 The 8ch, 16ch, 32ch Arrays with Different 3D Orthogonal Loop Coil Elements
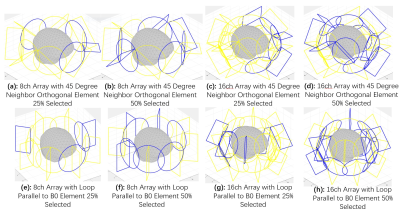
Fig.2 The 3D Orthogonal Coil Subarray Selection Results of 8ch, 16ch Cases (25% Selected, 50% Selected)
Fig.3 Shimming Results of 2D Loop and 3D Orthogonal Loop Geometries on a Certain Single Subject with 8ch, 16ch, 32ch, 39ch, 64ch, 128ch Coil Arrays (100% Coils Selected)
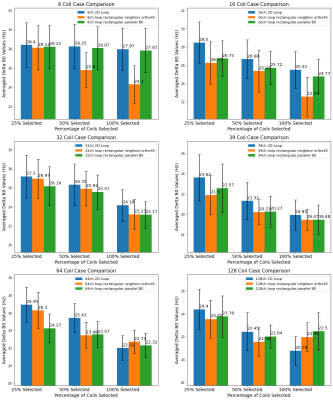
Fig.4 Shimming Results Using Coils Subarray Selection (25%, 50%, 100%) with Different Coil Arrays and Geometries