4508
Large benefit of RF coil B1 efficiency for X-nuclear MRS at ultrahigh field beyond the gyromagnetic ratio advantage of proton1Center for Magnetic Resonance Research (UMN), Minneapolis, MN, United States
Synopsis
In this study, we conducted electromagnetic simulation of a surface loop coil to quantify the B1+ transmit efficiency of five nuclei ( 1H, 31P, 23Na, 2H and 17O) from 1.5T to 21 T field strength. The simulation is validated by FID measurements. Furthermore, we investigated the loading effect on the RF coil B1+ transmit efficiency and its dependence on B0. Our study shows the X-nuclei flip angle can be reached using the same or even less coil driving voltage as for proton, thus, significantly reducing the required RF power and SAR concern.
Introduction
In vivo X-nuclear MRS-based metabolic imaging technologies are promising for studying cerebral metabolism, neurophysiology and bioenergetics in animal and human brains1-4. Their detection sensitivity and spectral resolution are significantly improved at high/ultrahigh field5-7. However, the X-nuclei have a lower Larmor frequency owing to their low gyromagnetic ratio (γ), especially for specific low-γ nuclei, such as 2H and 17O with approximately 6-7 times lower frequencies than 1H. They require a much large RF pulse voltage to achieve the same RF pulse flip angle (FA) as the proton according to the following relation:α = γ·pw·B1·V
where α is the RF excitation pulse flip angle; γ is the gyromagnetic ratio; pw is the RF pulse width; B1 is the magnetic transmission field of an RF coil; V is the RF pulse driving voltage. Hypothetically, as a worst-case scenario if one would assume the RF coil B1+ efficiency being independent from the resonant frequency, X-nuclei require γproton/γX-nuclei times of higher RF pulse voltage. This could be a potential SAR concern and cause serious implications on the RF-hardware. Nevertheless, the RF coil B1 efficiency is sensitive to the resonant frequency and increasing the resonant frequency leads to a larger reduction of B1+ efficiency for 1H than X-nuclei, thus, significantly reducing the high demand of X-nuclear RF pulse voltage and power.8 It is critical to investigate how exactly the RF coil B1+ efficiency scales with frequency, field-strength and how RF field penetration relates to coil configuration versus sample size and loading effect.8-11
In this study, we extend our previous study8, by simulating the B1+ transmit efficiency of five nuclei 1H, 31P, 23Na, 2H and 17O using a surface loop across a wide B0 range. We validated the simulation results with the FID measurement results of 1H and 17O B1 field8 at 7T. Furthermore, we investigated the loading effect on the RF coil B1 and its dependence on B0.
Materials and Methods
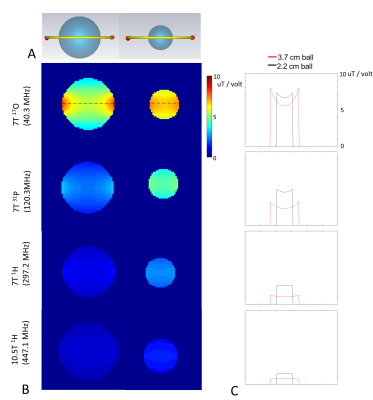
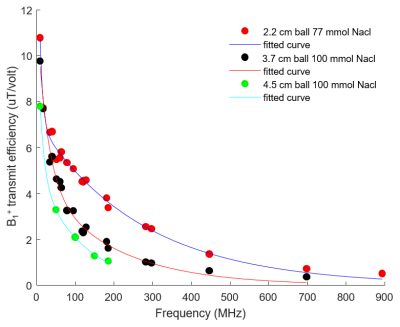
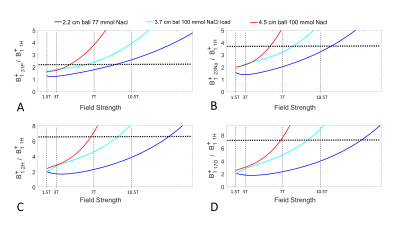
The electromagnetic simulation setup is shown in Fig. 1A. A 5.9 cm circular surface loop with one split capacitor is loaded with three NacCl solution phantoms (phantom diameter (cm)/NaCl concentration(mmol)): 2.2 / 77, 3.7 / 100, and 4.5 / 100. The loop coil is tuned and matched to ~ -20 dB at different resonant frequencies ranges from 8.7MHz to 894MHz. . We define the transmit efficiency of the x-nuclear (B1+x-nuclear) as the average |B1+| in the phantom divided by the driving voltage, and plotted it against the resonant frequency as shown in Fig. 2. A two terms exponential model was used as fitting function to fit the B1+x-nuclear and B0 for the three loading conditions. Using the fitted model, we predicted B1+x-nuclear / B1+proton for five nuclei at different B0 (Fig. 3).The electromagnetic simulation was conducted in CST studio suite 2020 (Dassault Systèmes, Vélizy-Villacoublay, France) and based on the hexahedral time-domain solver with 0.5 mm isotropic resolution mesh. In simulation, the NaCl solution ball and copper wire were constructed with realistic dielectric properties (copper wire conductance = 5.96×107 S/m; 77/100 mmol NaCl solution, Er = 77.5/80, conductivity = 0.87/1.15 S/m).
To validate the accuracy of the simulation, we imaged the 2.2 cm diameter 77 mmol NaCl solution ball at 7T at 17O and 1H frequencies with a B1 ratio of 2.838.
Results
Fig. 1B shows the |B1+| distribution within a small load (2.2 cm diameter 77 mmol NaCl) and heavy load (3.7 cm diameter 100 mmol NaCl). The |B1+| decreases as the resonant frequency increases. The 2D line plot through the middle of the NaCl ball shows that at low resonant frequencies, the |B1+| is stronger in the peripheral area while weaker in the center. On the other hand, for high frequency (10.5T 1H), |B1+| in the center of the phantom become stronger than the peripheral area associated with the RF wave behavior. Fig. 2 shows three fitting models to fit the B1+x-nuclear dependence on the resonant frequencies, and each fitting line corresponds to one loading condition. Fig. 3 demonstrates the benefits of B1+x-nuclear at ultrahigh field strengths and large loading condition. For the heavy loading condition (red lines in Fig. 3), the required RF pulse voltage for the same FA for 31P becomes smaller than that of 1H at ≥ 5T, and for 2H and 17O at ≥ 7T.Discussion
The B1+ x-nuclear / B1+ proton increases as the B0 increases. In addition, as loading increases, the B1+ x-nuclear increases even more dramatically. The flip angle of spin is proportional to the γ and B1+ x-nuclear. As B1+ x-nuclear / B1+ proton reaches γproton/γX-nuclei, the X-nuclei flip angle can be reached using the same or even less coil driving voltage as for proton, thus, significantly reducing the required RF power and SAR concern.Conclusion
The technical challenges of using X-nuclei compared to proton are dramatically reversed at high and ultrahigh fields, as in largely beneficial in higher RF coil B1+ efficiency towards lower γ nuclei at higher field strength and loaded condition. This is highly significant for advancing the X-nuclear MRS and imaging technology for human brain metabolic imaging applications at UHF.Acknowledgements
This work was supported in part by NIH grants of R01 CA240953, U01 EB026978, R01 MH111413, P41 EB027061.References
1. Zhu XH et al. In vivo 17O NMR approaches for brain study at high field. NMR in Biomedicine 18, 83-103, doi:10.1002/nbm.930 (2005).
2. Lu M et al. Quantitative assessment of brain glucose metabolic rates using in vivo deuterium magnetic resonance spectroscopy. J Cereb Blood Flow Metab 37:3518-3530 (2017).
3. De Feyter HM et al. Deuterium metabolic imaging (DMI) for MRI-based 3D mapping of metabolism in vivo. Sci Adv 4, eaat7314 (2018).
4. Zhu XH, Lu M & Chen W. Quantitative imaging of brain energy metabolisms and neuroenergetics using in vivo X-nuclear 2H, 17O and 31P MRS at ultra-high field. Journal of Magnetic Resonance 292, 155-170 (2018).
5. Lu M et al. In vitro and in vivo studies of 17O NMR sensitivity at 9.4 and 16.4 T. Magn Reson Med 69(6):1523-7 (2013).
6. Lu M, Chen W, Zhu XH. Field dependence study of in vivo brain 31P MRS up to 16.4 T. NMR Biomed 27(9):1135-41 (2014).
7. de Graaf RA et al. On the magnetic field dependence of deuterium metabolic imaging. NMR in Biomedicine 33:e4235 (2020).
8. Wiesner HM, Luo W, Yang QX, Zhu XH, Schillak S, Chen W. Quantitative Study of TX/RX-efficiency of X-Nuclear MRS/MRI at High/Ultrahigh Field. In: Proc Intl Soc Mag Reson Med; 2014; Milan, Italy. p. 810.
9. Hoult DI, Richards RE. The signal-to-noise ratio of the nuclear magnetic resonance experiment. J Magn Reson. 1976;24(1):71-85.
10. Hoult DI, Chen C-N, Sank VJ. The field dependence of NMR imaging. II. Arguments concerning an optimal field strength. Magn Reson Med. 1986;3(5):730-746.
11. Keltner JR, Carlson JW, Roos MS, Wong STS, Wong TL, Budinger TF. Electromagnetic fields of surface coil in vivo NMR at high frequencies. Magn Reson Med. 1991;22(2):467-480.
Figures