Introduction

Estimating the $$$B_1^+$$$-field is essential for many quantitative MRI methods and with the advent of ultra high-field MRI systems the demand of fast an accurate methods has become even more crucial.
In Bloch-Siegert shift based $$$B_1^+$$$-mapping an off-resonance RF pulses is applied which induces a phase shift proportional to the peek amplitude $$$B_{1p}$$$ of the RF pulse ¹. Because the shift is proportional to the RF power deposition, the method is SAR demanding, requiring long acquisition times. To increase the efficiency, the frequency of the RF-pulse can be decreased². Another possibility is a dense sampling of the k-space center in combination with variational image reconstruction³. Further, acquisition can be accelerated with 2D spiral and EPI readouts ⁴.
Here, we investigate the potential of faster image acquisition with a 3D segmented EPI sequence. To exploit errors associated with faster acquisition, the number of interleaves and the block-size of the k-space center were varied. This work shows that with an adequate choice of interleaves and block-size, whole-brain 3D $$$B_1^+$$$-maps can be acquired in 5 to 10 seconds.

Methods

Bloch-Siegert shift: Assuming that the off-resonance frequency $$$\omega_{RF}$$$ of the RF pulse with peak amplitude $$$B_{1p}$$$ is much larger than the field inhomgoneities $$$\Delta \omega_{B0}$$$ ( $$$\omega_{RF} >> \Delta \omega_{B0}$$$) and $$$|\gamma B_{1p}| << \omega_{RF}$$$, the Bloch-Siegert phase shift $$$\phi_{2BS} $$$ is given by¹:

\begin{equation} \phi_{2BS} =B_{1p}^2 \int_0^T \frac{(\gamma B_{1n}(t))^2}{\omega_{RF}} dt = B_{1p}^2 K_{BS}\end{equation}
wher $$$B_{1n}(t)$$$ is the normalized pulse envelope and the constant $$$K_{BS}$$$describes the pulse properties.

Sequence: We implemented a 3D segmented EPI with a variable number of interleaves $$$N_{Intlv}$$$ in phase encoding direction $$$k_y$$$ ⁵. For each shot, one partition $$$k_z$$$is encoded. In addition, a navigator echo prior and after the echo train and after was integrated to measure time-dependent $$$\Delta \omega_{B0}$$$ fluctuations. Before the imaging-part, one shot was acquired without phase encoding gradients to correct for Nyquist ghosts. Furthermore, 20 preparation pulses were executed to approach faster a steady-state. Because of the variable implementation of $$$N_{Intlv}$$$, the sequence can be scaled to a standard RF-spoiled gradient-echo sequence, and up to the acquisition of one partition in a single shot. In addition, a variable block-size in the k-space center can be selected to measure $$$n_y$$$ time $$$n_z$$$ out of $$$N_y$$$ times $$$N_z$$$ fully sampled k-space lines.

In-vivo measurements: Brain MRI of a healthy subject were carried out on 3T MR system (Magnetom Vida, Siemens Healthcare, Erlangen, Germany) with the following sequence parameters: binominal -1 1 water excitation pulse with $$$\alpha = 20^\circ$$$ and RF-spoiling increment of $$$90^\circ$$$ ⁶, $$$4ms$$$ Gaussian saturation pulse with nominal $$$\phi_{BS,nominal} = 46^\circ$$$, $$$TR = 45ms$$$, $$$BW=2000Hz/px$$$,$$$FOV=256x256x192mm^3$$$ and matrix size$$$N_x=64$$$, $$$N_y=64$$$ and $$$N_z=48)$$$.
First, fully sampled k-space ($$$n_y=64, n_z=48$$$) images were acquired with different $$$N_{Intlv}$$$. Second, the block-size was varied ($$$n_y= [32,16,12]$$$ and $$$n_z=[24,12,8,6]$$$), each with variable $$$N_{Intlv}$$$.

Image reconstruction: For all EPI data-sets ($$$n_y \neq N_{intlv}$$$), Nyquist-correction prior image reconstruction was applied. To combine the individual coil images and to estimate $$$\phi_{2BS}$$$, each coil image for the positive $$$\omega_{RF}$$$ was multiplied by the complex conjugate of the negative $$$\omega_{RF}$$$ coil image and summed up.

Results and Discussion

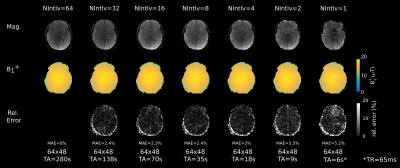
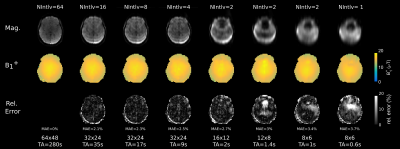
Figure 1 shows the reconstructed images for different $$$N_{intlv}$$$ (top), the estimated $$$B_1^+$$$ maps (middle), and the absolute error with respect to the gradient-echo images ($$$N_{intlv} = 64$$$). For the fully sampled k-space, visually a good correspondence between $$$B_1^+$$$-maps from $$$N_{intlv}=64$$$ with an acquisition time $$$TA=280s$$$ to $$$N_{intlv}=4$$$ ($$$TA=18s$$$) can be observed. Further reduction of $$$N_{intlv}$$$ leads to an increase of the mean absolute error (MAE). For $$$N_{intlv}=1$$$, distortions become more severe, which is also reflected in the $$$MAE$$$ by $$$5.2\%$$$.
The results for different block-patterns for varying $$$N_{intlv}=1$$$ are depicted in Figure 2. When comparing the quality of $$$B_1^+$$$-maps and the $$$MAE$$$ with the fully sampled results (Figure 1), it can be observe that the $$$MAE$$$ tends to be smaller for faster $$$TA$$$.

Conclusion

We have demonstrated that with segmented 3D EPI highly accelerated whole-brain $$$B_1^+$$$-maps can be acquired. To improve image quality, and potential further acceleration, variational reconstruction methods will be implemented and the navigator signals will be incorporated to compensate temporal $$$\Delta \omega_{B0}$$$ fluctuations⁷.

Acknowledgements

No acknowledgement found.