Introduction

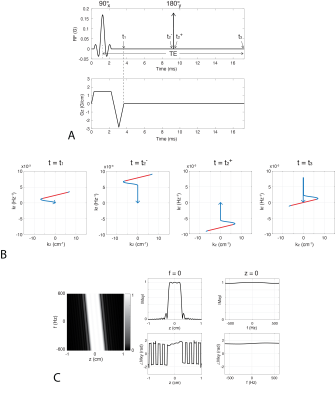
In the conventional excitation k-space formalism, only small tip angles are allowed for excitation. Here we present a new viewpoint for including 180° refocusing pulses in the excitation k-space to provide insights into the effects of refocusing pulses on off-resonance sensitivity. Spin echo pulse and refocused velocity-selective excitation pulse are explained by the proposed extended k-space formalism.

Theory

As shown in previous work [1,2], there is Fourier relationship between transverse magnetization M_xy and RF (with duration of T) deposited in multi-dimensional excitation k-space where the trajectory is determined by gradient waveforms and time:
$$M_{xy}=jγM_0 \int_{\bf K}p({\bf k}) e^{-j2π\bf{k}\cdot\bf x} d{\bf k},$$
where
$$p({\bf k})=\int_0^TB_1(t)δ({\bf k}(t)-{\bf k})dt,\\{\bf x}=[x\;\; y\;\; z\;\; f]^T,\\{\bf k}=[k_x\;\; k_y\;\; k_z\;\; k_f ]^T,\\{\bf k}(t)=[k_x(t)\;\; k_y(t)\;\; k_z(t)\;\; k_f(t)]^T,\\k_{x,y,z} (t)=\frac{γ}{2π}∫_t^TG_{x,y,z} (s)ds,\\k_f (t)=T-t.$$
Suppose that B₁(t) consists of multiple small-tip-angle subpulses within its duration of T, including one refocusing pulse at time T₀ (0 < T₀ < T). Immediately prior to the refocusing pulse (denoted by time T₀^-), M_xy can be written as:
$$M_{xy} (T_0^-)=jγM_0∫_{\bf K}p_0 ({\bf k})e^{-j2π{\bf k}\cdot{\bf x}} d{\bf k},$$
where
$$p_0({\bf k})=\int_0^{T_0^-}B_1(t)δ({\bf k}(t)-{\bf k})dt.$$
We assume that refocusing pulses are instantaneous for this k-space analysis. We describe the analysis for two different cases of refocusing pulse: rotation along x- and y-axis.

(i) Refocusing pulse along the x-axis:
The phase of M_xy at time T₀^- denoted by ϕ is changed to -ϕ at time T₀⁺, which is immediately after the refocusing pulse. Therefore, M_xy(T₀⁺) equals the complex conjugate of M_xy(T₀^-).
$$M_{xy} (T_0^+)=M_{xy}^* (T_0^- )=(-j)γM_0 ∫_{\bf K}p_0^* ({\bf k})e^{j2π{\bf k}\cdot{\bf x}} d{\bf k}.$$
Assuming that B₁(t) is real at all times (i.e. applied along the x-axis only), p₀^*(k) = p₀(k).
$$M_{xy} (T_0^+)=jγM_0 ∫_{\bf K}-p_0 ({\bf k})e^{-j2π(-{\bf k}\cdot{\bf x})} d{\bf k}.$$
This can be rewritten using k'=-k.
$$M_{xy} (T_0^+ )=jγM_0 ∫_{-{\bf K}}-p_0 (-{\bf k'})e^{-j2π{\bf k'}\cdot{\bf x}} (-d{\bf k'})=jγM_0 ∫_{\bf K}-p_0 (-{\bf k'})e^{-j2π{\bf k'}\cdot{\bf x}} d{\bf k'}.$$
Now k' can be changed back to k.
$$M_{xy} (T_0^+ )=jγM_0 ∫_{\bf K}-p_0 (-{\bf k})e^{-j2π{\bf k}\cdot {\bf x}} d{\bf k}.$$
This is still a form of Fourier transform of weighted k-space trajectory although the k-space weight is now determined by $$$p_0 (-{\bf k})=\int_{0}^{T_0^-}B_1(t)δ({\bf k}(t)+{\bf k})dt$$$, which corresponds to the origin symmetry of $$$p_0 ({\bf k})=\int_{0}^{T_0^-}B_1(t)δ({\bf k}(t)-{\bf k})dt$$$ in excitation k-space. Note that -1 is multiplied to $$$p_0 (-{\bf k})$$$ in the equation above, which indicates the sign change of the B₁(t) deposited for 0 < t < T₀. The rest of B₁(t) (T₀ < t < T) can continue to be deposited in k-space after the origin symmetric conversion of the B₁(t) deposited for 0 < t < T₀. However, after the refocusing pulse, magnetization is near the south pole (M_z < 0), and the effect of small tip α on M_xy is now -sin(α) instead of sin(α). Therefore, RF weight for T₀ < t < T should be considered to be -B₁(t) in the k-space analysis. Together with -1 multiplied to B₁(t) for 0 < t < T₀, this leads to multiplication of -1 to the entire B₁(t) (0 < t < T) in k-space analysis. M_xy at the end of excitation pulse sequence is
$$M_{xy} (T)=-jγM_0 ∫_{\bf K}p ({\bf k})e^{-j2π{\bf k}\cdot{\bf x}} d{\bf k},$$
where
$$p({\bf k})=∫_0^{T} \widehat {B}_1(t)δ({\bf k}(t)-{\bf k})dt,\\\widehat {B}_1(t)=B_1(t)\text { without refocusing pulse},\\{\bf k}(t)=[k_x(t)\;\; k_y(t)\;\; k_z(t)\;\; k_f(t)]^T,\\k_{x,y,z} (t)=\frac{γ}{2π}∫_t^T\widehat{G}_{x,y,z} (s)ds,\\\widehat{G}_{x,y,z} (t)=\begin{cases}-G_{x,y,z} (t) & t≤T_0\\G_{x,y,z} (t) & t>T_0\end{cases},\\k_f (t)=-|t-T_0 |+T-T_0.$$
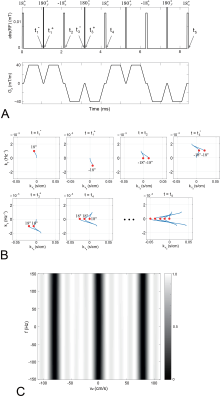
For RF pulses with multiple refocusing pulses, origin symmetric conversion should continue to be applied in k-space at each time point of refocusing pulse while non-refocusing RF pulses are deposited in k-space. Also, M_xy from RF pulse with n refocusing pulses will include a multiplier of (-1)ⁿ. This sign change, however, may be ignored because the sign of M_xy is not usually included in design parameters.

(ii) Refocusing pulse along the y-axis:
The phase of M_xy at time T₀^-, ϕ is changed to π-ϕ at time T₀⁺. Therefore,
$$M_{xy} (T_0^+ )=e^{jπ} M_{xy}^* (T_0^- )=-M_{xy}^* (T_0^-).$$
Note that there is an additional term of -1 here. This is different from the sign change described above. M_xy profile with refocusing along the y-axis will still include aforementioned sign change (i.e., (-1)ⁿ with n refocusing pulses), which again can be ignored. However, the additional term of -1 in the equation above imposes another sign change on the pre-deposited B₁(t). This sign change cannot be neglected because it reverses the sign of only parts of RF sequence (not entire RF sequence) and affects the shape of M_xy profile substantially. In general, if an RF pulse includes n refocusing pulses along the y-axis at time t₁, t₂, … t_n, the sign of B₁(t) deposited in k-space will be reversed for t_n > t ≥ t_n-1, t_n-2 > t ≥ t_n-3, t_n-4 > t ≥ t_n-5, and so on. Figure 1 and 2 illustrate examples of k-space analysis with refocusing pulses.

Discussion

We have proposed a new theoretical viewpoint for analyzing the effects of 180° refocusing pulses using excitation k-space, which was originally valid only in the small-tip-angle regime. This viewpoint may offer opportunities to design new complex RF pulse sequences with multiple refocusing pulses that require insensitivity to off-resonance in applications such as velocity-selective arterial spin labeling or velocity-selective angiography [3,4,6].

Acknowledgements

R01HD100012, R01HL135500