INTRODUCTION

Different reconstruction methods for magnetic resonance electrical properties tomography (MR-EPT) have now been published to estimate the conductivity and permittivity of tissues from MR measurements. However, there is a lack of comparative experiments between ground truth values, as measured by a vector network analyzer (VNA), and the reconstructed EP maps. In this work, we evaluated several phase-based (PB) and complex-image-based (CIB) methods, including the method by Soullié et al.[1], which was shown to overperform others in numerical simulations. Conductivity phantoms were made and scanned to evaluate the inter-session reproducibility of MR-EPT, and ground truth measurements were obtained using a VNA and a dedicated probe.

METHODS

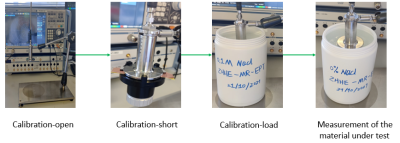
Phantoms: We made five simple gel tube phantoms (diameter = 10 cm, height=15 cm) consisting of 1 liter of deionized water, 15 g agar and different mass concentrations of NaCl, 0%, 1%, 2.5% and 5%, respectively.
Ground truth measurements: The reflection coefficient of dielectric materials (Γ) can be expressed by the complex relative permittivity (ε_r) and conductivity (σ), Γ= ε_r - jσ/( ε₀) where ω is the angular velocity and ε₀ is the vacuum permittivity. We used the dedicated probe from a commercial dielectric assessment kit (DAK 12, from SPEAG, Zurich, Switzerland), which was connected to a VNA (VNA ZNB 4, Rhodes & Schwartz), see Figure1. Reflection coefficients were measured by the VNA at high frequencies [50MHz, 150MHz], in open, short, and loaded circuit modes respectively, to calibrate the instrument. A reference saline solution (0.1 M NaCl) with known EP values was used for the loaded circuit mode. Finally, reflection coefficients from our phantoms were measured and permittivity and conductivity values were inferred [2], at the Larmor frequency of our scanner (Siemens Prisma 3T), i.e. 123MHz. Using a known EP value TSL-HU16 gel, we ensure that the measurement error is below 2.5%. Measurements, including the calibration procedure, were repeated over 4 sessions (4 different days, within 6 hours of the MR-EPT session) and temperatures were reported each time.
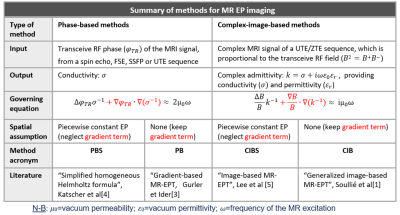
Phase-based and Complex-image-based MR-EPT: MR-EPT methods aim at reconstructing EP maps from images with strong RF weighting (B₁⁺ and B₁^- weighting), which are spatially modulated by local EP changes. We used two categories of MR-EPT methods (see Figure 2): phase-based (PB) methods [3] and complex-image-based methods (CIB) [1]. Phase-based methods use a phase image as an input, assumed to be the transceive RF phase (i.e. the phase of 𝐵₁⁺𝐵₁⁻) to reconstruct conductivity(σ). Complex-image-based methods use the complex MRI signal of a UTE/ZTE sequence with a low flip angle, which is proportional to the complex transceive RF field (𝐵² = 𝐵₁⁺𝐵₁⁻), to provide conductivity (σ) and permittivity (ε_r). Both PB and CIB methods involve a numerical solver to invert a regularized Helmholtz-type equation (regularization parameter was tuned manually for each method). Both methods have a simplified version, called phase-based simplified (PBS) [4] and complex-image-based simplified (CIBS) [5], in which EP values are considered piecewise constant, resulting in a simple and direct reconstruction formula. Therefore 4 MR-EPT maps were reconstructed (PBS, PB, CIBS, CIB).

RESULTS

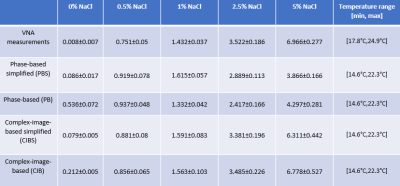
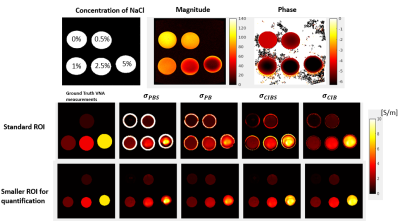
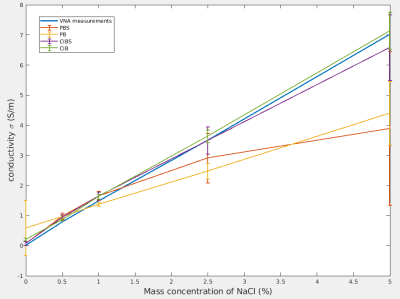
EP values obtained by MR-EPT and VNA are summarized in Figure 3. For all concentrations, except 0%, CIB provided the conductivity values closest to VNA measurements. However, at 0% NaCl, CIB exhibited overestimated values and CIBS was the closest. For all values, including VNA ones, the inter-session standard deviation was strongly dependent on the NaCl concentration and temperature. Examples of raw UTE images and reconstructed maps are shown in Figure 4. The raw maps are shown: the typical boundary artifacts can be seen for all reconstructions, especially for the simplified methods (PBS and CIBS). Masks used to calculate the values in Figure 3 are also displayed. The CIB method provided more uniform maps and the highest spatial resolution. Figure 5 shows the CIB values and the VNA values obtained at session 4 (22.2 °C), where the temperature was the most consistent between the MRI and VNA experiments.

DISCUSSION AND CONCLUSION

Standard deviations of conductivity values in the VNA measurements can be explained mainly by temperature differences between different sessions. All MR-EPT methods provided conductivity maps that were reproducible over different sessions and strongly correlated with the VNA ground truth. However, all MR-EPT methods seemed to slightly overestimate low conductivities (< 1 S/m), and phase-based methods seemed to slightly underestimate high conductivities (> 1 S/m). MR-EPT maps all showed a boundary artifact, which was more pronounced in simplified methods. The proposed setup can be used to compare other MR-EPT reconstruction methods. In future work, we will design more complex phantoms of different sizes and with multiple compartments to mimic in-vivo conditions.

Acknowledgements

Funding: French ANR project ELECTRA (ANR-21-CE19-0040).