2870

Head Position Related SAR Uncertainty Depends on Slice Orientation and Pulse Complexity

Emre Kopanoglu¹
¹CUBRIC / Psychology, Cardiff University, Cardiff, United Kingdom

Synopsis

Safety models on scanners are unaware of the actual patient position while excitation pulses are inherently position dependent. This study investigates the effect of this positional mismatch on SAR estimation for axial, coronal and sagittal slice orientations. The positional mismatch yields up to 5.2-fold underestimation of peak local SAR. RF shimming and 2-spoke parallel-transmit pulses for axial slice orientation have reduced SAR-sensitivity to positional mismatch with the worst-case underestimation being <2.0-fold whereas a reduced SAR-sensitivity was not observed for coronal and sagittal slices. For extreme head positions not represented in safety-models, axial RF shimming / 2-spokes parallel-transmit pulses maybe beneficial.

Introduction

Safety models ^1-3 on scanners are unaware of the actual patient position while excitation pulses are inherently position dependent (referred to as ‘positional mismatch’ throughout). Patient position is affected by variations in head shape/size, padding material alternatives, coil hardware specifications and circumstantial variations (patient settling in etc.). Recent studies showed that resulting ‘positional mismatches’ may have a considerable effect on SAR estimation ^4,5, focusing on axial slice-selective pulses. Here, a library of 26,082 realistic pulses were designed to investigate if certain slice orientations / pulse complexities are less susceptible to SAR underestimation due to ‘positional mismatch’.

Methods

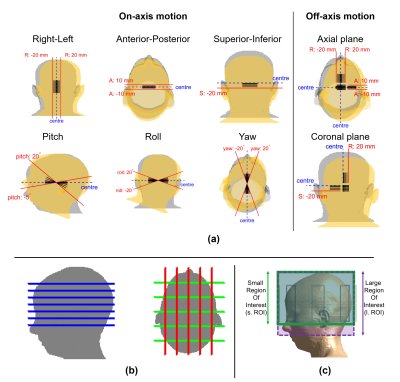
Body model Ella ⁶ was simulated at 161 different positions (Figure 1a) with respect to a generic 8-channel parallel-transmit (pTx) array at 7T, using Sim4Life (Zurich MedTech, Zurich, CH). Electromagnetic fields were exported to Matlab (Mathworks Inc., Natick, MA, USA) where small-tip angle (30-degrees) pulses were designed using Matching Pursuit-guided-Conjugate Gradient ^7-9 with Tikhonov regularization (β=0.5) over power in each channel. Full Q-matrices ^2,3 were used for SAR calculations, that were 10-gram averaged over cubical volumes ¹⁰. Omitted details followed Ref ¹¹.

Quadrature (single-channel) excitation, 1-spoke (RF shimming), 2-/3-/4-/5-spoke pTx pulses were designed for axial, coronal, sagittal slice-selective excitation at each position (Figure 1b). Pulses were designed for two different regions-of-interest (ROIs) for coronal and sagittal excitation (Figure 1c). A total of 26,082 pulses were designed. Each pulse was evaluated at its actual position ($$$SAR_{actual}$$$) and using the centred body position as the ‘safety-model’ ($$$SAR_{safety-model}$$$). The ratio $$$SAR_{actual}/SAR_{safety-model}$$$ is reported for peak spatial (local) SAR (psSAR) and whole-head SAR (whSAR) to characterize how much ‘positional mismatch’ affects SAR estimations. Maximum intensity projections (MIP) of the three-dimensional local SAR distributions are given. Effect of the ROI on pulses were tested at p=0.05 using two-sample t-tests against the null hypothesis that ROI does not affect mean SAR sensitivity to ‘positional mismatch’.

Results

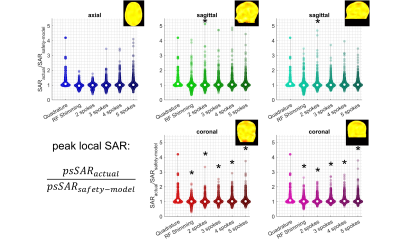
Actual peak local SAR was up to 5.2-fold higher than that estimated by the safety model (Figure 2). For axial, sagittal and coronal pTx-pulses, the worst-case underestimation was 4.2-fold, 5.2-fold and 3.8-fold, respectively. Quadrature excitation was invariant on slice orientation/location as channel coefficients are fixed relative to each other (global flip-angle scaling cancels in $$$SAR_{actual}/SAR_{safety-model}$$$), the worst-case underestimation being 4.2-fold.

RF shimming and 2-spokes axial pulses have less SAR-sensitivity to ‘positional mismatch’ than quadrature and pTx pulses with more spokes (Figure 2). For coronal and sagittal pulses, there was no considerable difference between pulses types.

Targeting a smaller ROI yielded reductions in SAR sensitivity for coronal and sagittal slices (Figure 2). The reductions were statistically significant in 6/10 comparisons.

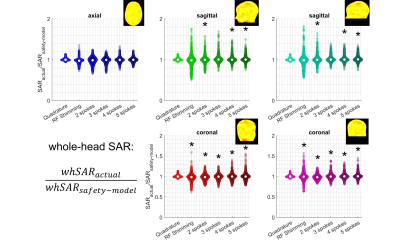
‘Positional mismatch’ yielded up to 1.4-fold, 1.8-fold and 1.6-fold underestimation of whole-head SAR for axial, sagittal and coronal pulses, respectively (Figure 3). The size of the ROI affected SAR sensitivity, as 8/10 comparisons across the sagittal and coronal pulses were statistically significant.

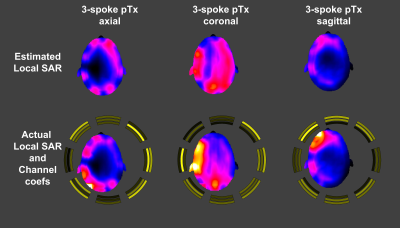
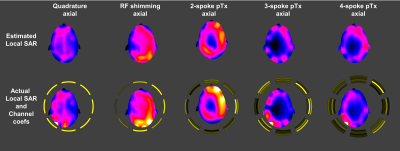
‘Positional mismatch’ may also lead to misinterpretation of the location of the hotspots (Figures 4-5). When channel coefficients are the largest for the coils closest to the head, the hotspots positions are correctly estimated by the safety-model (e.g. Figure 4 sagittal) although the peak value is underestimated. When distal coils are more heavily used, however, the actual hotspot positions can vary drastically compared to the estimate (e.g. Figure 4 axial/coronal).

Worst-case SAR underestimation depended considerably on the pulse type and did not always occur at the same head position (Figures 4-5). Figure 5 shows that for the worst-case RF shimming and 2-spoke pulses while the spatial distribution of local SAR was correctly estimated, the peak was underestimated, whereas both the distribution and the peak were incorrectly estimated for quadrature, 4-/5-spokes pulses.

Discussion

The results here are in agreement with Refs. ^4,5 for overlapping cases: axial slice-selection via RF shimming ^4,5 and multi-spokes pTx pulses ⁴.

Local SAR depends directly on channel coefficients of and proximity to coils (as well as interactions between coils). The ‘positional mismatch’ may cause misrepresentation of the head-coil proximity, leading to SAR underestimation. Furthermore, it may cause the location of local SAR hotspots to be estimated incorrectly, when distal coils have higher coefficients. This latter issue may cause concerning hotspot overlaps if SAR hopping ¹² is used.

This study shows that slice orientation has an impact on SAR-sensitivity to ‘positional mismatch’. Importantly, axial RF shimming and 2-spokes pTx pulses suffer less SAR-underestimation than other axial/sagittal/coronal pulses. This is due to their self-correcting nature: coils closer to the head will likely have smaller coefficients (compared to a centred position) to ensure that the flip-angle does not exceed the target in their vicinity. This isn’t true for more spokes as individual spokes target inhomogeneous distributions that complement each other, potentially leading to large channel coefficients in coils closer to the head. Coronal and sagittal pulses are not self-correcting either: with the coils being distributed azimuthally and outside the planes of interest, the effect of changing coil-head proximity is less direct and requires less self-correcting adjustments.

Conclusion

Axial RF shimming and 2-spokes pTx pulses suffer less SAR-underestimation than other axial/sagittal/coronal pulses. Hence, such pulses may be preferable for extreme/non-represented head positions.

Acknowledgements

This project was supported by the Wellcome Trust [204824/Z/16/Z].

References

1. Eichfelder G, Gebhardt M. Local specific absorption rate control for parallel transmission by virtual observation points. Magn Reson Med 2011;66(5):1468-1476.

2. Graesslin I, Homann H, Biederer S, Börnert P, Nehrke K, Vernickel P, Mens G, Harvey P, Katscher U. A specific absorption rate prediction concept for parallel transmission MR. Magn Reson Med 2012;68(5):1664-1674.

3. Bardati F, Borrani A, Gerardino A, Lovisolo GA. SAR optimization in a phased array radiofrequency hyperthermia system. Specific absorption rate. IEEE Trans Biomed Eng 1995;42(12):1201-1207.

4. Kopanoglu E. Patient specific parallel transmit pulses are patient position dependent while safety models are fixed: safety implications. 2021; online. p 2299.

5. Ajanovic A, Hajnal J, Tomi-Tricot R, Malik S. Motion and Pose Variability of SAR Estimation with Parallel Transmission at 7T. 2021; online. p 2487.

6. Andreas C, Wolfgang K, Eckhart GH, Katharina H, Marcel Z, Esra N, Wolfgang R, Rolf J, Werner B, Ji C, Berthold K, Peter S, Hans-Peter H, Jianxiang S, Michael O, Dominik S, Anthony K, Joshua WG, Niels K. The Virtual Family—development of surface-based anatomical models of two adults and two children for dosimetric simulations. Physics in Medicine & Biology 2010;55(2):N23.

7. Grissom W, Yip CY, Zhang Z, Stenger VA, Fessler JA, Noll DC. Spatial domain method for the design of RF pulses in multicoil parallel excitation. Magn Reson Med 2006;56(3):620-629.

8. Kopanoglu E. Near real-time parallel-transmit pulse design. Proc. ISMRM and ESMRMB; 2018; Paris, France. p 3392. (Proc. ISMRM and ESMRMB).

9. Kopanoglu E, Constable RT. Radiofrequency pulse design using nonlinear gradient magnetic fields. Magn Reson Med 2015;74(3):826-839.

10. IEC/IEEE International Standard -- Determining the peak spatial-average specific absorption rate (SAR) in the human body from wireless communications devices, 30 MHz to 6 GHz - Part 1: General requirements for using the finite-difference time-domain (FDTD) method for SAR calculations. IEC/IEEE 62704-1:2017 2017:1-86.

11. Kopanoglu E, Deniz CM, Erturk MA, Wise RG. Specific absorption rate implications of within-scan patient head motion for ultra-high field MRI. Magn Reson Med 2020;84(5):2724-2738.

12. Guerin B, Adalsteinsson E, Wald LL. Local SAR reduction in multi-slice pTx via “SAR hopping” between excitations. 2012.

Figures

Figure 1: Study details. (a) The body model was simulated at 161 relative positions with respect to the coil array, simulating all six degrees of freedom. (b-c) Pulses were designed for 7 axial, 5 coronal and 5 sagittal slices (b), and for two different ROI definitions for sagittal and coronal pulses (c). The volume covered with the smaller ROI definition is comparable to the volume covered by the axial slices.

Figure 2: The ratio of the actual peak local SAR to the peak local SAR calculated using the safety model is shown for different pulse types, slice orientations and ROIs. Example 5-spoke excitation profiles show ROI definitions. Axial RF shimming and 2-spokes pulses reduce the sensitivity of SAR to positional mismatch, while coronal and sagittal slices show considerable sensitivity to ‘positional mismatches’ for all pulse types. Asterisk denotes statistically significant difference between otherwise-identical sagittal/coronal pulses designed for different ROIs.

Figure 3: The ratio of the actual whole-head SAR to the whole-head SAR calculated using the safety model is shown for different pulse types, slice orientations and ROIs. Example 5-spoke excitation profiles show ROI definitions. The estimation error is less than 40% for axial slices, but as high as 80% for sagittal and 60% for coronal slices. Asterisk denotes statistically significant difference between otherwise-identical sagittal/coronal pulses designed for different ROIs.

Figure 4: ‘Positional mismatch’ (actual vs safety model) leads to underestimation of local SAR and misrepresentation of the spatial distribution. Local SAR estimated using the centred safety model and the actual local SAR are shown as MIPs onto the axial plane, for worst case axial, sagittal and coronal 3-spoke pTx pulses. Estimated and actual SAR plots have the same colour range. Yellow-shaded arcs: channel coefficients; brighter: higher amplitude; inner to outer: first to third spoke.

Figure 5: ‘Positional mismatch’ (actual vs safety model) leads to underestimation of local SAR and misrepresentation of the spatial distribution. Local SAR estimated using the centred safety model and the actual local SAR are shown as MIPs onto the axial plane, for worst case quadrature, RF shimming, 2- / 3- / 4-spoke axial excitation pTx pulses. Estimated and actual SAR plots have the same colour range. Yellow-shaded arcs: channel coefficients; brighter: higher amplitude; inner to outer: first to last spoke.

Proc. Intl. Soc. Mag. Reson. Med. 30 (2022)

2870

DOI: https://doi.org/10.58530/2022/2870