2553
A 16-channel transceiver dipole array for 1H body imaging at 10.5T: Validation and VOP enabled imaging in-vivo1Center for Magnetic Resonance Research, University of Minnesota, Minneapolis, MN, United States
Synopsis
To realize the increased pTx functionality of a 16-channel transceiver dipole array for body imaging at 10.5T, a validation process was implemented. After quantifying the uncertainty of the RF arrays electromagnetic simulations a total safety factor was determined enabling the implementation of real-time local SAR monitoring on the scanner through use of virtual observation points (VOP). In vivo data was acquired with the VOP enabled power monitoring demonstrating local RF shimming performance with and without local SAR constrained optimizations.
Introduction
Dipole antennas are a well-established design used for body imaging at ultra-high fields. Recently the fractionated dipole antenna1, originally designed for 7T, was adapted to 10.5T2 and successfully used in a 10-channel configuration to acquire the first in-vivo images in the body at 447MHz3. Increased element density at 10.5T compared to 7T is supported by the shorter wavelengths. To fully exploit the improved transmit efficiency and SNR of this 16-channel dipole array, real-time SAR prediction is required. In this work, we detail the needed validation process and subsequent safety factor determination used for scaling of Q-matrices prior to virtual observation point (VOP)4 compression. In vivo data was acquired with the VOP enabled power monitoring demonstrating the potential of SAR constrained parallel transmit management.Methods
Coil validationThe coil consists of 16 fractionated dipoles tuned to the resonance frequency of 1H at 10.5T (447MHz). Each element is 20cm in length as described in Erturk et al.2 with 6cm center-to-center spacing. For validation purposes, the elements were positioned around a body-sized polyvinylpyrrolidone (PVP)-agar phantom model (c.f. Tab.1). A small container was in the phantom to obtain a phase reference for MR thermometry5 (MRT). All MR experiments were conducted on a whole-bode 10.5T magnet.
Relative B1+ mapping was performed on a channel-wise basis6. In addition, absolute B1+ mapping using the AFI7 method was performed for two B1+-shims with elements driven with equal amplitude: CP+-mode and 0-180-mode (22.5° and 180° phase increment, respectively). Relative and absolute B1+ maps were combined to obtain absolute channel-wise B1+ maps. Subsequently, channel-wise real-valued scaling factors were determined for the simulated B1+ data to match experimental conditions.
MRT data was acquired using a multi-echo GRE (TE=5/10/15ms) before and after a ten-minute heating sequence (2.2ms rectangular RF pulse, Pavg=88.1W) for both shims. All MRT data was corrected for a DC offset based on the small oil container. SAR was calculated as . Simulation data was scaled based on the channel-wise scaling factors and averaged over the same voxel volume (4x4x4mm³) for comparison of SAR maps.
The inaccuracy of the EM model of the array was assessed as described in Steensma et al.7, by calculating the relative difference between the measurement and simulation for all voxels and both shims. Subsequently, the modeling error was defined as the positive error that is not exceeded in 99.9% of all voxels. Since both B1+ maps and SAR maps were available in this study, the largest error was used as the model inaccuracy.
EM simulations on the pelvis of three human body models (Duke, Ella, and Fats29; Sim4Life, Zurich Medtech, Zürich, Switzerland) were performed. To assess the intersubject variability, peak 10g SAR in all three models was evaluated for 500,000 random phase-magnitude shims. Subsequently, the Duke model was chosen as a reference and the relative peak 10g SAR deviation between the other two models and Duke was calculated for each shim:
Δpeak10gSAR,i=(peak10gSAR)i−(peak10gSAR)Duke(peak 10g SAR)Duke for i = Ella, Fats29
The intersubject variability was defined as the positive relative peak 10g SAR deviation that is not exceeded in 99.9% of all shims. The largest value of the two model comparisons was used as the final intersubject variability.
The model inaccuracy, intersubject variability, and the vendor-provided power monitoring inaccuracy (15%) were combined in a sum-of-squares way to derive a total inaccuracy, which was then used to determine a total safety factor. Safety factor scaled Q-matrices from the Duke model were compressed into VOPs with a 10% SAR overestimation factor.
In-vivo study
First in vivo imaging with the 16 channel array with VOP power monitoring was performed. To explore the functionality afforded by the use of VOPs as opposed to total or channel-wise power limits, T2W TSE acquisitions were performed in the pelvis of a healthy male subject following an IRB approved protocol with an FDA Investigational Device Exemption. Targeting the prostate, localized phase-only efficiency shimming9,10 was performed without and with local SAR constraint based on the VOPs.
Results
The channel-wise scaling factors derived from the simulated and measured channel-wise B1+ maps are 0.53±0.03 (Fig.1). Simulated and experimental B1+ maps as well as SAR distributions are shown in Figure 2A+B, together with the respective relative errors (Fig.2C), yielding a model inaccuracy of 33.4%. Histograms of peak 10g SAR for the random phase-magnitude shims are displayed in Figure 3A-C. The largest peak 10g SAR deviation relative to the Duke model of 39.4% was found in Ella (Fig.3E). Based on these results, the total safety factor was set to:SFtotal=1+√0.152+0.332+0.392=1.53
Compressing the 204,833 Q-matrices of the Duke simulation resulted in 155 VOPs.
In-vivo images of the efficiency shim targeting the prostate are displayed in Figure 4. Both solutions, unconstrained (Fig.4a) and local SAR constrained (Fig.4b), lead to similar results in terms of the overall B1+ field pattern. However, a reduction of peak 10g SAR of 18% was achieved when optimizing the shim under local SAR constraint.
Conclusion
In this work, we demonstrate the process needed to enable real-time SAR monitoring needed to exploit the full potential of a 16-channel transceiver array at 10.5T and demonstrate its use for SAR constrained RF shimming in vivoAcknowledgements
No acknowledgement found.References
[1] Raaijmakers, A.J., Italiaander, M., Voogt, I.J., Luijten, P.R., Hoogduin, J.M., Klomp, D.W. and van den Berg, C.A. (2016), The fractionated dipole antenna: A new antenna for body imaging at 7 Tesla. Magn. Reson. Med., 75: 1366-1374. https://doi.org/10.1002/mrm.25596
[2] Ertürk, M.A., Wu, X., Eryaman, Y., Van de Moortele, P.-F., Auerbach, E.J., Lagore, R.L., DelaBarre, L., Vaughan, J.T., Uğurbil, K., Adriany, G. and Metzger, G.J. (2017), Toward imaging the body at 10.5 tesla. Magn. Reson. Med., 77: 434-443. https://doi.org/10.1002/mrm.26487
[3] He, X, Ertürk, MA, Grant, A, et al. First in-vivo human imaging at 10.5T: Imaging the body at 447 MHz. Magn Reson Med. 2020; 84: 289– 303. https://doi.org/10.1002/mrm.28131
[4] Eichfelder, G. and Gebhardt, M. (2011), Local specific absorption rate control for parallel transmission by virtual observation points. Magn. Reson. Med., 66: 1468-1476. https://doi.org/10.1002/mrm.22927
[5] J. C. Hindman (1966), Proton Resonance Shift of Water in the Gas and Liquid States, J. Chem. Phys. 44, 4582-4592. https://doi.org/10.1063/1.1726676
[6] Van de Moortele, P-F and Ugurbil, K. (2009), Very Fast Multi Channel B1 Calibration at High Field in the Small Flip Angle Regime. In Proceedings of the 17th Annual Meeting of the ISMRM.
[7] Yarnykh, V.L. (2007), Actual flip-angle imaging in the pulsed steady state: A method for rapid three-dimensional mapping of the transmitted radiofrequency field. Magn. Reson. Med., 57: 192-200. https://doi.org/10.1002/mrm.21120
[8] Steensma, B., Luijten, P., Klomp, D., van den Berg, N. and Raaijmakers, A. (2020), Tier based formalism for RF safety assessment of custom-built RF coils providing flexibility in the tradeoff between effort and overestimation. In Proceedings of the 28th Annual Meeting of the ISMRM
[9] Zhu, Y., Deniz, C., Alon, L., Fautz, H. and Sodickson D.K. (2010), Understanding parallel transmit array efficiency. In Proceedings of the 18th Annual Meeting of the ISMRM
[10] Deniz, C.M., Brown, R., Lattanzi, R., Alon, L., Sodickson, D.K. and Zhu, Y. (2013), Maximum efficiency radiofrequency shimming: Theory and initial application for hip imaging at 7 tesla. Magn Reson Med, 69: 1379-1388. https://doi.org/10.1002/mrm.24377
Figures
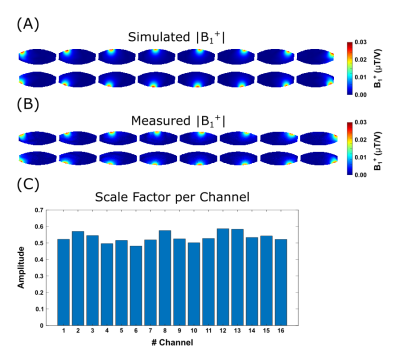
Figure 1:
Channel-wise absolute B1+ maps of the simulation (A) and experimental data (B). The simulation data was scaled by the channel-wise scale factors (C).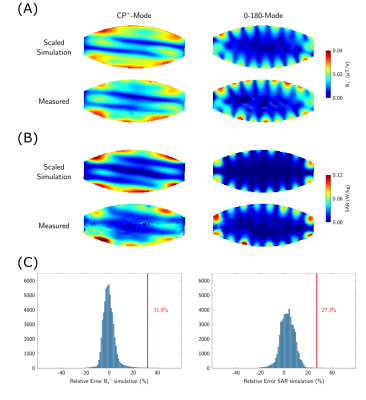
Figure 2:
B1+ (A) and SAR (B) comparison between scaled simulation results and experimental data for both shims. The model inaccuracy is based on the relative error (C).
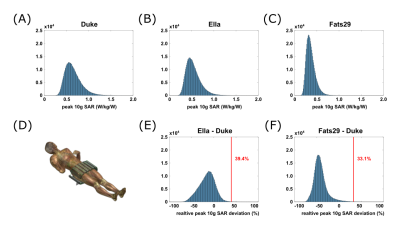
Figure 3:
Peak 10g SAR values for 500,000 random phase-magnitude shims normalized to 1W input power for all three human body models (A-C) with the array positioned around the pelvis (D). The intersubject variability is based on the relative peak 10g SAR deviation of Ella (E) and Fats29 (F) with respect to Duke.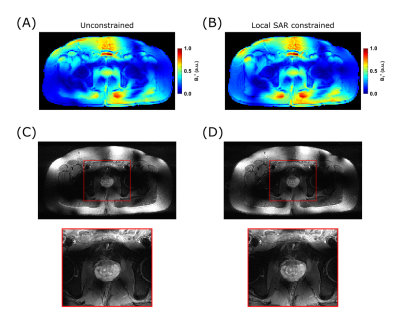
Figure 4:
Comparison between unconstrained (A+D) and local SAR constrained (B+C) efficiency phase-only shimming targeting the prostate. Both shim solutions produce similar B1+ patterns, while the constrained shim leads to 18% SAR reduction.
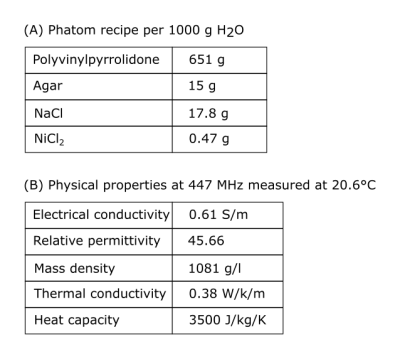
Table 1:
Phantom properties