1862
Practical RF-pulse shape designs to minimize off-resonance artifacts in dissolved-phase hyperpolarized 129Xe MRI1Medical Physics, Duke University, Durham, NC, United States, 2Biomedical Engineering, Duke University, Durham, NC, United States, 3Radiology and Medical Imaging, University of Virginia, Charlottesville, VA, United States
Synopsis
Minimizing artifacts in 129Xe gas exchange MRI requires selectively exciting the broad dissolved phase resonances, without exciting the much larger gas phase pool. This requires not only a short, selective pulse, but also overcoming pulse distortions imposed by the RF amplifier. Here, we demonstrate a novel, practical RF pulse based on augmenting the traditional Hanning window pulse with tunable DC bias and sub-lobes in the time-domain. We characterized the performance through simulations, phantom, and in vivo testing and determined that the tunable DC bias parameter creates a robust dissolved-phase excitation while minimizing gas-phase contamination.
Introduction
Hyperpolarized 129Xe MRI can evaluate pulmonary function via 3D mapping of ventilation, interstitial barrier uptake, and RBC transfer1,2,3. Such 3-compartment imaging provides sensitivity to many cardiopulmonary conditions. However, a major challenge in implementation remains minimizing gas-phase contamination when exciting the dissolved-phase compartment. Because the dissolved-phase 129Xe signal is only 1-2% of that from the gas-phase, images of this compartment are readily corrupted by unintended excitation of the latter. Although the chemical shift between compartments is relatively large (~200ppm), selective excitation remains challenging because the pulses must be short compared to T2* (~1ms at 3T). Leung et al.4 demonstrated elegant, short pulses that analytically suppress gas-phase signal. Nevertheless, such pulses are susceptible to very-minor distortions caused by RF amplifiers that can drastically reduce the real-world frequency selectivity. Alternatively, such contamination can potentially be removed through elegant post processing methods5,6 . Here, we propose to avoid such contamination using a generalized pulse shape based on a Hanning window with additional tunable waveform elements. Using both phantom and in-vivo testing, we demonstrate that this Hanning with Side Lobe Suppression (Hanning SLS) pulse provides robust frequency selectivity in a practical setting.Methods
Pulse Design and SimulationThe standard Hanning window of N samples is given as:
whann[n]=sin2(πn/N),0≤n≤N
Inspired by the original formulation7, we add a constant (DC) bias wDC and sub-pulses wSL that yield additional phase-tunable sidelobes:
wSL[n]=whann[n]+wDC+wSL[n],0≤n≤N
where
wSL[n]={wSL∗exp(iϕSL)if0≤n≤NSLwSL∗exp(−iϕSL)ifN−NSL≤n≤N0,otherwise
A representative pulse profile is displayed in Figure 1. In addition to the RF pulse length, this provides four additional degrees of freedom to tailor the frequency response: wDC, wSL, ϕSL, and NSL.
The frequency response of this pulse was simulated in Matlab as a series of rotations about the time-varying B-field vector (assuming only one dissolved resonance). The time domain RF pulse envelopes evaluated were initialized according to the above equations (N=690, total duration 690μs , frequency offset = 7090Hz corresponding to excitation at 208ppm at 3T, α=20o). Optimal parameters were determined by searching the parameter-space for the highest ratio of dissolved phase (f = 7090Hz) to gas phase (f = 0 Hz) spectral magnitude.
Experimental Verification in a 129Xe sample and in vivo
RF pulses described above were then implemented on a 3T scanner (Siemens Magnetom Trio – VB19A), first using a proton phantom to determine the optimal empirical parameters. Results were subsequently verified on a 1L bag of hyperpolarized 129Xe and in 1 healthy volunteer using a flexible quadrature vest coil (Clinical MR Solutions, USA). Because the gas-phase resonance has a non-zero linewidth (typically 40-50Hz), the pulses were tested across a range of off-resonance frequencies (bag: 6.5Khz-7.7kHz, human: 7.0kHz-7.25kHz).
Results
Figure 2a shows the log magnitude frequency responses of a standard Hanning window pulse of duration 690µs and one with DC bias added (parameters: wDC=0.021, wSL=0, ϕSL=0, and NSL=0). These parameters were determined by sweeping through the parameter space and identifying suppressed frequency response at the gas-phase frequency, with a finite linewidth. Shown in Figure 3 is the frequency response upon independently sweeping through each variable.For our scanner, the same amplifier is used for both local proton transmit/receive coils and multinuclear coils. We thus used a proton phantom in a Tx/Rx head coil to sweep through all the possible parameters which indicated pulse length = 710µs, no sub-pulses, and DC bias = 3% provided the lowest contamination ratio for the gas frequency offset. Figure 4a shows the gas phase contamination plotted as a function of offset frequency for a hyperpolarized 129Xe bag. Although, at frequencies near 208ppm, the gas phase contamination of the Hanning SLS was only mildly superior to the simple Hanning window, its gas phase contamination remained under 0.1% throughout the entire frequency range. This pattern is replicated in the human subject (Figure 4b) as the gas phase contamination had a maximum of 3.6% for the Hanning SLS pulse versus 6.8% for the Hanning pulse.
Discussion
The Hanning SLS RF pulse can achieve both theoretically and empirically superior performance compared to the simple Hanning window. Theoretically, the DC bias parameter can reduce gas-phase excitation as shown in Figure 3. Similarly, the three parameters defining the sub-pulses largely affect the location of the local minima in frequency response. For our specific system, a DC bias of ~3% provided optimal performance. In our hands, the sub-pulses improved performance compared to simple Hanning, but not as much as DC bias. However, as RF amplifier distortions can vary across systems, this added degree of freedom may provide additional tuning. Our investigation in varying the nominal offset frequency demonstrates that the Hanning SLS pulse with DC bias provides not only lower off-resonance contamination but maintains performance over a broader frequency range. Moreover, with the implementation of additional tunable parameters, the Hanning SLS pulse provides practical flexibility across various sites and hardware manufacturers.Acknowledgements
R01HL105643, R01HL12677, NSF GRFP DGE-1644868References
1. Mugler, J. P. & Altes, T. A. Hyperpolarized 129Xe MRI of the human lung. J. Magn. Reson. Imaging 37, 313–331 (2013).
2. Wang, Z. et al. Using Hyperpolarized 129 Xe Gas Exchange MRI to Model the Regional Airspace, Membrane and Capillary Contributions to Diffusing Capacity . J. Appl. Physiol. (2021) doi:10.1152/japplphysiol.00702.2020.
3. Niedbalski, P. J. et al. Protocols for multi- site trials using hyperpolarized Xe MRI for imaging of ventilation , alveolar- airspace size , and gas exchange : A position paper from the 129 Xe MRI clinical trials consortium. 1–21 (2021) doi:10.1002/mrm.28985.
4. Leung, G., Norquay, G., Schulte, R. F. & Wild, J. M. Radiofrequency pulse design for the selective excitation of dissolved 129Xe. Magn. Reson. Med. 73, 21–30 (2015).
5. Hahn, A. D., Kammerman, J. & Fain, S. B. Removal of hyperpolarized 129Xe gas-phase contamination in spectroscopic imaging of the lungs. Magn. Reson. Med. 80, 2586–2597 (2018).
6. Willmering, M. M., Cleveland, Z. I., Walkup, L. L. & Woods, J. C. Removal of off-resonance xenon gas artifacts in pulmonary gas-transfer MRI. Magn. Reson. Med. 86, 907–915 (2021).
7. Nuttall, A. H. Some Windows with Very Good Sidelobe Behavior. IEEE Trans. Acoust. 29, 84–91 (1981).
Figures
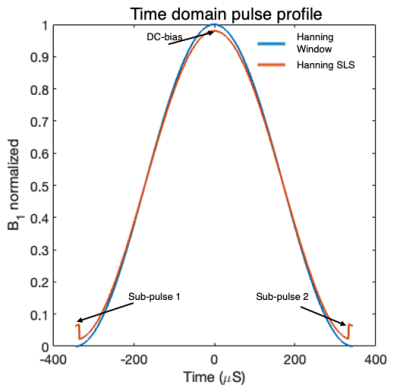
Figure 1. Time domain magnitude pulse profile of a Hanning window pulse (blue) and the proposed Hanning with side lobe suppression (Hanning SLS) pulse. Both pulses are normalized to achieve a 20o flip angle. The Hanning SLS is illustrated with 10% DC bias, and a 10us, 0.1o sub pulse (phase not shown), which is exaggerated for visual display purposes.
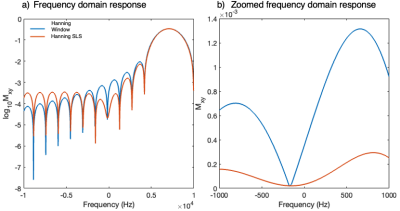
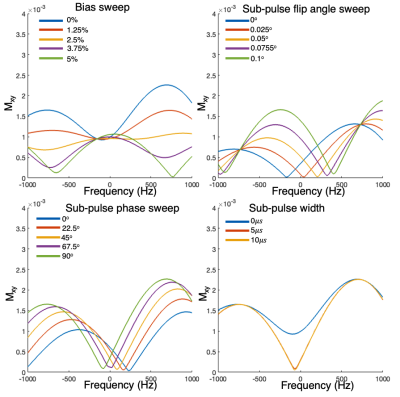
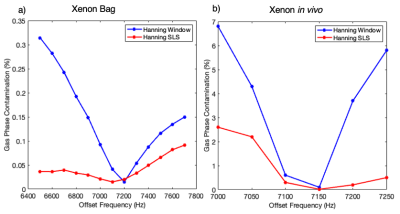
Figure 4. Gas phase contamination percentage as a function of prescribed offset frequency acquired in a) xenon bag and b) in vivo from a healthy subject. Again, for Hanning SLS, the off-resonance contamination is suppressed over a broader frequency range.