1844
Quantifying Cardiac Hypertrophy using Biophysical Models of Diffusion MRI1Biomedical Imaging Science Department, University of Leeds, Leeds, United Kingdom, 2Cardiovascular Medicine, University of Oxford, Oxford, United Kingdom, 3Diamond Light Source Ltd., Oxfordshire, United Kingdom
Synopsis
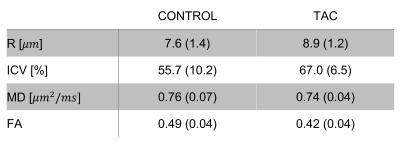
Biophysical modelling is a valuable technique for interrogating cardiac microstructure. We utilise a two-compartment model where intra- and extra-cellular space were represented by impermeable cylinders and an oblate diffusion tensor, respectively. Data was acquired ex vivo using a bespoke diffusion scheme with five diffusion times, six b-values and ten directions. Cardiomyocyte radius (r = 7.6 versus 8.9 μm) and volume fraction (ICV = 0.56 versus 0.67) were sensitive markers of cardiomyopathy validated in one healthy and one disease heart with transverse aortic constriction (TAC).
Introduction
Biophysical modelling of diffusion-weighted magnetic resonance imaging (DW-MRI) data is a promising means of characterising cardiac microstructure1-3. We have recently examined a range of two-compartment models in the heart where cardiomyocytes were represented with four different cylinder models including the standard cylinder, cylinders with an elliptical cross-section (ECS), cylinders with Gamma distributed radii (GDR), and cylinders with Bingham distributed axes (BDA)3. The extracellular space was represented by either an isotropic tensor (ball) or an oblate tensor (pancake). However, the sensitivity of estimated biophysical parameters to cardiac pathologies has not been examined yet.This study aims to assess the sensitivity of estimated biophysical parameters including cardiomyocyte radius (r) and volume fraction (ICV) using one healthy and one disease heart with transverse aortic constriction (TAC).
Methods
Sample preparation and data acquisition: Mouse hearts were excised and scanned as previously described4. In brief, images were acquired on a 9.4T MRI system using a fast spin-echo sequence with six gradient strengths, five diffusion times (Δ=10-50ms), and ten diffusion-encoding directions. The remaining imaging parameters are as follows: resolution=187.5 𝜇m isotropic, field-of-view = 13.5⨉9⨉9 mm, diffusion duration 𝛿 = 2.5 ms, and maximum b-value = 2500 s/mm2.Biophysical model: A cylinder-pancake model was utilised in this study as previously described3. To estimate biophysical parameters, the L2-norm between the measured diffusion data and the synthesised signal from the model was minimised using a Levenberg–Marquardt algorithm. All experiments were conducted using an in-house Matlab toolkit (Matlab2019b, MA, USA).
Analysis: Parameters were estimated on one cross-section in the short axis orientation. To avoid the partial volume effect, numerical analysis is conducted in the mid-ventricle wall.
Results
Figure 1 demonstrates the spatial distribution of cardiomyocyte radius and volume fraction in one cross-section in the short axis orientation. Cardiomyocyte radius and volume fraction were higher in the TAC heart by ~17% and ~19% on average in comparison to the control heart, respectively (Table 1). Mean diffusivity (MD) was similar in the control and TAC hearts. Fractional anisotropy (FA) was ~14% lower in the TAC heart.Discussion
At the macroscopic level, the left ventricle (LV) wall thickness was higher in the TAC model. At the cellular level, this increase in cardiac mass could be attributed to the 17% increase in the cardiomyocyte radius in comparison to the control heart. This is in keeping with the suggestion that an increase in cardiomyocyte cell size (i.e. increase in ICV) putatively occurs before the onset of irreversible myocardial fibrosis (i.e. decrease in ICV)5. Further validation using histology data is required to confirm these findings.Conclusion
We quantitated cardiac microstructure in one healthy and one TAC mouse heart ex vivo using a cylinder-pancake model. The estimated biophysical parameters r and ICV could serve as sensitive biomarkers for the characterisation of hypertrophy in the heart.Acknowledgements
This work was supported by the British Heart Foundation (BHF) [grant numbers PG/17/28/32943, PG/19/1/34076 and RG/13/8/30266].References
[1] Hsu E. W, Buckley D.L, Bui J.D, et al. Two-component diffusion tensor MRI of isolated perfused hearts. Magnetic Resonance in Medicine. 2001; 45(6):1039–1045.
[2] Kim S, Chi-Fishman G, Barnett A.S, et al. Dependence on diffusion time of apparent diffusion tensor of ex vivo calf tongue and heart. Magnetic Resonance in Medicine. 2005; 54(6):1387–1396.
[3] Farzi M, McClymont D, Whittington H, et al. Assessing Myocardial Microstructure with Biophysical Models of Diffusion MRI. IEEE Transaction in Medical Imaging. 2021.
[4] Teh I, McClymont D, Burton H, et al. Resolving fine cardiac structures in rats with high-resolution diffusion tensor imaging. Scientific Reports. 2016; 6:30573.
[5] Coelho-Filho O. R, Shah R. V, Mitchell R, et al. Quantification of cardiomyocyte hypertrophy by cardiac magnetic resonance: Implications for early cardiac remodeling. Circulation, 2013; 128(11):1225–1233.