0874
Joint estimation of T2 and diffusion tensor from DW-SSFP using EPG
Julien Lamy1, Mathieu Santin2, and Paulo Loureiro de Sousa1
1ICube, Université de Strasbourg-CNRS, Strasbourg, France, 2Institut du Cerveau, Paris, France
1ICube, Université de Strasbourg-CNRS, Strasbourg, France, 2Institut du Cerveau, Paris, France
Synopsis
The DW-SSFP sequence is useful to attain strong diffusion-weighting on clinical systems while keeping a short repetition time. However, its analytical model does not account for the echo time. Using a 3D EPG model, we show that the echo time plays an important role, and that it cannot be neglected. We then provide a new method to jointly estimate T2 and the diffusion tensor on an ex-vivo fetal brain at 3 T.
Introduction
DW-SSFP1 is a strongly diffusion-weighted sequence with high SNR, high CNR and few artifacts. Although the sequence is conceptually simple, its analytical model2 shows that the steady-state signal depends, in addition to the diffusion coefficient, on both T1 and T2. This analytical model was developed for a stopped-SSFP spectroscopy experiment and hence does not account for the echo time; it is however still the gold standard when estimating the diffusion tensor from DW-SSFP data3. In this abstract, we use EPG simulations4 to explore how the DW-SSFP signal is modified by the TE and to jointly estimate T2 and the diffusion tensor on an ex-vivo fetal brain.Methods
We implemented two EPG simulations of the DW-SSFP sequence using the Sycomore toolkit5. The first one mimics the analytical model and accounts only for flip angle, diffusion weighting and TR; the second one, more complete, accounts additionally for TE, readout bandwidth, and maximum gradient amplitude. Using reference values of an in-vivo adult human brain at 3 T6,7 (T1/T2 = 1084/69 ms, eigenvalues of D=1708/303/114 µm2/s), and timing parameters achievable on a Siemens 3 T Verio system (flip angle 37°, q=200 cm-1, TE/TR = 27/30 ms, bandwidth = 1034 Hz/pixel, Gmax=25 mT/m, resolution 1 mm isotropic), we compared signals simulated using both EPG models with the analytical model (TE=TR, no bandwidth). We then studied the signal simulated using the complete EPG model on a range of T2 (100 ms to 400 ms) and D (0 µm2/s to 2000 µm2/s) at two diffusion weightings (q=12 cm-1 and q=200 cm-1) and on a range of echo times (5 ms to 25 ms). Finally, on an ex-vivo fetal brain (35 gestational weeks, fixation 22 weeks in 4% formaldehyde followed by 30 weeks in tap water), we acquired DW-SSFP images (60 diffusion-weighted volumes with aforementioned parameters plus 6 additional volumes at q=12cm-1 and TE 7/12/17/22/27 ms), a XFL-B1 map8, and a VFA-T1 map9. This data was used in an evolutionary algorithm10 to jointly estimate T2 and the diffusion tensor; our T2 estimation was then compared to a bSSFP-T2 map11.Results
Figure 1 shows the relative error of the two EPG simulations with respect to the analytical model on a set of 60 directions of the diffusion gradient (i.e. different apparent diffusion coefficients of the same diffusion tensor): the simplified EPG simulation closely matches the analytical model, while the complete model shows that the TE indeed has an effect on the signal, with discrepancies reaching 20 %. Figure 2 shows the signal simulated by the complete EPG model versus the echo time for different values of T2 (line color) and of D (marker shape): at low diffusion weighting (q=12 cm-1), the signal depends on T2 but barely on D while at high diffusion weighting (q=200 cm-1), the signal depends on T2, D and TE. On the ex-vivo fetal brain acquisitions, Figure 3 shows the effect of the diffusion weighting on the banding artifacts inherent to SSFP sequences: the artifacts disappear when q is larger than the inverse of the resolution. Figure 4 shows a map of R2 and a map of the axial diffusivity jointly obtained by our estimation method, and Figure 5 shows a joint histogram of the R2 values from the bSSFP map and from our method: our method globally underestimates R2 with respect to the bSSFP map.Discussion
The TE effect (Figure 1) varies with the ADC and hence cannot be only attributed to T2 relaxation: for a precise estimation of biophysical parameters, the TE must be accounted for in numerical models of the DW-SSFP. Our EPG model does include this effect, as well as those of the imaging gradients, albeit at the cost of a higher computational load. The removal of the banding artifact through a diffusion weighting dependent on the resolution can be explained by the spoiling effect of the diffusion sensitization gradient. This however implies that artifact-free images at high resolution will require a larger amount of diffusion weighting, thus complicating the T2 estimation. The lower R2 estimated by our method may be caused by the finite duration of the RF pulse12, further experiments are required to validate the correction proposed12 for SSFP on DW-SSFP. The diffusion tensor estimated by our method also needs to be validated: the aforementioned discrepancy between the analytical model and the EPG simulation, as well as the lack of an obvious equivalence in diffusion weighting between the gold-standard DW-SE and the DW-SSFP are problems that need to be addressed.Conclusion
We have shown that the analytical model of the DW-SSFP does not fully account for the parameters of the sequence and that the TE has an effect on the observed signal; this limitation can be solved by using an EPG simulation of the sequence. The dependency of the DW-SSFP on T2 at low diffusion weightings can be exploited in a multi-parametric fit to compute a joint estimation of the T2 and the diffusion tensor. These results have proven useful in obtaining measures of the diffusion on a ex-vivo fetal brain on a clinical 3 T scanner. The code used in this paper has been published on GitHub13.Acknowledgements
We would like to thank Karla Miller for the DW-SSFP sequence, Cristina Antal for the ex-vivo fetal brain, and the HPC center of the University of Strasbourg for the computing resources.References
1. McNab & Miller. Steady-state diffusion-weighted imaging: theory, acquisition and analysis. NMR in Biomedicine 23(7). 2010.2. Freed et al. Steady-state free precession experiments and exact treatment of diffusion in a uniform gradient. Journal of Chemical Physics 115(9). 2001.
3. McNab et al. High resolution diffusion-weighted imaging in fixed human brain using diffusion-weighted steady state free precession. NeuroImage 46(3). 2009.
4. Weigel et al. Extended phase graphs with anisotropic diffusion. Journal of Magnetic Resonance 205(2). 2010.
5. Lamy & de Sousa. Sycomore: an MRI simulation toolkit. ISMRM 2020.
6. Stanisz et al. T1, T2 relaxation and magnetization transfer in tissue at 3T. Magnetic Resonance in Medicine 54(3). 2005.
7. Pierpaoli et al. Diffusion tensor MR imaging of the human brain. Radiology 201(3). 1996.
8. Amadon et al. Validation of a very fast B1-mapping sequence for parallel transmission on a human brain at 7T. ISMRM 2012.
9. Preibisch & Deichman. T1 mapping using spoiled FLASH-EPI hybrid sequences and varying flip angles. Magnetic Resonance in Medicine 62(1). 2009.
10. Biscani & Izzo. A parallel global multiobjective framework for optimization: pagmo. The Open Journal 5(53). 2020.
11. Jutras et al. Analytical corrections of banding artifacts in driven equilibrium single pulse observation of T2. Magnetic Resonance in Medicine 76(6). 2016.
12. Bieri & Scheffler. SSFP signal with finite RF pulses. Magnetic Resonance in Medicine 62(5). 2009.
13. https://github.com/lamyj/dw-ssfp-fit
Figures
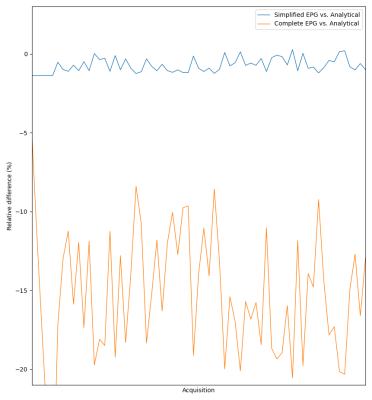
Relative difference of simplified EPG model (blue) and complete EPG model (orange) with respect to the analytical model of DW-SSFP
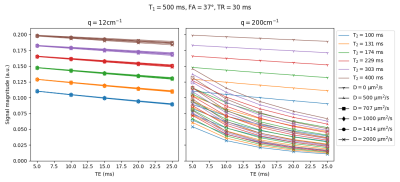
Effect of the TE on the DW-SSFP signal for a range of T2 values (colors) and diffusion coefficients (markers)
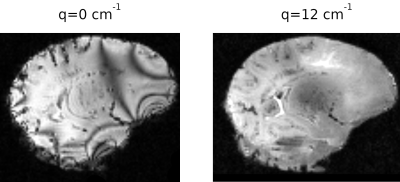
Effect of the diffusion weighting on banding artifacts. Left: no diffusion weighting, banding artifacts visible. Right: diffusion weighting > 1/resolution, no banding artifacts visible
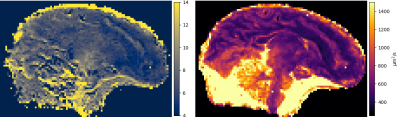
Joint estimate of R2 and diffusion tensor from DW-SSFP. Left: R2 (Hz). Right: axial diffusivity (µm2/s)
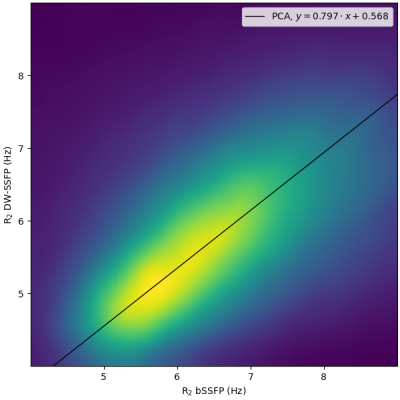
Joint histogram of the R2 values estimated by our method on DW-SSFP and by the reference method on bSSFP
DOI: https://doi.org/10.58530/2022/0874