Introduction

T2-weighted imaging is standard in clinical MR exams, but efficient T2-weighted imaging can be challenging. 3D turbo-spin-echo (TSE) sequences enable volumetric T2-weighted imaging, but have reduced SNR efficiency from complicated echo pathways leading to magnetization loss. Additionally, the long repetition times (TR) required for T1 recovery add to signal acquisition inefficiencies. Alternatively, multi-slice 2D TSE sequences are often used for T2-weighted imaging, such that multiple slices are sequentially excited and imaged in each TR. To achieve reasonable SNR and coverage, however, using a thick slice thickness (~4mm), often with a slice gap, is required for high-resolution scans. The long TR’s, and/or multiple passes, necessary for 2D TSE methods to collect is also SNR inefficient.Here, we present an improved T2-weighted 2D spin-echo (SE) technique that allows contiguous volumetric coverage with improved SNR. This method employs localized quadratic (LQ) encoding, in which a frequency swept RF pulse excites a slab wider than the desired resolution in each excitation.^1,2,3 The quadratic phase accrued over this slab is used to decompose it into thinner resolved slices during reconstruction. This hybrid of 2D and 3D imaging has expected SNR benefits resulting from the averaging effects of this slice encoding scheme, particularly when combined with a long-readout acquisition using (e.g.) spiral trajectories. This study examines application of LQ encoding toward efficient T2-weighted SE imaging.

Methods

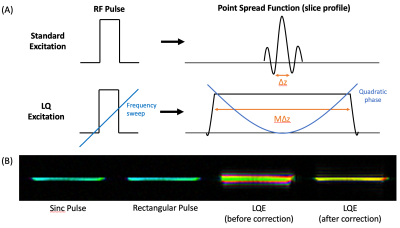
A multi-slice 2D SE sequence was used with a spiral readout acquisition and a rectangular RF pulse and gradient appropriate for a slice resolution of Δz. An RF pulse frequency sweep was added to enable LQ excitation, such that each excited slab, of width MΔz, accrued a quadratic phase along the slice direction(Fig.1A). The refocusing 180˚ pulse was chosen to preserve this LQ encoding and maintain a minimal transition zone. A stack of LQ-encoded slabs was collected with a center-to-center spacing of Δz, and decomposed to a true resolution of Δz during the reconstruction by removing the quadratic phase in the k-space(Fig.1B). These slabs were excited in separate passes with a center-to-center spacing of NΔz, to minimize cross-talk (along with the standard even/odd collection order), where N is the slice spacing in each pass. An ideal M should be chosen relative to N such that all spins contribute signal to each pass.T2-weighted multi-slice SE scans were performed using the LQ excitation sequence at 1.5T and 3.0T MRI scanners, using a 16-channel head coil and stack-of-spiral in-out readout trajectories. All LQ scans were acquired using 2-echo mDixon (for water-fat separation), TE=80ms, TR=3000ms, LQ bandwidth M=N (slice spacing in multi-pass acquisition), FOV=230x230x122-144mm, 4:03-5:51 scan time, 1x1x1mm or 0.75x0.75x1.5mm resolution, no slice-gap, and spiral-in and spiral-out readout durations (𝜏_in/𝜏_out) of 33ms/33ms at 1.5T and 20ms/20ms at 3.0T.The reconstruction processed spiral-in and spiral-out data separately, including corrections for LQ phase, off-resonance blurring, concomitant field, and center frequency drift, before producing a combined image. The SNR was measured at an axial slice within gray and white matter automatically segmented using FMRIB Software Library (FSL)⁴. The noise was measured by 2x-oversampling each readout, and then subtracting the images reconstructed from the even- and odd-samples.

Results

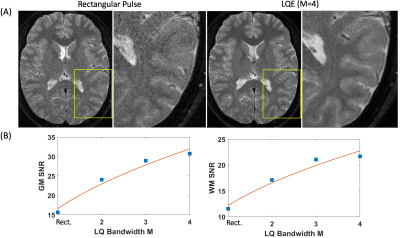
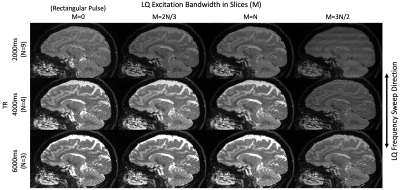
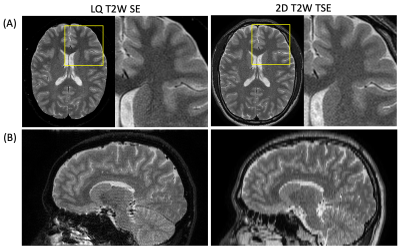
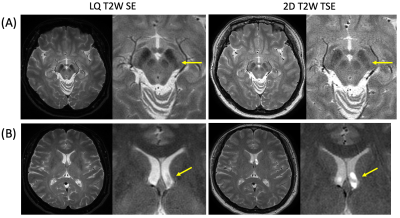
LQ encoding benefits from an increased SNR of $$$\sqrt{M}$$$. This SNR benefit in T2-weighted imaging is illustrated in Figure 2, in which increasing LQ excitation bandwidth (M) yielded higher SNRs. While higher M is favored for higher SNR, exciting slabs which are too wide can create crosstalk with neighboring slices in a given pass. In Figure 3, the LQ excitation bandwidth M was varied relative to the slice spacing N of the interleaved multi-slice acquisition, to illustrate the SNR vs. crosstalk tradeoff of wider slabs as M becomes equal or greater to N.With an identical scan time, the proposed LQ T2W SE scan acquired images with equivalent (or marginally better) SNR as 2D T2W TSE scans (Fig.4A). The high in-plane resolution LQ scans acquired contiguous volumes with no slice gap, while the comparison TSE scan acquired half of the slices, including a slice gap, to achieve an identical scan time. When reformatted on a sagittal plane, the contiguous LQ SE scan yielded finer through-slice details than the 2D TSE.Compared with 2D T2W scans, LQ T2W SE scans also demonstrated increased sensitivity to iron accumulation (Fig.5A). In general, a thin-slice 2D SE scans are sensitive to motion across the slice between the excitation and refocusing pulses. By exciting a thick slab and resolving it to the desired slice solution during reconstruction, LQ SE scans were characterized by decreased motion artifacts in pulsating CSF, when compared with standard 2D SE and TSE scans (Fig.5B).

Discussion

We demonstrated a novel method of T2W SE imaging using localized quadratic encoding. By exciting slabs (of thickness MΔz) that are decomposed into desired slice thickness Δz during reconstruction, this technique yielded an SNR benefit proportional to $$$\sqrt{M}$$$. Whereas spins undergo complicated echo pathways in TSE imaging, LQ T2-SE scans refocuses all excited spins for measurement and employed efficient long spiral-in-out acquisitions. This efficient sampling combined with inherent averaging nature of LQ acquisition allowed the technique to produce high-resolution T2-weighted images with large, contiguous volumetric coverage (no slice gap), in either isotropic (Fig.3) or anisotropic (Fig.4) resolutions.

Acknowledgements

We gratefully receive research support from Philips Healthcare.