0386

Low power free-breathing absolute B1+ mapping in the human body at 7T using magnetic resonance fingerprinting
Max Lutz1, Christoph Stefan Aigner1, Sebastian Dietrich1, Sebastian Flassbeck2,3, Constance G. F. Gatefait1, Christoph Kolbitsch1, and Sebastian Schmitter1,4,5
1Physikalisch-Technische Bundesanstalt, Braunschweig and Berlin, Germany, 2Dept. of Radiology, Center for Biomedical Imaging, New York, NY, United States, 3Center for Advanced Imaging Innovation and Research, New York, NY, United States, 4Medical Physics in Radiology, German Cancer Research Center (DKFZ), Heidelberg, Germany, 5Center for Magnetic Resonance Research, University of Minnesota, Minneapolis, MN, United States

Synopsis

At 7T, power restrictions are a major limitation to accurately map the absolute transmit magnetic field (B1+) in the body. To overcome this, we investigate an absolute B1+ mapping method with low RF power using magnetic resonance fingerprinting (MRF). Measurements are done in a phantom at 3T and in-vivo in the liver at 7T. Resulting maps are compared to the actual flip angle (AFI) method. The obtained results show good agreement between the two methods, while the MRF approach seems to perform better in regions of low B1+ amplitude. Motion robustness introduced by a radial acquisition scheme enables free-breathing measurements.

Introduction

Heterogeneous image contrast due to variations of the transmit (Tx) magnetic field (B1+) is a key challenge at ultrahigh fields (UHF). Accurate but fast mapping of B1+ or the underlying flip angle (FA) is essential for techniques mitigating spatial FA variations such as parallel transmission1. When focusing on the human body at 7T, existing absolute B1+ mapping methods2-5 are often limited by respiratory motion, long acquisition times and especially by radiofrequency (RF) power demand. Recently, we presented a radial phase-encoding (RPE) based, 3D respiration-resolved actual flip angle imaging (RPE-AFI) acquisition6 that addresses some of these challenges. However, in low FA regions the AFI does not yield reliable values6 and increasing the power is not feasible given safety limits and/or peak power amplifier limits (8kW total at our system). Here, to overcome this issue, we aim to quantify absolute B1+ in the body using magnetic resonance fingerprinting (MRF).
MRF has previously been used to quantify B1+ jointly with tissue parameters at 3T in animals7, in humans8-10, and at 7T in the brain11,12, in simulations, phantom experiments and in the abdomen (under breath-hold) with multiple coil modes10,13,14. The proposed approach builds up on Ref 14, however, we aim for free-breathing measurements and a comparison to an established absolute B1+ mapping method (AFI). Due to power constraints, no inversion pulse and only limited FAs can be applied. Given this constraint we quantify B1+ in the human liver at 7T using MRF during free-breathing.

Methods

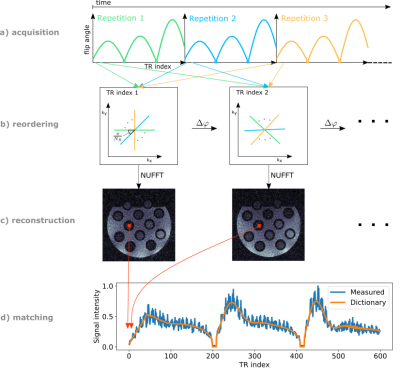
MRF acquisition: A slice-selective radial FLASH sequence15 with SINC-shaped RF-pulses (BWTP=4), radial readout, 117° RF spoiling angle, and a time-varying FA pattern16 with constant TR was used for data acquisition. The number of TR indices (length of the FA pattern) was set to 600 with 32 repetitions of the entire FA pattern being performed. Data acquisition and reordering is demonstrated in Figure 1. Data acquisition was performed from the second repetition onwards to assure identical initial magnetization at the start of each repetition.

Initial phantom experiments were performed at 3T (Verio, Siemens, Germany) using a 12-channel head coil and a multi-compartment phantom (Eurospin II T05 System Phantom17) with different T1-values (range 444-1415ms). Parameters: field-of-view(FOV)=256x256mm2; resolution=2x2mm2; slice-thickness=5mm; TE/TR=2.4/5.0ms; nominal FA=60°; TA=1min39sec.

Subsequently, a healthy volunteer was scanned at 7T (Magnetom 7T, Siemens, Germany) with a commercial 32-element body coil array (MRI.TOOLS, Berlin, Germany) according to an IRB approved protocol. Free-breathing, transverse liver scans were performed with parameters: FOV=384x384mm2; resolution=2x2mm2; slice-thickness=5mm; peak RF voltage=391.6V(limit=550V); TA=1min39sec.

Dictionary calculation and matching: Bloch equations were used to calculate a dictionary of magnetization fingerprints for specified T1 and B1+ values (T1: 30–3000ms, 10ms increments; B1+: 0.01–1.5, increments of 0.01, scaled with nominal FA). 801 isochromats across the slice were simulated to account for slice-profile, gradient- and RF-spoiling. A first FA repetition was used to obtain the initial magnetization for the continuous FA repetition. Dot-product matching was applied.

AFI: The setup for the 3T phantom scan was identical to the MRF phantom acquisition. A slab-selective 3D Cartesian AFI was acquired. Parameters: FOV=256x256x160mm³; resolution=2x2x5mm³; TE/TR1/TR2=1.8/20/100ms; nominal FA=60°; TA=8min15sec. For evaluation, only the centre slice was considered.
For the 7T in-vivo scan the setup was identical to the in-vivo MRF measurement. Here, a 3D RPE-AFI was used6. Parameters: FOV=400x500x500mm³; resolution=5x5x5mm³; TE/TR1/TR2=2.04/15/75ms; peak RF voltage=461.11V; TA=9min36sec. For evaluation, only the centre slice was considered.

Results

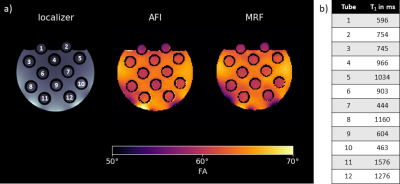
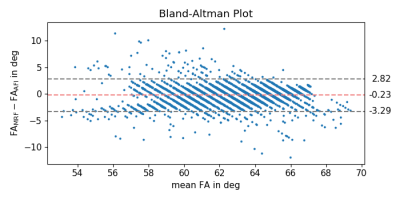
Figure 2 shows a GRE localizer and the measured FA distribution in the phantom at 3T using the MRF and AFI sequence, qualitatively showing high agreement.
Figure 3 provides a quantitative pixel-wise comparison of the AFI and MRF method where a mean difference of -0.23°±1.56° (-0.35%±2.54%) is found.
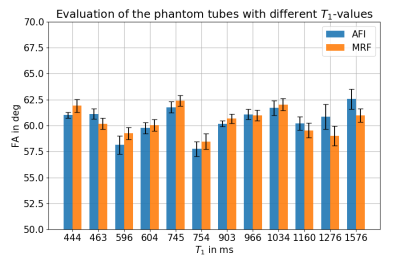
Figure 4 evaluates the FAs for different T1-values in the phantom tubes for both methods. The difference observed is below 1.2°(<2%) for most tubes, only at high T1-values deviations larger than 1.2° (T1=1276ms:1.85°(3.04%); T1=1576ms:1.57°(2.50%)) occur.
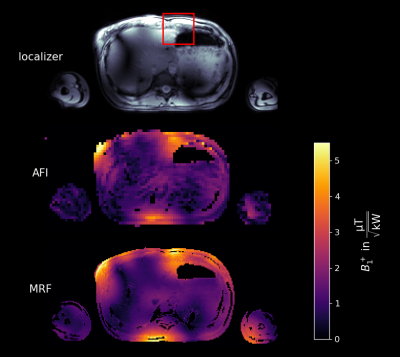
Figure 5 shows the acquired in-vivo B1+ maps at 7T using both, the RPE-AFI and the MRF method. Qualitatively, the patterns obtained by both methods agree, however, as expected, areas with low B1+ result in unreliable FAs and discontinuities in the AFI map. In contrast, these discontinuities are not perceptible in the MRF-based map. In a region of interest (ROI) of higher B1+ values (c.f. Figure 5) the AFI is expected to return accurate values. Here, average B1+ is (3.16±0.70)µT/√kW for the AFI acquisition and (3.58±0.73)µT/√kW for the MRF acquisition, respectively. The mean voxel-wise difference within the ROI is (0.42±0.56)µT/√kW(16.30%±26.19%).

Discussion & Conclusion

While both B1+ mapping methods were performed close to the scanner's SAR/power limits only the MRF-based method is evaluable in large regions of the liver at such low B1+ conditions (<2µT/√kW in the body centre, <4µT/√kW close to the skin). Thus, with MRF, either direct or hybrid B1+ mapping for multi-Tx-channel body coils18 combined with different shim solutions10 may be performed, which was not feasible using the AFI in recent works6. The MRF-results also reflect robustness to motion artefacts likely due to the radial k-space trajectory. In addition, improvements to this initial acquisition scheme are viable such as optimizing the FA pattern using Cramér-Rao Bounds9,19,20 to enhance B1+ sensitivity, possibly yielding shorter acquisition times and/or even higher accuracy of the obtained maps.

Acknowledgements

We gratefully acknowledge funding from the German Research Foundation (GRK2260, BIOQIC and SCHM 2677/4-1).

References

1. Padormo F, Beqiri A, Hajnal JV, Malik SJ. Parallel transmission for ultrahigh-field imaging. NMR Biomed. 2016; 29: 1145- 1161.

2. Sacolick LI, Wiesinger F, Hancu I, Vogel MW. B 1 mapping by Bloch-Siegert shift. MRM 2010; 63: 1315- 1322.

3. Nehrke K, Börnert P. DREAM-a novel approach for robust, ultrafast, multislice B1 mapping. MRM 2012; 68: 1517- 1526.

4. Fautz H, Vogel M, Gross P. B1 mapping of coil arrays for parallel transmission. Proceedings of the 16th Annual Meeting of ISMRM, Toronto, Canada, 2008. p. 1247.

5. Yarnykh VL. Actual flip-angle imaging in the pulsed steady state: A method for rapid three-dimensional mapping of the transmitted radiofrequency field. MRM 2007; 57: 192- 200.

6. Dietrich, S, Aigner, CS, Kolbitsch, C, et al. 3D Free-breathing multichannel absolute B1+ Mapping in the human body at 7T. MRM 2021; 85: 2552– 2567.

7. Buonincontri G, Sawiak SJ. MR fingerprinting with simultaneous B1 estimation. MRM 2016;76(4):1127-1135.

8. Körzdörfer, G, Jiang, Y, Speier, P, et al. Magnetic resonance field fingerprinting. Magn Reson Med. 2019; 81: 2347– 2359.

9. van Riel MHC, Yu Z, Hodono S, Xia D, Chandarana H, Fujimoto K, Cloos MA. Free-breathing abdominal T1 mapping using an optimized MR fingerprinting sequence. NMR Biomed. 2021 Jul;34(7):e4531.

10. Cloos, M., Knoll, F., Zhao, T. et al. Multiparametric imaging with heterogeneous radiofrequency fields. Nat Commun 7, 12445 (2016).

11. Buonincontri G, Schulte RF, Cosottini M, Tosetti M. Spiral MR fingerprinting at 7T with simultaneous B1 estimation. MRM 2017; 41: 1-6.

12. Akasaka, T, Fujimoto, K, Cloos, M, Okada, T. In-Vivo Evaluation of MR Fingerprinting at 7T. Proc ISMRM 27, Abstract 3293 (2018).

13. Cloos, M, Zhang, B, Sodickson, D. Abdominal MRF at Ultra-High-Field Strengths. Proc ISMRM 24, Abstract 0740 (2016).

14. Cloos, M, Paška, J, Yu, Z et al. Abdominal Imaging with Heterogeneous Radiofrequency Fields at 7 Tesla. Proc ISMRM 25, Abstract 1125 (2017).

15. Flassbeck S, Schmidt S, Bachert P, Ladd ME, Schmitter S. Flow MR fingerprinting. MRM. 2019;81(4):2536-2550.

16. Jiang Y, Ma D, Seiberlich N, Gulani V, Griswold MA. MR fingerprinting using fast imaging with steady state precession (FISP) with spiral readout. MRM 2015; 74(6): 1621-31.

17. Lerski RA, de Certaines JD. Performance assessment and quality control in MRI by Eurospin test objects and protocols. MRM 1993;11(6):817-33.

18. Van De Moortele P-F, Ugurbil K. Very fast multi channel B1 calibration at high field in the small flip angle regime. Proceedings of the 17th Annual Meeting of ISMRM, Abstract 367.

19. Lee, PK, Watkins, LE, Anderson, TI, Buonincontri, G, Hargreaves, BA. Flexible and efficient optimization of quantitative sequences using automatic differentiation of Bloch simulations. MRM 2019; 82: 1438– 1451.

20. Bo Zhao, Haldar JP, Congyu Liao, Dan Ma, Yun Jiang, Griswold MA, Setsompop K, Wald LL. Optimal Experiment Design for Magnetic Resonance Fingerprinting: Cramér-Rao Bound Meets Spin Dynamics. IEEE Trans Med Imaging. 2019 Mar;38(3):844-861.

Figures

Figure 1: Overview of the MRF approach: a) Data is acquired with 32 repetitions (NR) of the entire FA pattern (600 TRs). From one TR to the next, a golden angle increment is applied for readout (Δφ≈137.5°) and from repetition to repetition an increment of π/NR is applied, b) all data points with identical TR indices are binned into one k-space, c) an NUFFT is performed to reconstruct the undersampled images, d) for each pixel the dictionary entry that maximizes the dot-product with the measured signal is chosen.

Figure 2: a) Resulting FA maps from the phantom experiment at 3T for the cartesian AFI and the MRF acquisition with nominal FA=60°. b) T1-values of the tubes numbered according to the localizer in a).

Figure 3: Bland-Altman Plot evaluating the pixelwise difference between the AFI and the MRF approach for the phantom experiment at 3T (displayed in Figure 2) with a mean difference of –0.23°±1.56° (-0.35%±2.54%). The streaks perceptible in the plot originate from the discrete nature of the MRF approach, as the FAs of the dictionary are calculated with a finite step size (∆FAdict=0.6°). Only pixels with non-zero values in both methods are evaluated.

Figure 4: Evaluation of the FA in phantom tubes at 3T with different T1-values for the MRF and AFI method. The observed difference is below 1.2° (<2%) for most tubes, only at high T1-values deviations larger than 1.2° (T1=1276ms: 1.85°(3.04%); T1=1576ms: 1.57°(2.50%)) occur.

Figure 5: In-vivo B1+ maps for an axial slice of the liver at 7T for the RPE-AFI and the MRF approach. An ROI is drawn (red rectangle) where the two methods are quantitatively compared (only pixels with non-zero values are considered). In the ROI an average B1+ of (3.16±0.70)µT/√kW for the AFI acquisition and (3.58±0.73)µT/√kW for the MRF acquisition is observed, respectively. The mean voxel-wise difference within the ROI is (0.42±0.56)µT/√kW(16.30%±26.19%).

Proc. Intl. Soc. Mag. Reson. Med. 30 (2022)
0386
DOI: https://doi.org/10.58530/2022/0386