Introduction:

Spiral-based TSE techniques have shown advantages over conventional Cartesian TSE at high fields (3T), in terms of SNR efficiency, improved image contrast, and reduced SAR^1,2. However, for TSE imaging at lower field strengths, concomitant-gradient effects may induce artifacts because Maxwell-terms scale inversely with the field strength. Maxwell-field phase errors induced by differences in spiral readouts along the echo-train may disturb the TSE signal pathway and violate the CPMG condition³, thus resulting in severe signal loss that cannot be recovered in image reconstruction.

Researchers have investigated Maxwell compensation for interleaved-spiral TSE imaging^1,4, and recently Mugler et al. proposed a general method to achieve the compensation of self-squared Maxwell-field terms by modifying gradient-waveforms along the entire echo-train for interleaved-spiral T₂-weighted 2D-TSE imaging with different trajectory types⁴. Promising results show this approach enables Maxwell compensation for spiral trajectory types that are temporally asymmetric, such as spiral-out.

In this work, 2D-TSE imaging using annular-spiral-rings² (SPRING-RIO TSE) was developed that incorporates gradient-waveform modifications to compensate the self-squared Maxwell-terms at the echo time and over echo spacings, with an image reconstruction method to correct for residual Maxwell and B₀-inhomogeneity induced phase accruals along the trajectory.

Methods:

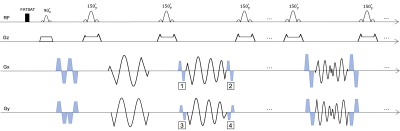
Sequence-based Maxwell field-corrections: The goal of gradient-waveform modifications was to eliminate the phase shift from self-squared terms at the k-space center, and to reduce the difference in phase shifts between echoes targeting a constant phase shift at the end of every echo. The implementation was accomplished (Fig.1) as follows:
1) For each shot, the maximum Maxwell integral $$$M$$$ for each gradient axis was determined from the spiral-ring with the highest gradient amplitude.
2) Bipolar-gradient pairs were added at both the beginning and end of each remaining echo spacing (i.e., two pairs for each axis) to increase the Maxwell integrals. The gradient amplitudes were determined by subtraction of the Maxwell integral $$$M_{i}$$$ for the current $$$i$$$th ring from $$$M$$$.
3) The polarity of one of the four bipolar-gradient pairs in each echo spacing was set to be the opposite of the others for self-balancing the quadratic cross-terms⁵ induced by the added bipolar gradients. Additional time was added to the echo spacing as needed to achieve compensation, and the final Maxwell integral at the end of each echo spacing was designed to be a constant value of $$$\frac{M}{2}$$$.

Reconstruction-based Maxwell field-corrections: The goal of the image reconstruction method was to further reduce the residual phase errors from Maxwell-gradients⁶ and off-resonance effects accrued during the readout.
1) For the axial scan case, the data from each ring was demodulated by multiplying its own time-dependent Maxwell phase shift by the factor of $$$e^{-\phi_c{(z,t)}}$$$, where $$$\phi_c{(z,t)}=\frac{\gamma z^2}{2 B_0}\int(g_x^2+g_y^2)\ dt$$$.
2) For sagittal and coronal orientations, the data was first demodulated as indicated for the axial plane, followed by multi-frequency interpolation⁷ to mitigate the in-plane blurring caused by spatial- and time-dependent Maxwell phase error.
3) A semi-automatic deblurring method⁸ was used for off-resonance correction.

Experiments were performed on 1.5T (MAGNETOM Avanto) and 0.55T⁹ (prototype MAGNETOM Area) MR scanners (Siemens Healthcare, Erlangen, Germany). For phantom and human studies, images were acquired using SPRING-RIO TSE with and without compensation, and Cartesian TSE as a reference.

Results and Discussion:

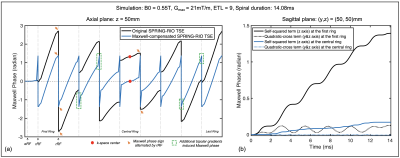
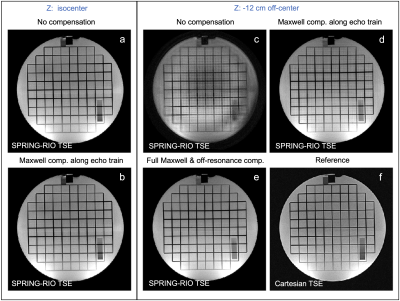
Fig. 2a shows the simulation of the Maxwell phase pathway from self-squared terms along the echo-train for SPRING-RIO TSE with (blue) and without (black) sequence modifications (axial plane). After adding compensation gradients, the accrued phase for each echo spacing starts at $$$-\phi$$$ and ends at $$$\phi$$$, where $$$\phi$$$ is a constant value, and the phase at the k-space center (spin echo) is zero. The green dashed boxes indicate examples of increased Maxwell phase by added bipolar gradients. Fig. 2b shows that the first ring produces the largest Maxwell phase accrual while the central ring has the smallest value. For the sagittal scan example, the self-squared phase terms are substantially larger than the quadratic-cross phase terms.

Fig. 3 illustrates the effectiveness of the proposed Maxwell compensation via sequence modification and image reconstruction in a phantom study at 1.5T. While both uncompensated and compensated axial images show no significant artifacts at isocenter (Fig.3a-b), the uncompensated image (Fig.3c) shows substantial artifacts at z = -12 cm. With Maxwell compensation along the echo-train, no signal loss and reduced artifacts are seen (Fig.3d). Performing further Maxwell and off-resonance phase correction during image reconstruction removes residual artifacts (Fig.3e).

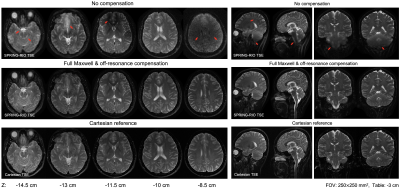
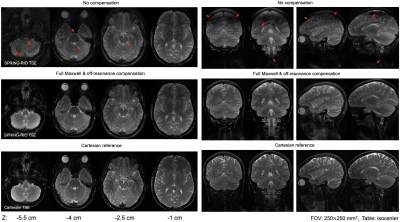
Fig. 4 and Fig. 5 show the image quality obtained in-vivo from SPRING-RIO TSE, with no compensation, or with full Maxwell and off-resonance compensation, compared to that for Cartesian TSE at 1.5T (Fig.4) and 0.55T (Fig.5), respectively. The uncompensated images at different off-center locations and slice orientations show image degradation, while the fully compensated images show similar image quality when compared to Cartesian TSE.

Conclusion and future work:

We demonstrated a spiral-ring T₂-weighted 2D-TSE pulse sequence that incorporates sequence modification and image reconstruction to mitigate the image degradation associated with concomitant-gradient effects at 0.55T and 1.5T. This approach can be extended to compensate Maxwell-gradient-induced effects for TSE imaging with other asymmetric trajectory types. Further studies are needed to determine whether quadratic-cross Maxwell-terms will also require full compensation to obtain high image quality, and to optimize the sequence parameters to improve acquisition efficiency when including Maxwell compensation.

Acknowledgements

The authors would like to acknowledge the assistance of Siemens Healthcare in the modification of the MRI system for operation at 0.55T under an existing cooperative research agreement (CRADA) between NHLBI and Siemens Healthcare. This work was supported in part by Siemens Healthcare.