3963
Universal parallel transmit pulses for a 2-dimensional local excitation target pattern at 9.4T1High-field Magnetic Resonance, Max-Planck-Institute for biological Cybernetics, Tübingen, Germany, 2Biomedical Magnetic Resonance, University Hospital Tübingen, Tübingen, Germany, 3Advanced Imaging Research Center, University of Texas Southwestern Medical Center, Dallas, TX, United States
Synopsis
In this study, the concept of ‘Universal pTx pulses’ for local excitation is tested in vivo at 9.4T. Based on B0/B1+ maps from eight different subject heads, universal pulses for a 2-dimensional local excitation target pattern were designed. The pulses aiming to excite the visual cortex of the human brain (with a flip angle of 90 and 7 degree, respectively), while the remaining areas should experience no effective excitation.
In simulations and in vivo at 9.4T, the resulting universal pules perform just slightly worse compared to the subject specific tailored pulses (on non-database heads).
Introduction
The most flexible approach to overcome inhomogeneity of the radiofrequency field1,2 accompanying operating at ultra high field (B0≥7T) is parallel transmission (pTx). However, pTx pulse design is in general based on a set of calibration scans for each individual subject, which leads to lengthy scan times. Gras et al3 introduced the concept of ‘Universal pTx pulses’ (UPs), which does not require the knowledge of channel-wise B0/B1+distribution in individual subjects, and rather relies on a pre-calculated pulse-database. The literature provides UPs for non-selective and slice selective excitation4,5,6,7, but this concept was never tested for in vivo local excitation applications (simulation studies were presented8,9,10). Local excitation pulses are especially useful, for reduced field-of-view applications, in order to avoid unwanted folding artefacts from surrounding tissues11.In this work, we will design UPs that locally excite the visual cortex area in the human brain for proof-of-principle. The UPs will be compared to subject specific tailored pulses (TPs) and tested in vivo at 9.4T.
Methods
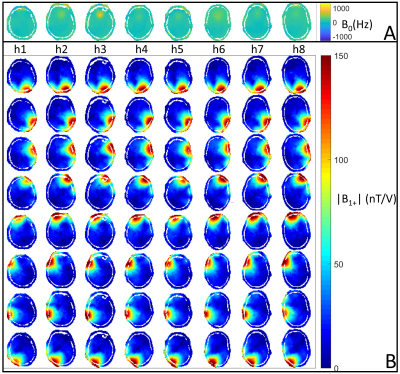
All measurements were performed on a 9.4T whole-body MR scanner (Siemens Healthcare). The utilized RF coil12 consists of eight transceiver surface loops and eight receive-only loops. In order to create a representative database for the UP calculation, whole head B0/B1+ maps (Figure 1) were acquired from eight different subjects with a 3-dimensional presaturated TurboFLASH sequence (satTFL)13.The UPs were designed by means of an extension of the ‘Spatial domain method’14. As described earlier9, the basic idea of this procedure is to include eight instead of one subject head in the optimization problem (Eq. 1):
$$\mathbf{p} _{UP}^* = \underset{\mathbf{p} }{\operatorname{argmin}} \left\{ \left\| \begin{bmatrix}\mathbf{A}_{full,1}\\ \vdots\\ \mathbf{A}_{full,2}\end{bmatrix}\mathbf{p} - \begin{bmatrix}\mathbf{m}_{tar}\\ \vdots\\ \mathbf{m}_{tar}\end{bmatrix} \right\|^2 \right\} \,, $$
where $$$\mathbf{A}_{full,j}$$$, with $$$j = 1, ... , 8$$$, is the full system information matrix of subject $$$j$$$, $$$\mathbf{p}$$$ is the RF pulse shape vector and $$$\mathbf{m}_{tar}$$$ is the target excitation pattern.
The optimization problem in Eq. 1 was solved by using MATLABs (MathWorks, Natick) lsqr-function (20 iterations). The resulting pulse was utilized as an initial guess for the active-set algorithm implemented in MATLABs fmincon-function. The solution was constrained to a maximum pulse amplitude of 130 Volt.
As a reference, TPs were calculated by solving the optimization problem in Eq. 1 with MATLABs lsqr-function (20 iterations) for only one head.
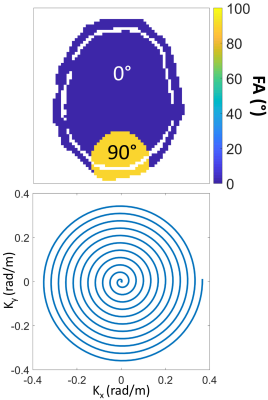
During this study, we focused on a 2-dimensional target pattern $$$\mathbf{m}_{tar}$$$ (Figure 2). Only the central transversal slice of each head was considered during the pulse optimization. We calculated an UP aiming to excite the visual cortex region of the human brain with a flip angle of 90 degree (FA90), while the remaining areas should experience no excitation. Furthermore, by scaling down the FA90 UP, a UP with a flip angle of 7 degree (FA7) was created. The underlying transmit k-space trajectory was a 7.96 ms long 2-dimensional spiral. The pulses were applied in vivo with a Gradient-Echo (GRE) sequence and a satTFL sequence at 9.4T.
Results
Figure 3 shows that the flip angle profiles (simulated with full Bloch equations) for both, the UP and the respective TPs, are very similar (for FA90 and FA7, respectively). In general, the Normalized Root Mean Squared Error (NRMSE) values between the target pattern and the simulated performances of the TPs are slightly lower than the NRMSEs of the UP. For non-database heads, the NRMSE-values of the UP is increased compared to database heads, but the resulting profiles are still similar to the TP-profiles.Confirming the simulations, Figure 4 depicts GRE-acquisitions where UP (FA7) excites the visual cortex area, while the remaining area have very little excitation. The UP results are very similar to the TP results. The TPs outperform UP at the subcutaneous fat tissue regions
The FA-maps acquired at 9.4T (Figure 5) show low excitation in the desired non-excitation areas and reasonable excitation in the visual cortex area. UP and TP (FA90) results are very similar, however there is some lack of FA homogeneity within the excited area visible.
Discussion
In simulations and in vivo the TPs and the UP exhibits comparable performances for FA90 and FA7. As expected, the TPs perform slightly better, than the UP. Considering the non-database heads, the TPs (UP) have a mean NRMSE of 0.035 (0.069) for FA90 and a mean NRMSE of 0.033 (0.069) for FA7. The slight lack in terms of excitation uniformity visible in the satTFL FA profiles (Figure 5) is not visible in the anatomical images (Figure 4) and can therefore be deemed acceptable. The slight excitation of the subcutaneous fat regions next to the visual cortex possibly occurs due to nonlinearities in the scanners gradient system or inaccuracies in the B0/B1+ maps. Applying these pulses in other types of sequences with a reduced field-of-view is a future challenge.Conclusion
With the proposed pulse design routine, it is possible to create a UP for a 2-dimensional target pattern that performs just marginally worse compared to TPs. It is worth noting, that for the TPs a set of calibration measurements combined with the pulse calculation is necessary, while the subject is waiting inside the scanner. In contrast, the UP can be applied directly. This significantly reduces scan time and is an enabling step to transfer pTx to clinical research.Acknowledgements
Funding by the European Union (ERC Starting Grant, SYNAPLAST MR, Grant Number: 679927) the Cancer Prevention and Research Institute of Texas (CPRIT, Grant number: RR180056) is gratefully acknowledged.References
1. Röschmann P. Radiofrequency penetration and absorption in the human body: Limitations to high-field whole-body nuclear magnetic resonance imaging. Medical Physics. 1987 Nov; 14: 922-931.
2. Ibrahim TS, Lee R, Abduljalil AM, Baertlein BA, Robitaille PML. Dielectric resonances and B 1 field inhomogeneity in UHFMRI: computational analysis and experimental findings. Magnetic Resonance Imaging. 2001 Feb; 19: 219-226.
3. Gras V, Vignaud A, Amadon A, Bihan DL, Boulant N. Universal pulses: A new concept for calibration-free parallel transmission. Magnetic Resonance in Medicine. 2016 Feb; 77: 635-643.
4. Gras V, Vignaud A, Rabrait-Lerman C, Le Bihan D, Stoecker T, Stirnberg R, et al. Gain of temporal signal-to-noise ratio with pTx universal pulses and the whole-brain fMRI 3D-GE EPI sequence at 7T. In Proceedings of the International Society for Magnetic Resonance in Medicine Meeting 2018 (Paris), 5440.
5. Gras V, Mauconduit F, Vignaud A, Amadon A, Bihan DL, Stöcker T, et al. Design of universal parallel-transmit refocusing kT -point pulses and application to 3D T2 -weighted imaging at 7T. Magnetic Resonance in Medicine. 2017 Nov; 80: 53-65.
6. Gras V, Pracht ED, Mauconduit F, Bihan DL, Stöcker T, Boulant N. Robust nonadiabatic T2 preparation using universal parallel-transmit kT -point pulses for 3D FLAIR imaging at 7 T. Magnetic Resonance in Medicine. 2019 Jan; 81: 3202-3208.
7. Wiggins C, Poser B, Mauconduit F, Boulant N, Gras V. Universal Pulses for MRI at 9.4 Tesla - a Feasibility Study. In 2019 International Conference on Electromagnetics in Advanced Applications (ICEAA).
8. Geldschläger O, Shao T, Henning A. Universal Parallel Transmit Pulse Design for Local Excitation. In Proceedings of the International Society for Magnetic Resonance in Medicine Meeting 2018 (Paris), 3395.
9. Geldschläger O, Shao T, Henning A. Universal Parallel Transmit Pulse Design for 3-Dimensional Local-Excitation – A 9.4T Simulation Study. In Universal Parallel Transmit Pulse Design for 3-Dimensional Local-Excitation – A 9.4T Simulation Study.
10. Geldschläger O, Herrler J, Nagel A, Henning A. Universal Parallel Transmit Pulse Design for 3-D Local-Excitation based on different sized databases of B0/B1+-maps - A 7T Study. In Proceedings of the International Society for Magnetic Resonance in Medicine Meeting 2020 (Virtual conference), 3699.
11. Stadler A, Schima W, Ba-Ssalamah A, Kettenbach J, Eisenhuber E. Artifacts in body MR imaging: their appearance and how to eliminate them. European Radiology. 2006 Dec; 17: 1242-1255.
12. Avdievich NI, Giapitzakis IA, Pfrommer A, Borbath T, Henning A. Combination of surface and `vertical' loop elements improves receive performance of a human head transceiver array at 9.4 T. NMR in Biomedicine. 2017 Dec; 31: e3878.
13. Bosch D, Bause J, Ehses P, Zaiss M, Scheffler K. Rapid pre-saturated TFL transmit field mapping with an optimized 3D centric single-shot readout. In Proceedings of the International Society for Magnetic Resonance in Medicine Meeting 2020 (Virtual Conference), 3703.
14. Grissom W, Yip Cy, Zhang Z, Stenger VA, Fessler JA, Noll DC. Spatial domain method for the design of RF pulses in multicoil parallel excitation. Magnetic Resonance in Medicine. 2006; 56: 620-629.
Figures