Introduction

As an important biomarker, quantitative T1 mapping was used for various diseases diagnosis [1]. T1 cardiac magnetic resonance (CMR) offered by multitasking [2] is a free-breathing, ECG-free technique featuring high spatial and temporal resolution myocardial T1 mapping, by utilizing low-rank tensor imaging model [2,3] to solve multiple ‘tasks’ at the same time. Despite its ability to produce 2D T1 cine maps in a short 1-min scan, T1 CMR multitasking is capped at 50% efficiency due to scan time spent on navigator readouts that had limited contribution to spatial basis. Navigator lines of a constant k-space trajectory between imaging lines were needed for temporal subspace estimation in standard CMR Multitasking [2]. This abstract presents a new approach to accelerated T1 mapping of the heart under free-breathing and without ECG. The proposed approach is still within the “multitasking” framework, but requires no navigator data. In data acquisition, imaging data was acquired continuously using 2D spoiled-GRE sequence with variable-density spiral trajectories updated with the golden angle. In image reconstruction, the temporal basis is first estimated from the central densely sampled spiral k-space data within a chosen radius. Respiratory motion, cardiac motion, inversion recovery images, and T1 cine maps were then reconstructed using multitasking framework.

Methods

1. Sequence design: A pulse sequence based on 2D spiral spoiled-GRE was developed. Consecutive spiral leaves are rotated by the golden ratio angle of 137.5° such that each new spiral leaf is sampling a substantially different part of k-space compared to the immediately preceding leaf. Imaging Parameters: All data were acquired on a clinical 1.5T scanner (uMR 570, United Imaging Healthcare, Shanghai, China).
Imaging parameters: TR/TE=6.40/0.91ms, Flip angle=5°, FOV=250x250mm², slice thickness=8mm, matrix size = 160x160, 15-channel cardiac coil. The spiral trajectory was designed using the maximum available gradient strength and slew rate, assuming 16 interleaves to cover the entire k-space, and using 4 times undersampling at the peripheral of k-space [5].

2. Gradient delay correction: Spiral readout trajectory was corrected using a gradient system response model that has been previously calibrated. Each spiral trajectory was individually processed for actual trajectory before image reconstruction.

3. Reconstruction: The underlying multidimensional image was represented by a 4-way tensor $$$\mathcal{A} =I(x,c,r,τ)$$$. The first dimension concatenating all image pixel locations and other dimensions indexing cardiac-motion, respiratory-motion, and inversion recovery. The tensor can be decomposed in the partially separable form $$$\mathcal{A}_{(1)} = \textbf{U}_{\textbf{x}} \Phi$$$, where $$$\mathcal{A}_{(1)}$$$ is the mode-1 matricization of $$$\mathcal{A}$$$ and $$$\textbf{U}_{\textbf{x}}$$$ is the spatial basis, $$$\Phi$$$ is the multi-dimensional temporal basis.

Initialization of $$$\underline{\Phi}$$$
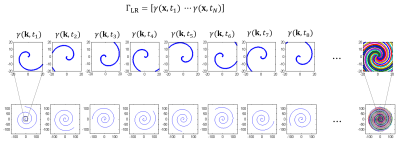
Spiral sampling offers a variable density k-space coverage where the undersampling rate is much lower around k-space center than the outer, as shown in Figure 1. Therefore, a low spatial resolution image from each spiral can be reconstructed and used for temporal basis estimation. [4] The initial temporal basis $$$\Phi$$$ is first estimated from the central part of the undersampled spiral k-space data within a chosen radius. These data can be used to obtain a series of low spatial resolution but high temporal resolution images, represented in matrix $$$\Gamma_{LR}$$$. Then $$$\Phi$$$ can be obtained from the R dominant right singular vectors through the singular value decomposition (SVD).

Calculation of $$$\underline{\textbf{U}_{\textbf{x}}}$$$
$$${\textbf{U}_{\textbf{x}}}$$$ can be recovered by solving the optimization problem: $$\widehat{\textbf{U}_{\textbf{x}}} = \mathop{argmin}\limits_{\textbf{U}_{\textbf{x}}} ||\textbf{d}-\textbf{E}(\textbf{U}_\textbf{x}\Phi)||_2^2+R(\textbf{U}_\textbf{x}) $$ where $$$\textbf{d}$$$ is the acquired imaging data, $$$\textbf{E}(\cdot)$$$ describes multichannel MR encoding with undersampling operator, and $$$R$$$ is spatial regularization operator.

Results

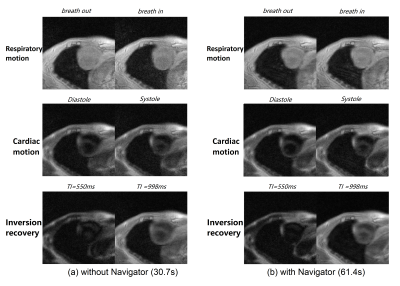
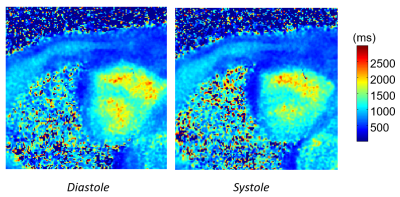
Figure 1 depicts the spiral sampling trajectory used in self-navigated spiral multitasking. The densely sampled central k-space can be used to generate a series of very low-resolution images $$$\gamma(\textbf{x},t_1)\cdots \gamma(\textbf{x},t_N)$$$, which form a low rank matrix $$$\Gamma_{LR}$$$. Figure 2 illustrates typical volunteer cardiac imaging results reconstructed from the proposed method. Figure 3 shows typical diastole and systole T1 maps.

Conclusion and Discussion

To our best knowledge, we have implemented the first spiral, navigator-less, T1 free-breathing cardiac MRI under the framework of multitasking. Compared with traditional radial sampling trajectory, spiral sampling offers the possibility to get ride of navigator data in multitasking, though at an extra cost of increased susceptibility to system imperfections such as gradient delay.

Acknowledgements

This work was partially facilitated by a non-exclusive license agreement between Cedars-Sinai Medical Center and United Imaging Healthcare.