Introduction

B0 inhomogeneity at ultra-high fields poses a significant challenge for many MR imaging and spectroscopy applications. Local arrays of shim coils¹ have gained popularity as a complementary technology to vendor-provided spherical harmonic shim coils due to the increased degrees of freedom for counteracting B0 inhomogeneities with minimal eddy current effects. However, the proximity of these shim coils to RF loops can pose challenges and care should be taken in positioning them to minimize RF interference. Previous work^2,3 used the Rx coils to also carry the DC shim currents, however this restricts the shim coil positions and geometry. On the contrary, separate RF and shim coils provide more freedom for optimisation. This way, by tailoring the geometrical arrangement of the separate shim coils to the brain anatomy, the achievable B0 homogeneity given a finite number of shim coils can be maximized^4-6.

In this work, we investigate the optimal design considerations for a combined 7T RF/B0 head coil. Simulations were performed to characterize the effect of the proximity of shim coils on the RF loops, and bench tests were performed to validate the optimal size and distance between the loops. Finally, with these considerations in mind, we optimised the geometrical arrangement of the local array of shim coils for maximum B0 homogeneity. To the best of our knowledge, such an investigation of local shim coil-to-RF interactions has not been done in the literature before. Previous work either used local shim coils as an add-on to the RF coil and only compared B1 maps⁴ or they combined RF and shim coils and either used the same conductors for RF/DC current purposes^2,3 or used orthogonal shim coils⁷.

Methods

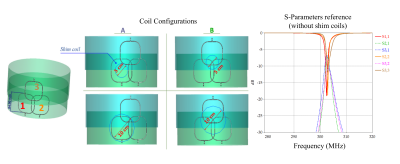
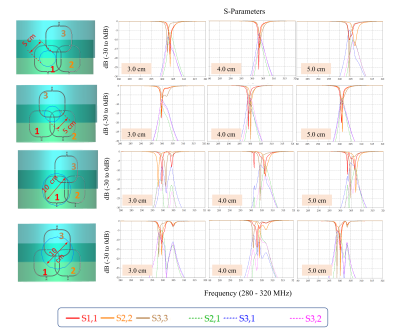
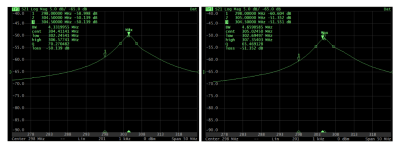
RF Simulations: The effect of the size and position of the shim coil with respect to three circular Rx loops (diameter 84mm) was evaluated using CST-Microwave studio software. Twelve sets of simulations were performed (Figure 1): Two different shim loop diameters of 5.5cm (which is much smaller than the Rx loop diameter) and 10cm (which is close to the Rx loop diameter) were used in two different positions and in each case the gap between the Rx and shim coil was 3cm, 4cm and 5cm. The S-parameters for each setting were evaluated.

Bench Tests: The best shim loop diameter and gap resulting from the numerical simulations were then used to perform bench tests on a 150mm x 200mm x120mm saline phantom to validate the simulation results. Three RF loops were constructed with the following parameters: inner diameter: 60mm, outer diameter: 66mm. A shim coil with a 5cm diameter and 10 turns was also constructed out of copper conductor wire. The S21 parameter with and without the shim coil positioned at the optimal gap was measured using a network analyzer.

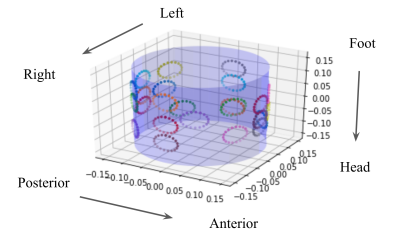
B0 shimming simulations: Finally, given the optimal shim coil radius and gap, the geometrical arrangement of 24 shim coils (5cm diameter, 10 turns) was optimised on a cylindrical holder (30cm diameter, 30cm length) to achieve maximum B0 homogeneity in the brain. A dataset of nine in-vivo B0 maps measured on healthy volunteers at 7T was used. Optimization took 15 mins with a Nvidia K80 GPU (12Gb RAM). Eight of the nine datasets were used in the optimization, and the performance of the optimized design was validated on the other volunteer dataset and compared to a standard 2nd order spherical harmonic shim.

Results

The RF simulations (Figure 2) showed that the smaller shim coil has less interference with the RF loops (less frequency shift and less peak-splitting). On the contrary, the shim coil with similar diameter to the RF loop consistently showed worse performance. Furthermore, simply moving the shim coil further from the Rx loops does not guarantee less interference. The optimal gap was found to be 4cm.

The results of the simulations were confirmed with a bench test where the S21 in the presence of the shim coil with 5cm diameter vs. in the absence of the shim coil were less than 1.2dB different (Figure 3).

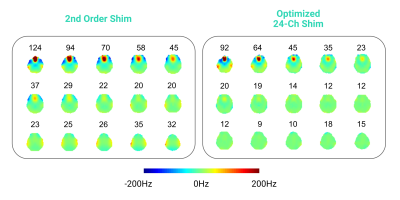
Finally, Figure 4 shows the optimized arrangement for the shim coils. It can be seen that the best performance is achieved when a large number of shim loops are clustered in the challenging shimming regions (e.g. frontal cortex). Figure 5 shows the performance of such a setup on the validation dataset compared to 2nd order spherical harmonic shimming. It can be seen that the optimized arrangement improves the shim quality especially in challenging regions such as the frontal lobe. An average improvement of 43% in standard deviation of the resonance frequencies (averaged across all slices) was observed.

Discussion/Conclusion

We investigated the practical design considerations for a combined RF/B0 shim 7T head coil. Numerical simulations showed that in case of similar Rx and shim loop sizes the coupling will be larger. Hence, for best performance the Rx loops should be larger than the shim coils. In addition, the simulations showed that the optimal gap between the shim and Rx loops is 4cm. With an optimized distribution of the shim loops significant B0 homogeneity improvement can be achieved (43% improvement compared to 2nd order spherical harmonic shimming) with at max 1.2dB loss in MR sensitivity.