INTRODUCTION

High Spectral and Spatial resolution (HiSS) MRI has primarily been applied to breast imaging where it has shown high diagnostic utility for lesion characterization and could serve as a powerful tool for non-contrast enhanced breast cancer screening.^1-3 Successful application of compressed sensing (CS) k-space under-sampling would allow for longer echo trains and thus stronger T2* weighting and/or higher spectral resolution in HiSS MRI, potentially increasing sensitivity and diagnostic performance. Additionally, it would increase utility of HiSS MRI in applicaitons where SENSE acceleration is constrained by coil geometry. Here, we evaluate the effect of CS acceleration on effective image resolution, at acceleration factors of up to R = 10.

METHODS

A two-bottle phantom was prepared from agar gel and vegetable oil, such that a water-fat boundary was created perpendicular to the readout and phase encoding directions (Figure 1), and each bottle was positioned in one of the volumes of a dedicated 15-channel breast coil. Imaging was conducted on a 3T Philips dStream Ingenia scanner (Philips Healthcare, Andover, MA). An axial image through the center of the phantom was selected from a 3D fast-field echo mDixon sequence (FOV 256 x 384 x 36 mm³; spatial resolution 0.8 x 0.8 x 3 mm³; TR/TE1/TE2 40/2.1/3.7 ms, flip angle 12°), with SENSE acceleration factors of 1, 2, 3, 4, 6, 8, and 10. A geometry-matched image was acquired using the High Spectral and Spatial (HiSS) MRI sequence, which is based on a 2D echo-planar spectroscopic imaging sequence (FOV 256 x 384 x 3 mm³; spatial resolution 0.8 x 0.8 x 3 mm³; TR/TE/ΔTE 1000/122/1.89 ms, flip angle 90°; echo train length 127; spectral resolution 4.17 Hz), with the same SENSE acceleration factors. At SENSE acceleration factor of 1, HiSS imaging was done with full k-space coverage on a Cartesian grid and the data for each coil element was exported individually. All acquisitions were repeated five times to allow evaluation of variability and noise levels.

k-space variable-density random under-sampling of HiSS MRI data was simulated by using k-space under-sampling masks, constant for all echoes. Full k-space information and complex gradient echo images at 127 individual TEs were then reconstructed for acceleration factors R of 2, 3, 4, 6, 8 and 10 by using a distributed multi-sensor implementation⁴ of CS⁵ including sparsifying operators in space. A Fourier transform in the temporal direction yielded the proton spectrum in each voxel, and water resonance peak height (WPH) images were constructed.¹

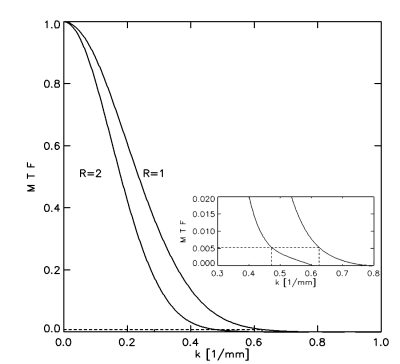
To quantify the effect of the CS algorithm on image quality, WPH signal profiles across the lateral (orthogonal to phase encoding direction) and anterior (orthogonal to readout direction) boundary of the breast phantom were extracted at a representative location, to approximate a sharp edge (Figure 1). The spatial derivatives of the edge profiles were fit to a Gaussian function whose Fourier transform provided the modulation transfer function (MTF). Equivalent image resolution was calculated for each acceleration factor by determining k values at which the MTF equals that of R = 1 at k = 0.625 1/mm (corresponding to the 0.8 mm in-plane resolution), as illustrated in Figure 2. This analysis was also performed on a set of HiSS WPH images obtained with increasing SENSE acceleration factors.

To evaluate noise, a small 50 x 15 voxel area surrounding the readout edge profile was considered. Images from acquisitions 2-5 were normalized to the corresponding images from acquisition 1, removing spatial gradients. The noise was quantified as 1/sqrt(2) of the standard deviation of the resulting image intensity ratio, averaged over acquisitions 2-5.

RESULTS

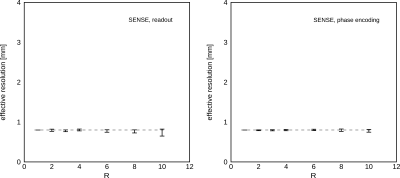
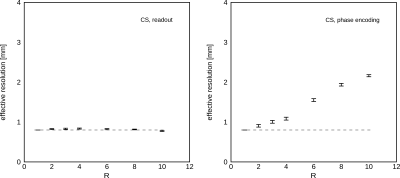
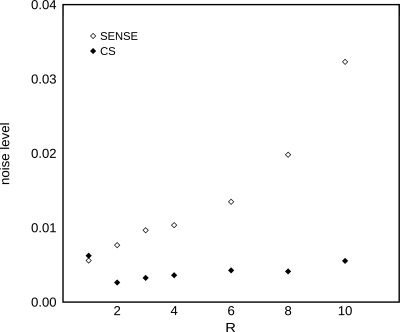
As expected, SENSE acceleration did not degrade spatial resolution, which was nominally 0.8 in-plane (Figure 3). In HiSS MRI data, effective spatial resolution under SENSE reconstruction was approximately constant, at 0.78 ± 0.02 mm in the readout direction, and 0.79 ± 0.01 mm in the phase encoding direction. Effective spatial resolution under CS reconstructions was 0.81 ± 0.02 mm in the readout direction, while in the phase encoding direction it was was 0.80 mm, 0.91 mm, 1.00 mm, 1.08 mm, 1.55 mm, 1.94 mm, and 2.16 mm, for R = 1, 2, 3, 4, 6, 8, and 10, respectively (Figure 4). The noise level remained approximately constant with R for CS-accelerated imaging, while SENSE acceleration significantly amplified noise, approximately 6-fold for R = 10 (Figure 5).

DISCUSSION

When performance in the phase encoding and readout directions are considered, our results indicate that minimal to acceptable blurring due to CS implementation can be expected with acceleration factors up to R = 4. This would allow significant acceleration without the correspondingly increased in-plane resolution loss or noise amplification. SENSE acceleration preserved the spatial resolution robustly, but is known to amplify noise and suffer from geometric artifacts. CS would extend relevance of HiSS MRI to applications such as prostate MRI, where SENSE acceleration factors are limited due to geometry.

CONCLUSION

CS acceleration simulation in HiSS MRI resulted in minimal to acceptable levels of reduction in spatial resolution for acceleration factors of up to R = 4, without noise amplification. Therefore, CS is a promising acceleration strategy for HiSS MRI in noise- or geometrically-constrained applications and for acquisitions with a low number of coil elements.

Acknowledgements

This work was supported by NIH R01 CA167785.