2507

Analysis of common-mode rejection ability and Insertion Loss of Dual band Lattice Balun with non-ideal components

Yue Zhu^1,2 and Xinqiang Yan^1,2
¹Vanderbilt University Institute of Imaging Science, Nashville, TN, United States, ²Department of Radiology and Radiological Sciences, Vanderbilt University, Nashville, TN, United States

Synopsis

A dual band Lattice balun was proposed previously, which holds the promise of removing common mode current for both frequencies in dual-tuned MRI coils with a single interfacing unit. However, the previously fabricated unit had a considerable insertion loss at the proton Larmor frequency. In this work, we analyzed how each lumped element will affect this device's performance and optimized the circuit performance based on the analysis.

Purpose

Dual-tuned MRI coils are highly desired in MRI and MRS to meet the need for acquiring signals from multiple nuclei in the same study [1-3]. Due to the presence of two resonate frequencies, each coil is required to connect cable traps or balun circuits for both frequencies to avoid the cross-talk, reduce the signal-to-noise loss and ensure patient safety [4-6]. The dual-band Lattice balun is a novel method that inherits the benefits of standard Lattice balun (small footprint and easy to integrate with feed board) and meanwhile exhibits the ability to suppress the common mode current at the Larmor frequencies of both nuclei [7]. However, in dual-band Lattice balun, there are up to 8 lumped elements that complicate the practical fabrication and may degrade the performance due to non-ideal components. To better understand such a dual-band balun and give guidance to practical fabrication, in this work, we investigated how the balance of two branches and the circuit's insertion loss will be affected by using non-ideal components (up to 5% tolerance).

Methods

Figure 1 shows the schematic of the dual-band balun [1]. It consists of two branches from balanced ports to the unbalanced port. It can be seen as a first-stage 90-degree lattice balun at each frequency.
EM Simulation setup
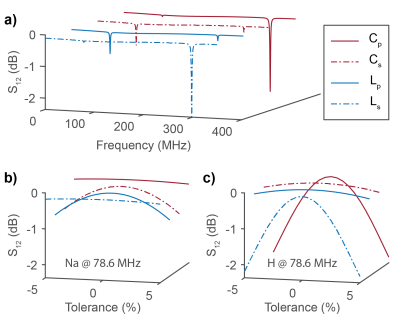
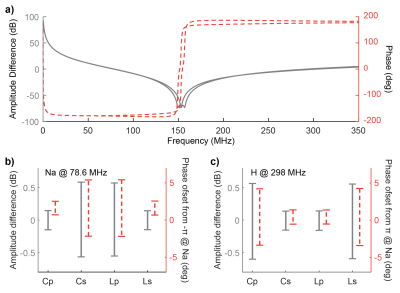
We simulated a dual-band balun circuit for a 7 T proton (Larmor frequency 298 MHz) and sodium imaging (Larmor frequency 78.6 MHz) with the Ansys EM package (Designer, Ansys, Canonsburg, PA). Inductors were set with a serial resistance (0.3 Ohm at 298 MHz, 0.6 Ohm at 78.6 MHz) based on the manufacturer's datasheet. We varied two copies of each component in two different ways, either multiplying the variation factor both from 0.95 to 1.05 or oppositely multiplying these factors, i.e., one from 0.95 to 1.05 while the other from 1.05 to 0.95. The balun's insertion loss was evaluated directly by the transmission coefficient (S₂₁) between the balanced and unbalanced ports. The common-mode rejection ability was evaluated indirectly by the amplitude and phase balance between two balanced ports.
Fabrication and Evaluation
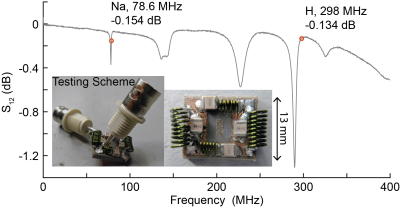
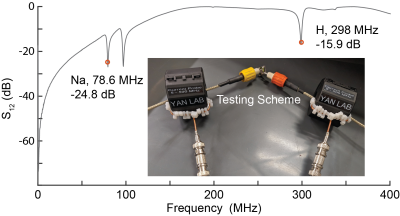
An improved miniatured dual-band balun (dimension 12.75 x 15.15 mm²) was fabricated on a Rogers CUCLAD 217 double-side board. All lumped elements' values were adjusted based on the finding in simulation results (described later) to achieve a high common-mode rejection ratio (CMRR) and low insertion loss. The values of practical components are: L_p = 82 nH, L_s = 39 nH, C_p = 15 pF, C_s = 30 pF, while the calculated values are: L_p0 = 14.5 nH, L_s0 = 36.3 nH, C_p0 = 14.5 pF, C_s0 = 29.8 pF. Capacitors were chosen from a pool of 5%-tolerance high-Q and non-magnetic capacitors (1111C series, PPI, Huntington, NY). Inductors are modified from air-core RF inductors from CoilCraft (Cary, IL). The insertion loss and CMRR were tested by a calibrated four-port Vector Network Analyzer (Keysight, Santa Rosa, CA).

Results and Discussion

Simulation
Partial simulation results are shown in Figure 2 (insertion loss) and Figure 3 (CMRR). Varying lumped elements in the opposite direction (for example, increasing L_p in one branch while decreasing it in the other branch) led to higher insertion loss, as shown in Figure 2a. Figures 2b and 2c show the insertion loss at sodium and proton frequency with different element value variation levels. It is found that Cp and L_s values mainly impact the insertion loss at proton frequency, while C_s and L_p affect the sodium frequency more. A similar phenomenon was found with the CMRR simulation. As shown in Figure 3, C_p and L_s determine CMRR at proton frequency, while C_s and L_p are more prominent for sodium frequency. These results are expected by examining the impedance equations. The $$$1/j\omega C_s$$$ and $$$1/j\omega L_p$$$ terms are more effective at low frequencies while $$$j\omega L_s$$$ and $$$j\omega C_p$$$ are more pronounced at high frequencies.
Fabricated device and bench test
The measured insertion loss and CMRR of fabricated balun are shown in Figures 4 and 5, respectively. Since the discrete values from commercial parts differ from that of the calculated value, the inductors are varied empirically to meet the desired results. Based on the simulations and equations shown above, increasing Lp's values will increase the frequency of the CMRR peak for Na while Ls for H and vice versa. The resulted insertion losses are -0.154 dB for Na and -0.134 dB for H. The CMRRs are 24.8 dB for sodium and 15.9 dB for proton. These combined results showed that this dual tuned balun is low loss and has high CMRR at desired frequencies. It is also easy to fabricate the balun for other frequencies.

Conclusion

We have constructed a high-performance dual-band Lattice balun with the guidance of circuit analysis and optimization. This balun has a low insertion loss of -0.154 dB (sodium) and -0.134 dB (proton) and a high CMRR of 24.8 dB (sodium) and 15.9 dB (proton).

Acknowledgements

This work was supported by NIH R21 EB029639.

References

1. Shen G X, Boada F E, Thulborn K R. Dual-frequency, dual-quadrature, birdcage RF coil design with identical b1 pattern for sodium and proton imaging of the human brain at 1.5 T. Magn Reson Med 1997;38: 717–725.

2. Wiggins G C, Brown R, Fleysher L, Zhang B, Stoeckel B, Inglese M, Sodickson D K. A nested dual frequency birdcage/stripline coil for sodium/proton brain imaging at 7T. In Proceedings of the 18th Annual Meeting of ISMRM, Stockholm, Sweden, 2010. Abstract 1500.

3. Shajan G, Mirkes C, Buckenmaier K, et al. Three‐layered radio frequency coil arrangement for sodium MRI of the human brain at 9.4 Tesla. Magnetic resonance in medicine 75.2 (2016): 906-916.

4. Dabirzadeh A, McDougall M P. Trap design for insertable second-nuclei radiofrequency coils for magnetic resonance imaging and spectroscopy. Conc Magn Reson B. 2009;35B:121–132

5. Wilcox M, Wright S M, McDougall M P. Multi-Tuned Cable Traps for Multinuclear MRI and MRS. IEEE Transactions on Biomedical Engineering. 2019 Aug 7;67(4):1221-8.

6. Enríquez Á G, Vincent J M, Rispoli J V. Dual-Tuned Removable Common-Mode Current Trap for Magnetic Resonance Imaging and Spectroscopy. In2019 41st Annual International Conference of the IEEE Engineering in Medicine and Biology Society (EMBC) 2019 Jul 23 (pp. 6802-6805). IEEE.

7. Sappo C, Grissom W A, Gore J C, Yan X. Dual Band Lattice Balun for Multinuclear MRI. In 2020 Annual meeting of International Society of Magnetic Resonance in Medicine. P 4066.

Figures

Figure 1. Diagram of the dual tuned balun and approximations for different nuclei. The dual-band balun approximates two different single-band baluns at different frequencies, making it suitable for dual-band detection systems.

Figure 2. Simulated insertion loss in differential mode by varying only C_p, C_s, L_p, or L_s. The two elements of the same type in the network are varied reversely, i.e., one increase and one decrease. a) Insertion loss in the frequency domain by changing the element values by ± 5%. b, c) Insertion loss at Na frequency of 78.6 MHz (b) and 298 MHz (c).

Figure 3. Simulated common-mode amplitude differences and phase offsets. a) The frequency response of amplitude differences (solid grey line) and phase (red dashed line) between Z_L to ground when changing one L_s±5% while the other one ∓ 5%. The general shape is similar when changing other elements. b, c) Amplitude differences and phase offset from (b) -180 degrees at 78.6 MHz and (c) 180 degrees at 298 MHz when varying the element's value up to 5%.

Figure 4. Fabricated device and tested insertion loss. The insertion loss is less than -0.2 dB for both nuclei. The insets show the testing scheme and the top photo of the balun circuit. The circuit is miniaturized and measures 12.75 mm by 15.15 mm.

Figure 5. Common mode rejection ratio test with current probes. The CMRR shows a good rejection ratio at sodium and proton frequencies. The two current probes are connected to the VNA while the ground of the two cables from the balun circuit connects to a large resistor.

Proc. Intl. Soc. Mag. Reson. Med. 29 (2021)

2507