0912
A Deselecting Alias Approach to Volumetric Zoomed Imaging
Nicolas Arango1, Molin Zhang1, Jason Stockmann2,3, Jacob White1, and Elfar Adalsteinsson1,4
1Department of Electrical Engineering and Computer Science, Massachusetts Institute of Technology, Cambridge, MA, United States, 2A. A. Martinos Center for Biomedical Imaging, Massachusetts General Hospital, Charlestown, MA, United States, 3Harvard Medical School, Boston, MA, United States, 4Institute for Medical Engineering and Science, Massachusetts Institute of Technology, Cambridge, MA, United States
1Department of Electrical Engineering and Computer Science, Massachusetts Institute of Technology, Cambridge, MA, United States, 2A. A. Martinos Center for Biomedical Imaging, Massachusetts General Hospital, Charlestown, MA, United States, 3Harvard Medical School, Boston, MA, United States, 4Institute for Medical Engineering and Science, Massachusetts Institute of Technology, Cambridge, MA, United States
Synopsis
Reduced FOV imaging using ∆B0 control is limited by magnetostatics, but careful tracking of aliasing enables the vast reduction in the deselection volume required. A 2-sided spectral approach for the deselection enables the combination of strong linear gradients and adaptable multicoil shim array ∆B0 fields to perform selective excitation in concert. Simulations indicate potential for central volume rFOV where prior work in ∆B0-based rFOV imaging was limited to peripheral volumes.
Introduction
Multi coil ∆B 0 field control has been used for reduced field of view (rFOV) imaging to enable higher resolutions, lower acquisition durations, or higher SNR [5]. However, in comparison to RF pulse based inner volume imaging, capable of arbitrary excitation patters [1-4], potential ∆B 0 rFOV imaging volumes are limited by magneto-statics and the maximum field strength of the shim array used.In prior ∆B 0 based rFOV imaging, ∆B 0 fields were optimized to place the target region of interest (ROI) into the excitation band of an RF pulse while excluding as much of the remaining volume as possible.These techniques prove effective for target volumes at the periphery of the subjects anatomy, but are ineffective for interior volumes.Theory
We have developed a method for zoomed, or reduced field of view, volumetric imaging excitation by deselecting portions of a volume that would alias into the ROI within a reduced FOV. Imaging with a 3D phase-encoded acquisition, signal aliasing into a rFOV acquisition fall on a regularly spaced grid in the plane formed by the two phase encode directions. Oversampling in the readout direction adds no additional encoding burden and preventing the need for signal deselection in the readout direction. Figure 1 shows a comparison of the deselected volumes in a rFOV imaging scheme with a spherical ROI in the center of the phantom head with a left-right readout direction.We control the excitation of the ROI and the deselected regions through ∆B 0 control using a spectral separation approach. The magnetic field in target ROI is constrained within the bandwidth of a fixed RF pulse. At the same time the deselected volume is constrained to frequencies outside of the RF pulse bandwidth. In this work we explore both 1-sided and new 2-sided approaches to spectral separation for selective excitation using the optimization formulation shown in figure 5.
Figure 2 shows an example simulation of an excited volume from the one-sided optimization approach with a multicoil shim array. The approximately hyperboloid excited region does not take advantage of the phase-encode-plane alias pattern. While the this approach makes good use of the multicoil shim array’s degrees of freedom, shim current constraints resulting in bounded ∆B 0 field amplitude limit the size of the rFOV.
By taking a two-sided approach, as shown in the optimization problem 5b) we construct an excited volume that encloses the ROI and passes between the aliasing regions. In the case of a sufficiently large rFOV, a slab excitation, entirely formed by the linear gradients, can pass between the aliasing regions, an example of which is shown in 2D in figure 3
Methods
Numerical experiments to asses the proposed rFOV excitation method were performed on data from a human head phantom. The target ROI was a 2 cm diameter sphere placed in the center of the head. ∆B0 rFOV fields were optimized with a fixed SLR designed RF pulse with a 1 kHz flip band and 100 Hz transition bands. The minimum rFOV volume was assessed by growing an infeasible small rFOV until the constraints from equation 5b) were satisfied. Shim fields from the AC/DC 32 channel RF Rx and ∆B0 shim coil were optimized with a 3.5 amp current limit. Linear gradients were optimized with field limitations from the Siemens Prisma 3T scanner. Due to the 1 kHz bandwidth of the fixed RF pulse, the linear gradient constraints were never active. The convex optimization problem was posed in YALMIP and solved in MOSEK in under 2 seconds.Results
Figure .3 shows a sagital slice of the simulated excitation and resulting rFOV aliasing in three zoomed imaging cases. With only linear gradients a large rFOV is required to keep aliased signal out of the ROI. If the rFOV is shrunk without expanding the ∆B 0 control basis parts of the volume that should be deselected are incidentally excited resulting in aliasing into the ROI. When multicoil ∆B0 field control is added to the linear gradients, the slab shape is perturbed to fit between the deselection volumes.Figure .4 shows the excitation volume and deselection regions for the minimum size rFOV in both the one-sided and two-sided cases. The curved slice surface carves between the deselection volumes assigned to opposite sign (indicated in red and blue). By exploiting the aliasing pattern in this way, simulation indicates a reduction of the required phase encoding area from 10.1 cm2 to 8.3 cm2 .By defining an rFOV prior to optimization of ∆B0 based selective excitation for zoomed imaging, we are able exploit the aliasing pattern of linear phase encoded imaging to improve zooming performance. By using a 2-sided spectral separation optimization approach, we are able to leverage the high ∆B 0 field generation of the built-in linear gradients perturbed by a smaller field producing multi-coil shim array.Presently, deselected region sign is selected manually to minimize the rFOV. Future work will enable algorithmic sign selection for ease of workflow in phantom and in vivo experiments.The alias-based deselection region presented here minimizes the total volume of deselection and may bound the capabilities of static ∆B0 based zoomed imaging techniques.
Acknowledgements
MathWorks Engineering FellowhipReferences
- Feinberg A, Hoenninger JC, Crooks LE, Kaufman L, Watts JC, Arakawa M. Inner volume MR imaging: technical concepts and their application. Radiology 1985;156:743-747.
- Luo Y, de Graaf RA, Delabarre L, Tannus A, Garwood M. BISTRO: an outer-volume suppression method that tolerates RF field inhomogeneity. Magn. Reson. Med. 2001; 45:1095-1102.
- Finsterbusch J. Simultaneous functional MRI acquisition of distributed brain regions with high temporal resolution using a 2D selective radio frequency excitation. Magn. Reson. Med. 2015;73:683-691.
- Davids M, Schad LR, Wald LL, Guerin B. Fast three-dimensional inner volume excitations using parallel transmission and optimized k-space trajectories. Magn. Reson. Med. 2016;76(4):1170-1182.
- Stockmann J, Arango N, Poser B, Witzel T, White J, Wald L, Polimeni J. Spatially-selective excitation using a tailored nonlinear ΔB0 pattern generated by an integrated multi-coil ΔB0/Rx array. ISMRM 2018, p.0170
Figures
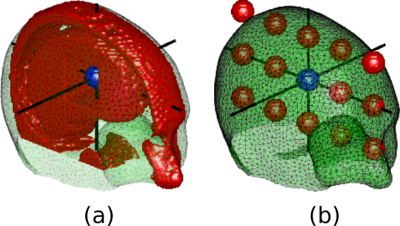
Figure 1. Excited volume from one-sided zoomed imaging excitation and optimized b) ΔB0 field. The Hyperboloid excitation volume does not take advantage of the don't care regions introduced by the alias deselection mask.
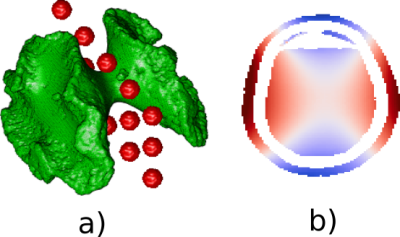
Figure 2. Excited volume from one-sided zoomed imaging excitation and optimized b) ∆B0 field. The Hyperboloid excitation volume does not take advantage of the don’t care regions introduced by the alias deselection mask.
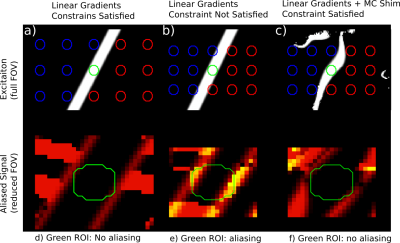
Figure 3. Sagital slice of excitation (a,b,c) and rFOV aliasing pattern (d,e,f) from Linear gradient optimized and linear gradients plus multicoil shim optimized rFOV excitation. (a,d) Linear gradients can select an off-axis slab between aliases with a sufficiency large rFOV. (b,e) Tighter rFOVs cannot be achieved with linear gradients only.(c,f) Multicoil shim array in conjunction with linear gradients achieves tighter rFOV.
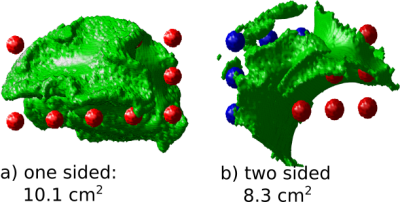
Figure 4. 3D comparison of excited volumes with a) 1-sided and b) 2-sided deselection regions. With the same multicoil shim array current limits (3.5 A), the 2-sided strategy achieves a tighter phase encode planer FOV (8.3 cm2 phase encodes) compared to the one sided strategy (10.1 cm2 ).

Figure 5. Optimization formulation for a) 1-sided and b) 2-sided selective excitation. Matrices A are the shim basis for the excitation region, lower spectral region, and upper spectral region. Shim currents, x, are chosen to keep the ∆B0 field of the excitation volume within the rf pulse bandwidth ∆fe while the lower and upper deselected regions are set bellow and above the RF pulse’s transition bands (of width ∆ftb ) respectively.