Target audience

MRI gradient designers and safety researchers

Purpose

Peripheral Nerve Stimulation (PNS) significantly limits the usability of the latest generation gradient coils in humans [1-3] where rapid gradient slewing induces strong E-fields that can excite nerves. We recently applied PNS optimization to whole-body and head-only gradients [4-5] using a single body model (adult female) and single body position (head-first supine brain imaging). This approach yielded up to 2-fold PNS improvements at the cost of moderate inductance and linearity penalties. It was not clear, however, that this improvement extends to non-head imaging applications. Here we apply a Huygens’ surface approach [6] to enable systematic characterization of PNS performance in a wide range of body positions/orientations and an additional body model in a matter of seconds.

Methods

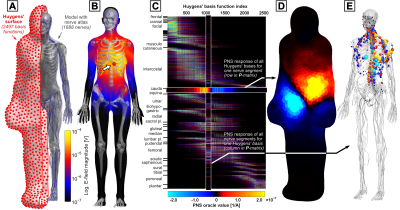
Huygens P-matrix approach: The PNS analysis was performed using a linear PNS matrix (P-matrix) for a current basis function set defined on a Huygens’ surface tightly enclosing one of our body models [7,8]. The PNS responses are precomputed for all basis functions on the Huygens’ surface (small current loops, Fig. 1A). For each current basis, we simulate the induced E-fields and extract the linear PNS oracle values [9] (reciprocal PNS thresholds) along all nerves (Fig. 1A/B). After precomputation and assembly of the full Huygens’ P-matrix (Fig. 1C) which takes a couple of days per body model, the Huygens’ P-matrix can be mapped to a new discrete coil winding or coil geometry and patient position/pose, yielding a coil-specific P-matrix. This mapping only takes seconds, as it relies on fast Biot-Savart calculations of the magnetic fields and solving a small linear system of equations.
Studied coil solutions and body positions: We analyze previously described Y-axis body gradients designed with 5% field non-linearity [5]. We focus our analysis on one conventionally designed coil without PNS optimization (coil B1), and one coil that was optimized with a PNS constraint from the female model in a head-imaging position (coil B2). We evaluate these coils in both female and male models in both head-first and feet-first body orientations for a variety of body z-positions ranging between −40 cm to +120 cm in 5 cm steps (z = 0 cm represents head at isocenter).

Results

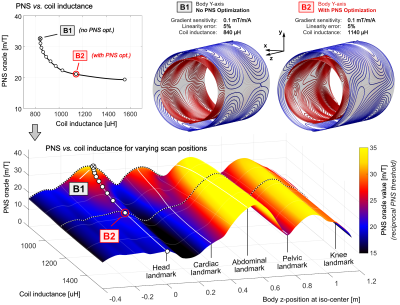
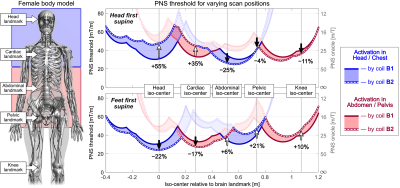
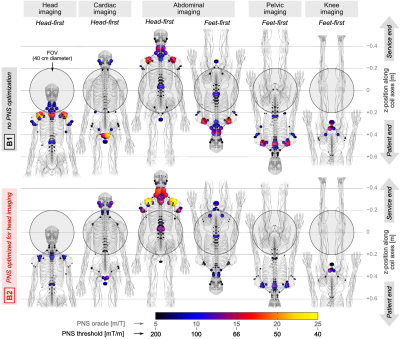
Figure 2 (top) shows the tradeoff L-curve between the maximum PNS oracle (reciprocal PNS threshold) and coil inductance for body Y-axis gradients optimized for the female model for head imaging (head-first supine). Every point on the L-curve is a winding solution, including the two coils B1 (without PNS optimization) and B2 (with PNS optimization). Figure 2 (bottom) extends the PNS analysis to show how the coils on the L-curve perform for other patient positions. The additional dimension corresponds to a new z-position of the female model in the coils. Optimization of the coils for head imaging (head-first supine) led to 35% PNS oracle improvements (55% higher thresholds) for that body position, but also improved PNS characteristics for cardiac imaging (25% PNS oracle reduction, 35% increased PNS threshold).
Figure 3 shows a more detailed PNS threshold characterization of the unoptimized coil B1 (solid curve) and the optimized coil B2 (dashed curve) as a function of position and orientation (including feet-first). Regions where the solid line lies above the dashed line indicate use-cases that retain some benefit from the PNS optimized design B2. The color corresponds to the origin of the nerve activation in the body (blue: head/chest; red: abdomen/pelvis).
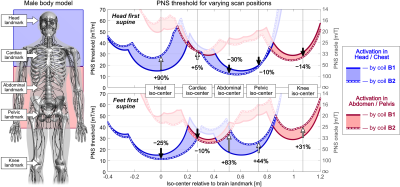
The optimized coil B2 achieves higher PNS thresholds for head/cardiac imaging and lower thresholds for abdominal/pelvic/knee imaging. Note that the origin of PNS changes multiple times between head/chest (blue ROI) and abdominal/pelvic activation (red ROI). The PNS performance for abdominal/pelvic/knee imaging is improved by switching to the more common feet-first orientation (Fig. 3, bottom), at the cost of PNS degradation for head/cardiac imaging (which would not be done feet-first). Figure 4 shows a similar analysis for the male body model. Here, using coil B2 with the head-first orientation showed PNS improvements for head/cardiac imaging (+90% and +5%, resp.), but degraded PNS performance for head-first abdominal/pelvic/knee imaging (thresholds lowered by 30%, 10%, and 14%). These results are qualitatively similar to those found in the female model, although PNS thresholds varied more strongly with body position for the male model.
Figure 5 shows maps of PNS hot-spots (sites of strongest activation) in the female model for both coils B1 and B2 at common clinically used body locations and orientations. The distribution of activation patterns was similar in many cases, with typical activation sites in the shoulders, arms, intercostal nerves, neck, facial and pelvic area nerves. In all cases (except for the head-first abdominal imaging case), the optimized coil B2 improved PNS performance (increased PNS thresholds) compared to the unoptimized coil B1, even though this coil was only optimized for head-first supine head imaging.

Conclusion

We applied the recently described Huygens’ P-matrix approach to characterize whole-body gradients for varying scan positions and body orientations. The data demonstrates that gradient coils optimized for a specific scan position also improve PNS for other imaging applications, underscoring a robust ability of PNS optimized gradient coil windings to provide PNS improvement in a wide range of imaging applications.

Acknowledgements

The authors would like to acknowledge the help of past and present members of the gradient coil group at Siemens Healthineers, including Peter Dietz, Gudrun Ruyters, Axel vom Endt, Ralph Kimmlingen, Eva Eberlein, and Franz Hebrank. Research reported in this publication was supported by the National Institute of Biomedical Imaging and Bioengineering, and the National Institute for Mental Health of the National Institutes of Health under award numbers R24MH106053, U01EB026996, U01EB025162, U01EB025121, R01EB028250. The content is solely the responsibility of the authors and does not necessarily represent the official views of the National Institutes of Health.