4497
Validation of NMR transient subdiffusion in controlled samples1Physics Dpt. Sapienza, CNR ISC, Rome, Italy, 2Physics Dpt., Sapienza University of Rome, Rome, Italy, 3Physics Dpt. Sapienza Roma, CNR ISC, Rome, Italy, 4Anatomical, Histological, Forensic Medicine and Orthopedics Sciences, Sapienza University of Rome, Rome, Italy, 5Drug chemistry and technology department, Sapienza University of Rome, Rome, Italy
Synopsis
The quantification of transient anomalous diffusion (tAD) parameters by NMR could provide higher sensitivity, resolution and complementary detailed information for detecting early changes due to pathological conditions than the conventional metric does. However, there are some questions and controversial issues which prevent the take-off of tAD NMR investigations in soft condensed matter and medical diagnostic field. To overcome these obstacles, we planned to investigate the potential and limits of NMR subdiffusion in calibration standards characterized by porous structured materials and diffusing probes matching or not characteristics length-scales of the porous matrix.
Introduction
Biological tissues are complex environments with dissimilarity length scales of intra and extracellular diffusion compartments, submicroscopic traps and barriers hindering water diffusion. The normal diffusion of bulk water in tissues, characterized by MSD ~t, is not able to capture all these detailed features, providing nonlocal, diffusion measurement averaged on a length-scale approximately equal to MSD1/2 of the diffusing particles. This length-scale (of about 10-20µm) that defines the intrinsic diffusion NMR resolution, is still one-two orders of magnitude greater than the submicroscale tissue structure, where physiological and early pathological processes occur. All the aforementioned features can be captured using a transient anomalous diffusion (tAD) description for which MSD is not linearly proportional to its diffusion time1,2,3. In particular, subdiffusion is characterized by MSD ∝ tα with α< 1. The quantification of tAD parameters could provide higher sensitivity, resolution and complementary detailed information for detecting early changes due to pathological conditions than the conventional metric does. However, there are some questions and controversial issues which prevent the take-off of tAD NMR investigations in soft condensed matter and medical diagnostic field. To overcome these obstacles, we planned to investigate the potential and limits of NMR subdiffusion in calibration standards characterized by porous structured materials. Varying sample microstructural characteristics, and observing the consequent modifications of the theoretical, simulated and experimental signal, it is possible to understand and reveal the fundamental relation between tAD and the underlying structure. In this work we take into account the CTRW description of tAD1,2,3 and the consolidated D(t) theory4,5,6 to investigate porous samples characterized by few and simple geometric and topological features with diffusing particles of three different order of magnitude in diameter to match or not some pores-traps in porous matrices.Materials and Methods
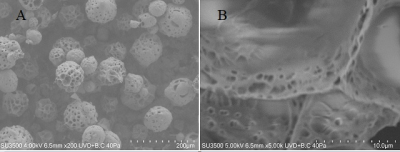
Packed monodispersed and poly-dispersed polystyrene beads (Microbeads AS, Norway) in water and Sephadex hydrogel (Sigma, St. Louis, MO) samples were used as porous matrices. Water, (B12F12)-- and liposomes, characterized by a diameter of about 0.2nm, 2-3nm and 100nm were used as diffusion probes. 100nm-unilamellar liposomes formed by (DMPC)-(DCP) 9:1mol:mol have been prepared with deuterated water by thin-film technique followed by freeze-thaw and extrusion. (B12F12)—was specifically obtained with 12 fluorine atoms to detect fluorine diffusion signal with no contamination from bulk protons. Monodispersed microbeads with nominal diameter 10µm (mono), porosity =0.28±0.03 and poly-dispersed microbeads with nominal diameters 6,10,40,80,140µm (Poly), porosity=0.32±0.03 in water and Tween20 were prepared in NMR tubes. G50-Sephadex gel was prepared by mixing the dry powder in excess water at room temperature and allowed to swell overnight. Then gel was carefully poured into an NMR tube and settle under the influence of gravity. Sephadex is a network of chemically cross-linked dextrans, well known for its relatively uniform pore size. This gel is a structurally heterogeneous swollen polymer network that is commonly used for protein separation based on size. In the absence of a bulk hydrostatic pressure, there is no net motion in the gel-water system other than that due to water diffusion. The dry bead diameter ranges from 20 to 80µm. To investigate the pores and mesh of Sephadex, the compound was observed using a variable pressure scanning electron microscopy (VP-SEM, Hitachi SU-3500)) combined with Peltier cool stage control optimally allowed imaging of hydrated specimens under varying pressure conditions limiting water vapor loss through control of stage temperature. All diffusion measurements were performed on a Bruker 9.4T Avance (10 mm internal diameter bore) equipped with a gradient unit (maximum gradient-strength =1200mT/m). The temperature was fixed at 291K. A PGSTE with δ=4ms, by varying D in the range (10-1000) ms for each gradient strength g from 12 to 240mT/m along x and z axes, was used for collecting S(Δ) data to fit with the relation:𝑆(Δ)=𝐴∙𝑒xp(−Ka∙𝑞2∙Δ𝛼)+𝑐 (1)
for a parameter quantification. PGSTE by varying g at different diffusion times t=D was used to obtain D(t) behavior. Structural and geometric parameters together with S/V and tortuosity τ were obtained and compared. Simulations with fast random walk (FRW) algorithm7, able to adapt to different local confining structures, were used to obtain the MSD of different probes in the different matrices.
Results
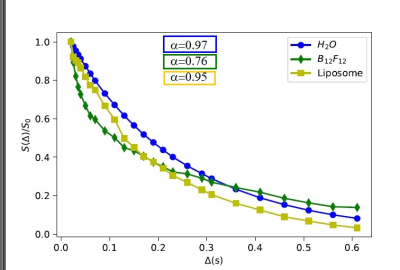
In Fig. 1, αvsg behavior in packed polystyrene-beads is reported. Poly-samples are characterized by a<1, while mono-samples show α close to 1. On the other hand, poly-sample shows a lower tortuosity compared to mono-sample in agreement with its porosity. Simulations confirm subdiffusion in poly-samples. In Fig.2 S(D) data points fit to Eq.1 is displayed for water B12F12-- and liposomes in G50-Sephadex. In Fig.3 characteristics length scales in G50-Sephadex are showed.Discussion
As Eq.1 held for tα<<1/Kaq2 , when g grows the relationship is valid for ever-smaller time intervals.Subdiffusion in poly-samples is due to multiple diffusion length-spaces.
Both water and liposomes in G50-Sephadex are characterized by a≈1, because their dimension does not match with the peculiar structural dimension of the Sephadex matrix. Conversely, diffusion of B12F12-- shows subdiffusion due to its dimension close to the mesh dimension of Sephadex-beads (Fig.3).
Conclusion
The comparison between samples geometrical characterization, simulations, conventional D(t) results and α estimated values highlights that subdiffusion is a potential powerful probe of submicroscopic organization.However, attention must be paid to the limits of validity of the tAD theory.
Acknowledgements
This project has received funding from the ATTRACT project funded by the EC under Grant Agreement 777222. Attract project title: Integrated Multimodal Optical and Magnetic Resonance Imaging (IMAGO)References
1. R. Metzler and J. Klafter, The random walk's guide to anomalous diffusion: a fractional dynamics approach. Phys. Rep. 339, 1 (2000).
2. M. Palombo, A. Gabrielli, S. De Santis, C. Cametti,G. Ruocco, S. Capuani, Spatio-temporal anomalous diffusion in heterogeneous media by nuclear magnetic resonance. 135, J. Chem. Phys. 034504 (2011).
3. M Palombo, A Gabrielli, VDP Servedio, G Ruocco, S Capuani, Structural disorder and anomalous diffusion in random packing of spheres. Sci Rep. 3, 2631 (2013)
4. Latour LL, Svoboda K, Mitra PP, Sotak CH. Time-dependent diffusion of water in a biological model system. Proc Natl Acad Sci USA. 91(4):1229 (1994)
5. V.G. Kiselev, Fundamentals of diffusion MRI physics. Nmr Biomed 30(3) (2017).
6. Novikov DS, Jensen JH, Helpern JA, Fiere-mans E. Revealing mesoscopic structural universality with diffusion. Proc Natl Acad Sci USA.111(14):5088 (2014)
7. Grebenkov DS A fast random walk algorithm for computing the pulsed-gradient spin-echo signal in multiscale porous media. J Magn reson 2, 208, 243--255 (2011)
Figures
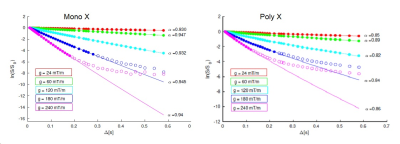
α parameter as a function of the magnetic gradient strength g along the x-direction obtained in polydispersed (Poly) and monodispersed (mono) packed polystyrene beads.
In mono-sample the diameter of the beads is 10 μm, in poly-sample, the beads diameters are: 6, 10, 40, 80, 140 μm.
The graph shows that as the gradient strength increases it is necessary to decrease the time range considered to perform the data fit to the function shown in Eq.1.