4055
Simulation of Focused RF Heating using a High-Channel-Count RF Array and a Maximum SAR Algorithm
Koray Ertan1,2, Joshua De Bever1, Mihir Pendse1, Paolo Decuzzi2, and Brian Rutt1
1Department of Radiology, Stanford University, Stanford, CA, United States, 2Italian Institute of Technology, Genoa, Italy
1Department of Radiology, Stanford University, Stanford, CA, United States, 2Italian Institute of Technology, Genoa, Italy
Synopsis
A 70-channel parallel transmit RF coil array intended for focused RF applications was designed and simulated at four frequencies (298 MHz, 447 MHz, 900 MHz and 1200 MHz) using a Ella body model. A previously proposed maxSAR algorithm was used to focus SAR in target regions while limiting the SAR in background tissues. Spatial maps of optimized SAR distribution are shown for several target locations and all frequencies. SAR distributions together with temperature and CEM43 maps suggest that clinical levels of focused hyperthermia can be achieved using high channel count parallel transmit RF coils and the maxSAR algorithm.
Introduction
Ultra-high field MRI scanners commonly use an array of parallel transmit RF coils to mitigate B1+ inhomogeneity while limiting absorbed power (SAR). Parallel transmit coils can also be used to intentionally generate targeted hyperthermia [1-3], in which one aims to generate maximum SAR inside a target region while constraining SAR in background tissues. In this work, we investigated the use of a novel maximum SAR algorithm for producing focused RF hyperthermia in human brain using a parallel transmit array operating at four different frequencies (298, 447, 900, 1200 MHz). The first two frequencies correspond to Larmor frequencies at 7T and 10.5T; therefore, the arrays in these two cases could serve as both MRI coil and focused RF applicator. Sharp RF focusing requires high channel count; therefore, these first simulations used a 70-channel loop array. We used our maxSAR algorithm [4-5] to compute the optimal channel weights for SAR focusing, and then performed thermal simulations incorporating bioheat/perfusion features, to evaluate several metrics of RF focusing: qualitative spatial localization, peak and mean SAR, temperature and thermal dose (defined by CEM43) in several target (central vs peripheral) and background regions.Methods
A 70-channel loop array was designed to fit the Ella body model [6] (Figure 1). EM design, modeling and thermal simulations were performed using Sim4Life (SPEAG, Zurich) at four frequencies (298, 447, 900, 1200MHz).$$\min_{\bf{b}}\max_{r=1,2...R}(\bf{b^HQ^{background}_{r}b})\quad [\textrm{1}]\\\textrm{such that}\max_{\textrm{p=1,2...P}}(\bf{b^HQ^{target}_{r}b})\geq \textrm{1}$$The maxSAR algorithm [4-5] was used to minimize the peak background SAR for constrained peak SAR inside the target region, as shown in Equation [1]: b is the complex RF channel weights, R and P are the number of background and target voxels respectively.$$$\bf{Q_{r}^{background}}$$$ and $$$\bf{Q_{r}^{target}}$$$ are the 10g averaged SAR matrices for background and target voxels respectively. Another region called the transition region surrounding the target region was introduced, within which SAR values were not included in the optimization. Our previously described vectorized oracle formalism [8] enabled highly efficient GPU-accelerated computation of SAR matrices. Calculation of SAR and sub-gradients at 92544 voxels for 70 channels takes less than 15 ms; this allowed us to perform an entire optimization in less than 4 minutes. Although amplifier power constraints can be easily embedded in the optimization problem, we only monitored but did not include amplifier power in the optimization; this was because the unconstrained peak amplifier power levels (<60W) were deemed low enough for practical implementation. Optimization was solved using ‘fmincon’ in MATLAB 2016. Optimum channel weights were then scaled to obtain a maximum peak local SAR of 20 W/kg in the background; and the resulting maximum and mean SAR values in the target region were then calculated. Optimized SAR distributions were used as heat source terms in a Pennes’ bioheat model that included perfusion and specific metabolic heat generation rates without dynamic thermoregulation effects.
Results
Figure 2 shows 10g-SAR, temperature and CEM43 maps resulting from maxSAR optimization for central and peripheral target regions. For the same target regions, quantitative SAR values as well as time evolution of temperature and CEM43 values are shown in Figure 3. Results suggest that 900 MHz has advantages over the other simulated frequencies, generating the highest maximum target SAR (~100W/kg), temperatures (~45oC) and CEM43 (~100min) values. In most cases, 10 minutes of RF application was found to be long enough to reach steady state temperature in target tissues; therefore, we report remaining thermal simulation results at 10 minutes of RF application. Figure 4 shows 10g-SAR, temperature and CEM43 maps for 900MHz and 8 different target locations. Location 7 is intentionally included as a challenging case in which the target region is near the periphery and the transition region ends directly below the skin. In all cases, high degrees of SAR, temperature and CEM43 focusing are achieved in the target region with focal spot diameters of approximately 1.6 cm in all directions, although performance varies significantly across different target locations. In figure 5, results are compared for all simulated frequencies at each target location. In general, 900 MHz and 1200 MHz show superior performance over lower simulated frequencies, consistent with the findings of Guerin et al [3]. Overall, our results show that target temperature ranges of 41-45 oC and target CEM43 ranges of 0.2-16 min can be achieved with only 10 minutes of RF exposure.Discussion
Using a maxSAR algorithm and a 70-channel loop array, very high target SAR values (~100W/kg) with peak background SAR no higher than 20W/kg are possible. Figure 5 suggests that this RF focusing performance should be enough for clinical hyperthermia applications, especially for targeted blood brain barrier permeability modulation [9]. Our computationally efficient vectorized SAR oracle allows sophisticated optimization methods to be designed and tested quickly and will allow us to more completely span the parameter space, for example to investigate the effect of frequency, number of transmit channels, body model variations, antenna configuration and types such as dipole, loop-dipole combination, bowtie.Conclusion
Ultra-high field, high channel count parallel transmit RF coils can be used together with a maxSAR algorithm to generate focal therapeutic hyperthermia in human brain.Acknowledgements
No acknowledgement found.References
[1] Winter, Lukas, et al. "Design and evaluation of a hybrid radiofrequency applicator for magnetic resonance imaging and RF induced hyperthermia: electromagnetic field simulations up to 14.0 Tesla and proof-of-concept at 7.0 Tesla." PloS one 8.4 (2013): e61661. [2] Winter Lukas, et al. “Ultrahighfield, One for all: Ultrawideband (279-500MHz) self-grounded bow-tie antenna for MR, and thermal.” ISMRM-ESMRMB 26th Joint Annual Meeting; 2018:#4281. [3] Guérin, Bastien, et al. "Computation of ultimate SAR amplification factors for radiofrequency hyperthermia in non-uniform body models: impact of frequency and tumour location." International Journal of Hyperthermia 34.1 (2018): 87-100. [4] Pendse, M., and B. Rutt. "An algorithm for maximum-SAR targeted RF hyperthermia." Proc Intl Soc Mag Reson Med. 2015. Abstract No: 3224 [5] Pendse, M.., and B. Rutt. "Method and apparatus for sar focusing with an array of rf transmitters." U.S. Patent Application No. 14/711,484. [6] Gosselin, Marie-Christine, et al. "Development of a new generation of high-resolution anatomical models for medical device evaluation: the Virtual Population 3.0." Physics in Medicine & Biology 59.18 (2014): 5287. [7] Kozlov, Mikhail, and Robert Turner. "Fast MRI coil analysis based on 3-D electromagnetic and RF circuit co-simulation." Journal of magnetic resonance 200.1 (2009): 147-152. [8] Pendse, M., and B. Rutt. "A Vectorized Formalism for Efficient SAR Computation in Parallel Transmission." Proc Intl Soc Mag Reson Med. 2015. Abstract No: 0379.[9] Yarmolenko, Pavel S., et al. "Thresholds for thermal damage to normal tissues: an update." International Journal of Hyperthermia 27.4 (2011): 320-343.Figures
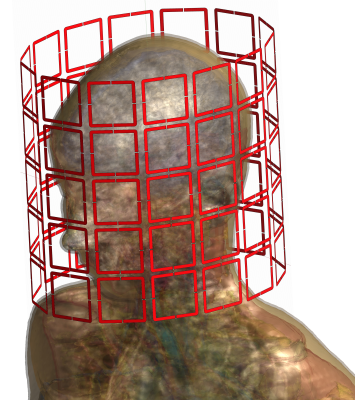
Figure 1: Simulated 70-channel loop array with 5 rows and
14 columns together with Ella body model (RF shield is omitted in the figure
due to visual concerns). Each element is a square with 5 cm dimensions. Both
coils and shield are modeled as a perfect electric conductor. ADS (Keysight
Technologies, CA) co-simulation software was used to find the optimal tuning
and matching component values [7].

Figure 2: Maximum intensity projections of optimized
(left) 10g-SAR
distribution, resulting (middle) temperature and (right) CEM43 maps for 298, 447, 900 and 1200 MHz.
Temperature and CEM43 results are provided for 10 minutes of RF application. (Top) Four rows show the results for
target volume located near the center of the brain and (bottom) four rows show the
results for target volume located at the periphery of the brain. Throughout the
study, the target region was defined by a sphere of 2 cm radius while a
surrounding sphere of 4 cm radius was used to bound the transition region.
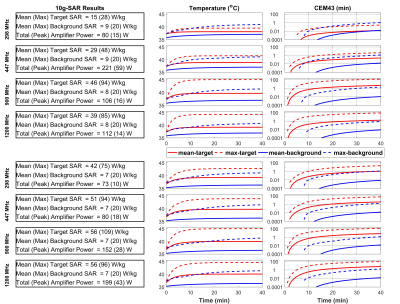
Figure 3: Quantitative results are shown for the
target locations and simulated frequencies in Figure 2. (left) shows mean and maximum 10g-SAR
values in target and background tissue. Additionally, required peak amplifier
power and total amplifier power are provided. (middle) and (right) shows mean and maximum values of resulting temperature and
CEM43 values as a function of time respectively. (Note: Vertical axes of CEM43
results are in logarithmic scale.)
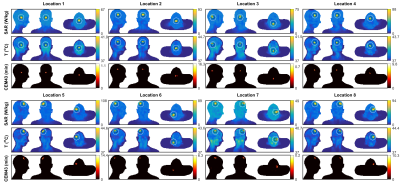
Figure 4: maxSAR optimization results at 900 MHz are
provided for 8 different target locations. For each target location, optimized
10g-SAR results as well as temperature and CEM43 distributions after 10 minutes
of RF application are shown. Variability in results across different target
locations can be observed. (Target region is bounded by black contour (a sphere
of 2 cm radius) and transition region (a sphere of 4 cm radius) is bounded by
white contour)
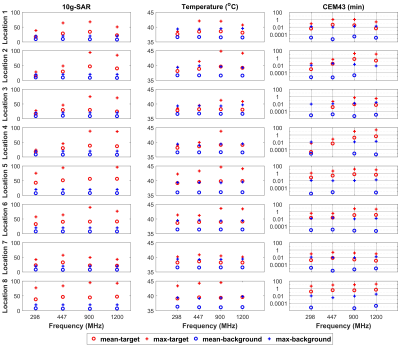
Figure 5: Evaluation of maxSAR optimization
performance for 298, 447, 900 and 1200 MHz. Maximum and mean
10g-SAR values in target and background tissues for (left) 10g-SAR, (middle) temperature and (right) final CEM43 values
after 10 minutes of RF application are plotted for each frequency. Each row
shows the results for different target locations which are marked and named in
Figure 4. (Note1: Vertical axes of CEM43 results are plotted in logarithmic
scale.) (Note2: Frequency axes is not uniformly scaled for space
considerations)