1274

An optimized multi-coil shim setup matching inhomogeneity distribution in the human brain; positive and negative aspects
Ali Aghaeifar1, Jiazheng Zhou1, Feng Jia2, Maxim Zaitsev2, and Klaus Scheffler1
1Max Planck Institute for Biological Cybernetics, Tuebingen, Germany, 2Dept. of Radiology, Medical Physics, Medical Center University of Freiburg, Faculty of Medicine, University of Freiburg, Freiburg, Germany

Synopsis

Multi-coil shim setup is a popular choice for B0 shimming. In contrast to conventional regular arrangement of the shim coils, one can effectively position the shim coil to match inhomogeneity distribution in the human brain. In this work, a comparison between regular and optimized arrangement of the local coils in a multi-coil shim setup is performed and the pros and cons of each design are evaluated.

Introduction

Expected gain in the B0-dependent parameters (e.g., SNR and contrast) increases the applications of ultra-high field MRI. However, inhomogeneity of the static field generated in the boundaries of components with different susceptibly (e.g., air and tissue) also scales with the field strength and requires additional tactic to counteract it. Dynamic slice-wise shimming can significantly improve field homogeneity1,2; however, it is not applicable for 3D sequences and significant gain cannot be achieved for multi-band imaging (MB factor ≥ 3)3. Employing additional shim coils can help; but, it is not a practical solution because of the need for more current amplifiers, larger space, and challenges of troubleshooting and maintenance. Recent work shows that a constrained nonlinear optimization can be performed to yield an effective arrangement of the local shim coils in order to improve shimming of the human brain without increasing number of the coils4. This work evaluates and compares such an optimized multi-coil with a regular arrangement of the local shim coils from various perspectives.

Method

Arrangement of the local shim coils in multi-coils with a different number of the coils (8, 16, 24, 32, 48, and 65 coils) was optimized using nonlinear constrained optimization in a cylindrical coordinate system. Three degrees of freedom for each coil were considered in optimization including size of the coil and angular and axial coordinates of the coil on the cylinder surface. Regular and the corresponding optimized design of multi-coils with same number of the coils were investigated and compared from several aspects. Constrained (1A to 5A) and unconstrained shimming were performed on whole brain B0 maps of 19 volunteers acquired at 9.4T with an average volume of 1383 ± 121 mL (mean ± SD). The calculated currents were averaged over volunteers to determine the number of coils with a high contribution.

Results and Discussion

Figure 1 shows the arrangement of the local coils before (regular design) and after optimization for multi-coils with a different number of the coils. Distribution of the coils after optimization represents a high density of the coils above the frontal and temporal lobe while a few coils are positioned below the occipital lobe.
Figure 2 displays plots of root-mean-square (RMS) level of inhomogeneity after shimming with multi-coils with different numbers of the coils for both regular and optimized designs. Several important points can be derived from Figure 2. First, the difference of constrained shimming (1A) between regular and the corresponding optimized multi-coil is larger for multi-coils with fewer channels. However, the relative improvement is similar for all (i.e., optimized design performed better than the regular one by ~13%). Second, the comparison between performance of constrained and unconstrained shimming reveals a major difference between two designs. The constrained and unconstrained shimming with the regular arrangement of coils (number of the coils below 32) yield similar results while performance of the optimized design highly depends on current constraint. Although unconstrained shimming is not practically feasible; however, acheiving the performance of the constraint of 5A which is close to unconstrained shimming (number of the coils below 32) is possible by increasing number of coils windings. Third, field homogeneity obtained with constrained shimming with optimized designs is even higher than unconstrained shimming with regular design for multi-coils with less than 48 channels (which are practical setups).
Figure 3 shows the average required current per channel after an unconstraint shimming. Number of channels that do not need high current (their contribution to the shimming is not limited by current) is higher for the regular design in comparison to the optimized design. The load of shimming is balanced among many coils, and more coils are engaged in shimming process for the case of optimized multi-coils while number of underactive coils is considerably high in regular multi-coils.
Because of coil overlapping in the optimized design, one has to put the coils into the several layers which can degrade the shimming performance. This issue is also true for the shim coil design based on the stream function method and singular value decomposition5. For the obtained optimized multi-coils in this work, the maximum number of the coils that can be kept in the first layer (original position) without overlapping is 7, 11, 13, 15, and 17 for multi-coils with 8, 16, 24, 32, and 48 channels. The rest of the coils must be moved to layer 2 or higher. The coils overlapping can also be controlled by including additional regularization term in the optimization process.
The optimized arrangement of the coils matches pattern of inhomogneity; however, that is not suitable arrangement for combined imaging and shimming6 or imaging acceleration7. One may note that such arrangement, because of the coils accumulation in some areas, is prone to be too warm in some local spots which may necessitate a cooling pathway to be embedded into the coil (figure 4).

Acknowledgements

No acknowledgement found.

References

  1. Juchem C, Umesh Rudrapatna S, Nixon TW, de Graaf RA. Dynamic multi-coil technique (DYNAMITE) shimming for echo-planar imaging of the human brain at 7 Tesla. Neuroimage 2015;105:462–472.
  2. Aghaeifar A, Mirkes C, Bause J, Steffen T, Avdievitch N, Henning A, Scheffler K. Dynamic B 0 shimming of the human brain at 9.4 T with a 16-channel multi-coil shim setup. Magn Reson Med 2018;80:1714–1725.
  3. Stockmann JP, Wald LL. In vivo B 0 field shimming methods for MRI at 7 T. Neuroimage 2018;168:71–87.
  4. Aghaeifar A, Zhou J, Heule R, Tabibian B, Schölkopf B, Jia F, Zaitsev M, Scheffler K. A 32‐channel multi‐coil setup optimized for human brain shimming at 9.4T. Magn Reson Med 2020;83:749–764.
  5. Jia F, Elshatlawy H, Aghaeifar A, et al. Design of a shim coil array matched to the human brain anatomy. Magn Reson Med 2019. doi: 10.1002/mrm.28016.
  6. Umesh Rudrapatna S, Fluerenbrock F, Nixon TW, de Graaf RA, Juchem C. Combined imaging and shimming with the dynamic multi‐coil technique. Magn Reson Med 2019;81:1424–1433.
  7. Scheffler K, Loktyushin A, Bause J, Aghaeifar A, Steffen T, Schölkopf B. Spread‐spectrum magnetic resonance imaging. Magn Reson Med 2019;82:877–885.

Figures

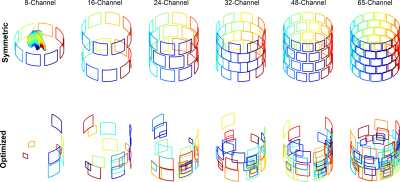
Figure 1: Arrangement of the local shim coils in multi-coils with a different number of the coils before (regular arrangement) and after position and size optimization with nonlinear constrained optimization. All coils are positioned on s cylinder with a diameter of 323 mm. The side length of the square coils in regular design is 100, 100, 100, 80, 70, and 70 mm for multi-coils with 8, 16, 24, 32, 48, and 65 channels, respectively. Details of the optimization process are reported in reference 4.

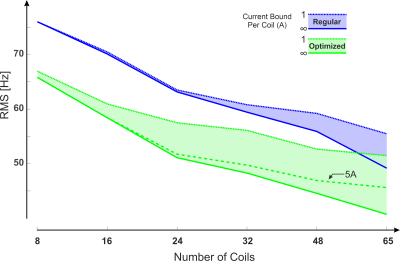
Figure 2: Performance of constrained and unconstrained shimming with regular and optimized multi-coils with a different number of the channels. Constrained shimming with the optimized multi-coil design generally performs better than unconstrained shimming with the corresponding regular multi-coil design.

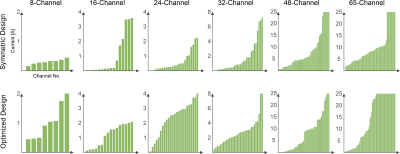
Figure 3: The average of the currents which were calculated for shimming of whole-brain of 19 volunteers. Shimming was performed without any bound (unconstrained) to allow the coils for the best performance. The number of coils that do not contribute significantly in shimming with regular design is more than optimized design. One may remove some coils in regular design without losing the performance substantially.

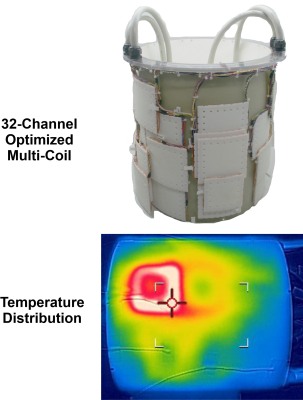
Figure 4: Temperature map of 32-channel optimized multi-coil reported in reference 4 after passing 1.5A into all channels for an hour. Heating is pretty local in the optimized design because of coils density on the top of the coil.

Proc. Intl. Soc. Mag. Reson. Med. 28 (2020)
1274