1160
Imaging of skeletal muscle contraction using Oscillating Gradient Spin Echo (OGSE)1Department of Radiology, Stanford University, Stanford, CA, United States
Synopsis
The apparent diffusion coefficient measured using DTI in skeletal muscles depends on the time allowed for diffusing water molecules to probe the local environment and on the size of muscle cells. Here we explore the use of Oscillating Gradient Spin Echo diffusion to obtain information on skeletal muscle microstructure over smaller distances than conventionally probed using PGSE. Our results show the ability to image skeletal muscle during active contraction (foot dorsiflexion and plantarflexion) using OGSE, and diffusion values are dependent on the oscillation frequency and on the contraction status of the muscle
Introduction
Diffusion Tensor Imaging (DTI) is becoming an increasing popular tool to assess skeletal muscle status in a wide range of diseases (1–3) and muscle injuries (4). Furthermore, DTI has been shown to be sensitive to transient changes such as passive shortening and lengthening (5, 6) with increased radial diffusivity associated with passive shortening and increase in the fascicle cross-sectional area.The apparent diffusion coefficient (ADC) measured using DTI depends on the time allowed for diffusing water molecules to probe the local environment (the so called “Diffusion Time”). For increasing diffusion times, the molecules will interact with more barriers and the ADC will decrease, eventually reaching an asymptotic lower value. Therefore, it is possible to study different level of tissue organization by tuning the diffusion time accordingly.
DTI in skeletal muscle is typically performed using Pulsed Gradient Spin-Echo (PGSE) diffusion, which has an inherently long mixing time (about 30 ms), resulting in an inability to provide information at the level of the individual muscle fibers, typically on the order of few micrometers. In practice, PGSE is also very sensitive to bulk motion such as muscle nervous stimulation that lead to unwanted signal dropout.
Oscillating Gradient Spin-Echo (OGSE) has been proposed as an efficient method to probe diffusion of water molecules over smaller distances while maintaining a sufficient amount of diffusion encoding (7, 8). By design, OGSE provides motion compensation, which is particularly useful to study contracted muscles.
The aim of this work is to explore OGSE for evaluation of human skeletal muscle on a clinical 3T scanner and exploit its inherent motion compensation to measure muscle microstructure during active muscle contraction.
Methods
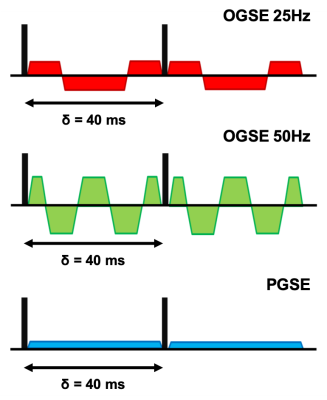
An OGSE sequence with cosine trapezoidal gradients (9) was implement on a 3T scanner (SIGNA 750w Premier, gradient strength = 80 mT/m, max slew rate = 120 mT/m/ms, GE Healtcare) using the KS Foundation framework.Two different OGSE frequencies were used: 25Hz and 50Hz, corresponding to N = 1 and N = 2 oscillations respectively (Fig 1). For both OGSE frequencies the encoding waveform duration before and after the refocusing pulse was 40 ms long. For comparison, a PGSE sequence with the same gradient duration and b-value was also used. The max gradient strength was adjusted in order to achieve the same b-value of 180 s/mm2 for all three acquisitions.
For all three scans (OGSE_25Hz. OGSE_50Hz and PGSE) diffusion was encoded along 15 non-collinear diffusion encoding directions. Other common scan parameters were TR/TE = 2800/94 ms, FOV = 160*160 mm2, 10 slices, voxel size = 2.7 x 2.7 x 10 mm3. The total scan time for each sequence was 1 min 35s.
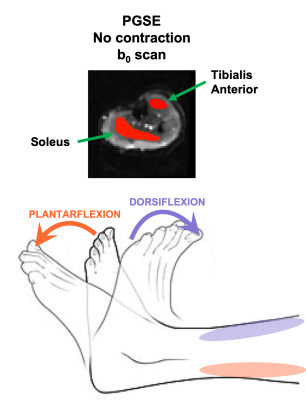
The left lower leg of five (n = 5) healthy volunteers was scanned in three positions: relaxed (no contraction), active dorsiflexion and active plantarflexion (Fig 2). For comparison, an isotropic phantom was also scanned with the same protocol.
All datasets were denoised using a local PCA-based algorithm and then fitted to a tensor model. Diffusion parameters including axial diffusivity and radial diffusivity were extracted from the tensor model (10).
Results
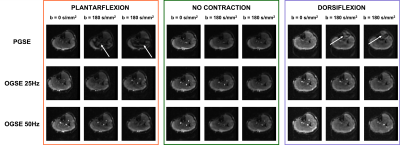
In the isotropic phantom no difference in ADC was measured between OGSE and PGSE acquisitions. As expected in free diffusion no dependency of diffusivity on the OGSE frequency was observed (data not shown).PGSE scans acquired during muscle contraction showed areas of signal voids localized in the anterior compartment (for dorsiflexion), and in the posterior compartment (plantarflexion) (Fig 3). These areas of signal dephasing present during contraction in PGSE scans result in areas of abnormally high diffusivity (Fig 4).
On the other hand, diffusion values during contraction in OGSE show a trend of decreasing diffusivities for decreasing frequencies, indicating that for longer diffusion times water molecules interact more and more with their environment.
Time-dependent diffusion was observed in the soleus muscle (Fig 4 and 5), with decreasing diffusivity at decreasing frequency, as well as differences in diffusion for different contractions. For all the scans performed without contraction, the PGSE approach gave significantly higher values of diffusivity compared to OGSE (Fig 4f and 5). This is likely caused by the residual perfusion effects measurable at small b-values (180 mm2/s in our case).
Discussion and conclusions
Our results show that PGSE diffusion waveforms are not suitable for assessing skeletal muscle during active contraction. The cosine OGSE waveform on the other hand, offers motion compensation and therefore is robust towards signal dephasing induced by muscle contraction (11).In general, the implementation of cosine-trapezoid waveforms on clinical scanner is limited by the achievable gradient strength (6.5 mT/m in our case), and sufficient diffusion encoding comes at the expense of lengthening the TE. Nonetheless, our preliminary result show that OGSE is feasible in skeletal muscle musculature and could allow for a better depiction of muscle microstructure during active contraction.
Further work will focus on eliminating contributions from microcirculation and perfusion (12).
In conclusion, we observed time dependent diffusion in contracting skeletal muscles and measured differences in the same muscles when relaxed or contracted. This technique holds potential to non-invasively measure changes in muscle size during contraction and for assessment of contraction abnormalities in patients with muscle diseases.
Acknowledgements
This work was supported by Rubicon NWO Grant 452182304. We receive research support from GE Healthcare.References
1. Oudeman J, Nederveen AJ, Strijkers GJ, Maas M, Luijten PR, Froeling M: Techniques and applications of skeletal muscle diffusion tensor imaging: A review. J Magn Reson Imaging 2016; 43:773–788.
2. Damon BM, Froeling M, Buck AKW, et al.: Skeletal muscle diffusion tensor-MRI fiber tracking: rationale, data acquisition and analysis methods, applications and future directions. NMR Biomed 2017; 3:e3563.
3. Hooijmans MT, Damon BM, Froeling M, et al.: Evaluation of skeletal muscle DTI in patients with duchenne muscular dystrophy. NMR Biomed 2015; 28:1589–1597.
4. Froeling M, Oudeman J, Strijkers GJ, et al.: Muscle Changes Detected with Diffusion-Tensor Imaging after Long-Distance Running. Radiology 2015; 274:548–562.
5. Mazzoli V, Oudeman J, Nicolay K, et al.: Assessment of passive muscle elongation using Diffusion Tensor MRI: Correlation between fiber length and diffusion coefficients. NMR Biomed 2016(September):1–12.
6. Schwenzer NF, Steidle G, Martirosian P, et al.: Diffusion tensor imaging of the human calf muscle: Distinct changes in fractional anisotropy and mean diffusion due to passive muscle shortening and stretching. NMR Biomed 2009; 22:1047–1053.
7. Baron CA, Beaulieu C: Oscillating gradient spin-echo (OGSE) diffusion tensor imaging of the human brain. Magn Reson Med 2014; 72:726–736.
8. Parsons EC, Does MD, Gore JC: Temporal diffusion spectroscopy: Theory and implementation in restricted systems using oscillating gradients. Magn Reson Med 2006; 55:75–84.
9. Van AT, Holdsworth SJ, Bammer R: In vivo investigation of restricted diffusion in the human brain with optimized oscillating diffusion gradient encoding. Magn Reson Med 2014; 71:83–94.
10. Garyfallidis E, Brett M, Amirbekian B, et al.: Dipy, a library for the analysis of diffusion MRI data. Front Neuroinform 2014; 8(FEB):1–17.
11. Whittaker RG, Porcari P, Braz L, Williams TL, Schofield IS, Blamire AM: Functional magnetic resonance imaging of human motor unit fasciculation in amyotrophic lateral sclerosis. Ann Neurol 2019; 85:455–459.
12. Wu D, Zhang J: The Effect of Microcirculatory Flow on Oscillating Gradient Diffusion MRI and Diffusion Encoding with Dual-Frequency Orthogonal Gradients (DEFOG). Magn Reson Med 2017; 77:1583–1592.
Figures
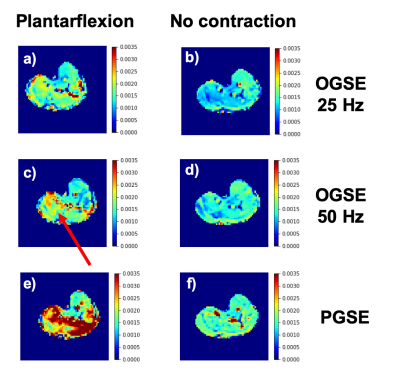
Radial diffusivity in one healthy volunteer. When muscles are relaxed (b and d) the diffusion decreases with decreasing OGSE frequency, indicating more restriction effects as water molecules travel for longer diffusion times.
When the subject performs active foot plantarlexion, the muscles in the posterior compartment of the leg (indicated by the arrow) contract and the cross-sectional area of muscle cells is increased. This results in an increase in diffusivity (a and c).
These changes in diffusivity for different OGSE frequencies could be exploited to derive cellular size.
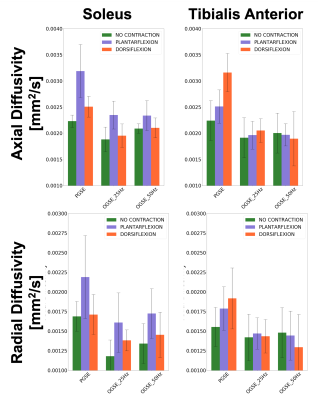
Time dependent diffusion is observed in the direction of muscle fibers (Axial Diffusivity) as well as in the cross section (Radial DIffusivity) and is dependent on muscle contraction status in the soleus. Less clear pattern of time-dependence seen in tibias anterior, probably reflecting the different ratio of fiber type I vs fiber type II in the two muscle groups.
Significantly higher diffusion values are measured with PGSE compared to OGSE, due to signal dephasing from muscle twitch and increased perfusion contribution when the muscle is activated.