4993
Multi-channel phase combination for quantitative susceptibility mapping at 7T using Phase-Offsets Estimation from Multi-echoes (POEM) method1University of Calgary, Calgary, AB, Canada, 2University of Melbourne, Melbourne, Australia
Synopsis
A Phase-Offsets Estimation from Multi-echoes (POEM) method is proposed to combined the phase-arrayed coil at 7T for quantitative susceptibility mapping (QSM). The method demonstrates equivalent or better results than adding a conventional reference scan for both single-orientation and multi-orientation QSM.
Introduction
Complex combination of multi-channel receiver signals is not trivial at ultra-high field due to the lack of a uniform body coil. One method requires an ultra-short TE reference scan (COMPOSER) [1], which adds scan time. Another approach requires multi-echoes with specific $$$TEs$$$ (ASPIRE) [2], which limits the sequence parameters. Here we propose a new technique that performs the Phase-Offsets Estimation from Multi-echoes (POEM) with arbitrary $$$TEs$$$ and without need of a reference scan.Methods
Theory: The measured phase from individual receiver channels has three main components. One from total field induced phase that accumulates with $$$TE$$$, one from an initial receiver phase-offset that remains constant, and one from eddy currents originated from the readout gradients that is assumed to be the same for the same readout polarity (positive or negative) [3]:$$\theta_{I} = -2 \pi \gamma \Delta B_{0} TE + \theta_{off,I} + \theta_{edd,I}.$$ Both the receiver $$$\theta_{off,I}$$$ and eddy currents phase-offsets $$$\theta_{edd,I}$$$ can be removed using the first two consecutive echoes of the same polarity:$$\Delta \theta_{I} = \angle S_{2,I} / S_{1,I} = -2 \pi \gamma \Delta B_{0} \Delta TE.$$ The phase difference maps $$$\Delta \theta_{I}$$$ are combined (complex summation) and unwrapped (best-path method [4]), producing a combined phase $$$\Delta \theta$$$ with effective echo time of $$$\Delta TE$$$, free of receiver and eddy currents phase-offsets. The theoretical phase without phase-offsets at $$$TE_{1}$$$ is then calculated as $$$\theta_{TE_{1}} = \Delta \theta \cdot TE_{1}/\Delta TE = -2 \pi \gamma \Delta B_{0} TE_{1}$$$. Phase-offset of individual channel $$$I$$$ is computed: $$\theta_{off,I} + \theta_{edd,I} = \theta_{TE_{1},I} - \theta_{TE_{1}}.$$
Data acquisition: (1) Seven subjects (32.4 ± 5.2) were scanned on a 7T whole-body research scanner (Siemens Healthcare, Erlangen, Germany) using a 3D unipolar 4-echo-GRE sequence: $$$TE_{1}$$$ = 4.8ms, $$$\Delta TE$$$ = 3.6ms, $$$TR$$$ = 18 ms, voxel size = 0.6mm isotropic, scan time = 8:52min.
(2) One subject was also scanned in another 4 head orientations (left, right, extension, flexion) using the same sequence to enable COSMOS reconstruction.
(3) Another subject was also scanned using a 3D bipolar 9-echo-GRE sequence: $$$TE_{1}$$$ = 5.1ms, $$$\Delta TE$$$ = 2ms, $$$TR$$$ = 24ms, voxel size = 0.75mm isotropic, scan time = 8:42min.
(4) An ultra-short TE radial acquisition was added for each subject to directly measure the receiver phase-offsets using COMPOSER: $$$TE$$$ = 0.07ms, $$$TR$$$ = 1.99 ms, voxel size = 1mm isotropic, scan time = 1:56 min. For all acquisitions, a 32-channel head coil (Nova Medical, Wilmington MA, USA) was used for signal reception.
Image processing: Phase-offsets were estimated and smoothed using a Gaussian filter with a sigma of 4mm. The COMPOSER reference scan phase was registered to the GRE scan using FLIRT. Estimated phase-offsets using either POEM or COMPOSER were removed and multi-channel images were combined using complex summation. Combined phase images were unwrapped using best-path method [4] and then fitted across echo times to generate a total field map. Background field was removed using the RESHARP method [5] with a kernel radius of 2mm and Tikhonov regularization of 10-4. QSM was performed using iLSQR method [6].
Results
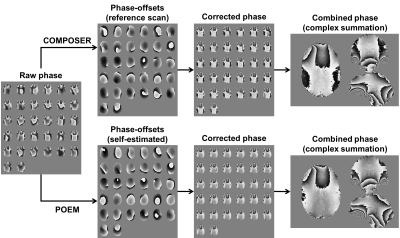
Figure 1: phase-offsets of the 32 channels from POEM look similar but different to COMPOSER reference scan, which may due to the removal of eddy currents induced phase-offsets in POEM. Combined phase images show significantly improved SNR and no singularities for both methods.
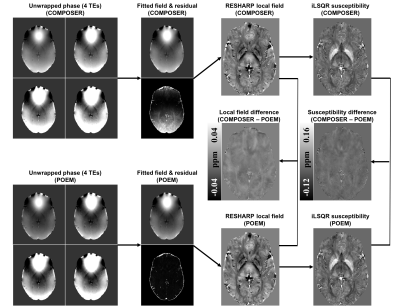
Figure2: combined phase images from POEM lead to smaller fitting residuals across $$$TEs$$$ than the COMPOSER method. Local field and susceptibility maps look similar for both methods, with no noticeable contrast in the difference maps.
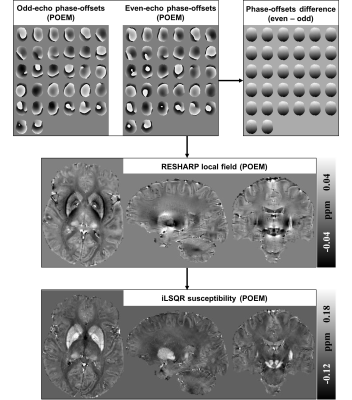
Figure 3: for bipolar GRE acquisition, estimated odd and even phase-offsets from POEM look slightly different. The offsets difference appears linear in the readout direction [7,8], which may due to eddy currents induced phase-offsets [3].
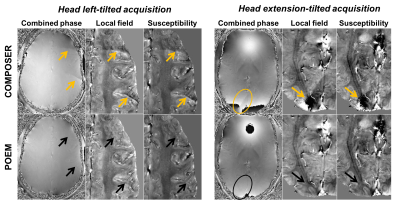
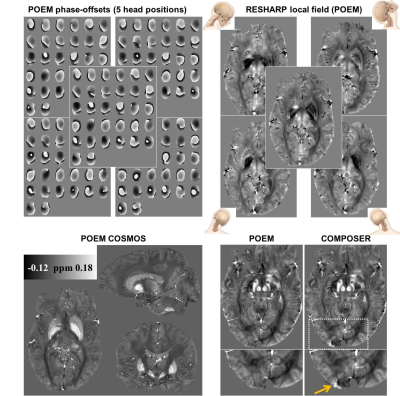
Figure 4: when using the COMPOSER reference scan from a neutral head position to correct for titled head positions (left and extension as shown), open-ended fringe lines and singularities result in substantial artifacts in local field and susceptibility maps. POEM method does not show artifacts at the same locations.
Figure 5: POEM successfully combines phase channels regardless of head orientations, generating successful COSMOS with superior susceptibility contrast and SNR. While COSMOS reconstructed from COMPOSER phase images show artifact.
Conclusion
The proposed POEM method can estimate phase-offsets from both receiver coil and eddy currents sources, resulting in robust phase combination for QSM. It produces similar local field and susceptibly maps as compared to adding a reference scan using COMPOSER. Moreover, POEM shows significant advantages when the actual scan is acquired in a different head position than the reference scan. This is valuable whenever there is a significant head displacement between scans due to motion or intentional head orientation shifts for COSMOS.Acknowledgements
Alberta Innovates: Health Solutions
RHISE HBI-Melbourne Trainee Research Exchange
University of Melbourne McKenzie fellowship
Canadian Institutesof Health Research
Campus Alberta Innovates Program
NHMRC Peter Doherty Fellowship
National Imaging Facility (Australian government NCRIS program)
High-performance computing support provided by the Multi-modal Australian ScienceS Imaging and Visualisation Environment (MASSIVE)
References
[1] Robinson SD, Dymerska B, Bogner W, Barth M, Zaric O, Goluch S, Grabner G, Deligianni X, Bieri O, Trattnig S. Combining phase images from array coils using a short echo time reference scan (COMPOSER). Magn Reson Med. 2017 Jan;77(1):318-327.
[2] Eckstein K, Dymerska B, Bachrata B, Bogner W, Poljanc K, Trattnig S, Robinson SD. Computationally Efficient Combination of Multi-channel Phase Data From Multi-echo Acquisitions (ASPIRE). Magn Reson Med. 2017 Oct 16. doi: 10.1002/mrm.26963.
[3] Feng W, Neelavalli J, Haacke EM. Catalytic multiecho phase unwrapping scheme (CAMPUS) in multiecho gradient echo imaging: removing phase wraps on a voxel-by-voxel basis. Magn Reson Med. 2013 Jul;70(1):117-26.
[4] Abdul-Rahman HS, Gdeisat MA, Burton DR, Lalor MJ, Lilley F, Moore CJ. Fast and robust three-dimensional best path phase unwrapping algorithm. Appl Opt. 2007 Sep 10;46(26):6623-35.
[5] Sun H, Wilman AH. Background field removal using spherical mean value filtering and Tikhonov regularization. Magn Reson Med. 2014 Mar;71(3):1151-7.
[6] Li W, Wang N, Yu F, Han H, Cao W, Romero R, Tantiwongkosi B, Duong TQ, Liu C. A method for estimating and removing streaking artifacts in quantitative susceptibility mapping. Neuroimage. 2015 Mar;108:111-22.
[7] Li J, Chang S, Liu T, Jiang H, Dong F, Pei M, Wang Q, Wang Y. Phase-corrected bipolar gradients in multi-echo gradient-echo sequences for quantitative susceptibility mapping. MAGMA. 2015 Aug;28(4):347-55.
[8] Sun H, MacDonald ME, Pike GB; Phase Correction of a Bipolar Gradient-Echo Acquisition for Quantitative Susceptibility Mapping; ISMRM; Singapore; 2016.
Figures
