4082
Slice-wise motion tracking during simultaneous EEG-fMRI1Danish Research Centre for Magnetic Resonance Imaging, Centre for Functional and Diagnostic Imaging and Research, Copenhagen University Hospital Hvidovre, Copenhagen, Denmark, 2Center for Magnetic Resonance, DTU Elektro, Technical University of Denmark, Lyngby, Denmark, 3Philips, Copenhagen, Denmark, 4Institute of Physics, Martin-Luther-Universität Halle-Wittenberg, Halle (Saale), Germany, 5Beijing Institute for Brain Disorders, Beijing, China, 6State Key Laboratory of Brain and Cognitive Science, Beijing MR Center for Brain Research, Institute of Biophysics, Chinese Academy of Sciences, Beijing, China, 7University of Chinese Academy of Sciences, Beijing, China, 8DTU Compute, Technical University of Denmark, Lyngby, Denmark
Synopsis
Slice-wise motion tracking during combined electroencephalography (EEG) and echo planar imaging (EPI) is developed. Using gradient-induced noise on the EEG for tracking, no interleaved navigator modules or additional hardware is needed. The motion parameters are determined after a calibration and training scan. The method is explored in a phantom and in vivo.
Intruduction
Simultaneous EEG-fMRI is widely used in cognitive neuroscience. Noise from gradient switching is problematic, especially in combination with motion. The noise can, however, provide accurate motion tracking [1-4] as a by-product of normal EEG-fMRI. This method was hitherto evaluated for tracking per volume. Using a high bandwidth EEG system, we explore brain motion estimation from individual slice acquisitions.Methods
During fMRI, EEG records motion-correlated voltages induced by gradient switching. Faraday’s law describes these for each EEG-channel $$$i$$$:
$$V_{i}(t)=w_{ix}\frac{\text{d}\tilde{G_{x}}}{\text{d}t}+w_{iy}\frac{\text{d}\tilde{G_{y}}}{\text{d}t}+w_{iz}\frac{\text{d}\tilde{G_{z}}}{\text{d}t},$$
where $$$\frac{\text{d}\tilde{G_{x}}}{\text{d}t},\frac{\text{d}\tilde{G_{y}}}{\text{d}t},\frac{\text{d}\tilde{G_{z}}}{\text{d}t}$$$ are altered versions of $$$\frac{\text{d}G_{x}}{\text{d}t},\frac{\text{d}G_{y}}{\text{d}t},\frac{\text{d}G_{z}}{\text{d}t}$$$ due to filtering of the EEG-system and uncompensated eddy currents. The weights $$$w_{ix},w_{iy},w_{iz}$$$, depend on position, geometry and orientation of the wire loop and therefore reflect the position and orientation of the subject. Assuming small changes in subject position and rigid body head movement, a linear approximation is valid:
$$\triangle w=A\triangle r_{h},$$
where $$$\triangle w$$$ is a 3Nx1 vector (N EEG-channels) with changes in weights relative to a reference position, $$$\triangle r_{h}$$$ is a 6 element vector representing relative head position with six degrees of freedom $$$(x,y,z,\theta,\phi,\psi)$$$. $$$A$$$ is a 3Nx6 matrix with partial derivatives of the weights with respect to image-derived head position parameters relative to the reference position. The matrix can be estimated from a training scan, where the subject is moving step-wise so that normal image-based motion tracking is possible. For partial validation, image-derived positions are correlated with gradient activity measured by EEG. A phantom attached to a 2m pole controlled by an experimenter was used to simulate subject motion. It consisted of an EEG-capped styrofoam head with three small and one big water sphere embedded for imaging. Measurements were performed using a MR-compatible EEG-cap (Easycap, Germany) with interconnected electrodes (carbon wired) of which 10 channels were used (80kHz sampling rate, highpass-filtering at 64 Hz to isolate gradient switching, NeurOne Tesla, Bittium Biosignals Ltd, Finland, & 3T Achieva MRI, Philips, Best,The Netherlands). An echo planar imaging (EPI) multi-slice sequence was used with TE/TR=30/7000ms (including 4000ms breaks for movement), 3mm isotropic resolution, tip angle 80°. During a static one-off preparation scan, the sequence was run repeatedly with different pairs of gradients deactivated for determining $$$\frac{\text{d}\tilde{G_{x}}}{\text{d}t}, \frac{\text{d}\tilde{G_{y}}}{\text{d}t}, \frac{\text{d}\tilde{G_{z}}}{\text{d}t}$$$. Then an EPI time series of 75 volumes was collected (50 volumes with motion between volume acquisitions, and 25 with free motion). The first 25 volumes were used for training, the next 25 for estimation of precision, and the last for tracking of free motion. EEG motion-tracking was compared to motion estimated from the EPI data using SPM12 volume realignment (UCL, UK). Similar volunteer data was acquired with a normal EEG-fMRI setup and parameters as above. We used 11 channels of the EEG (40 kHz sampling rate, highpass-filtering at 32Hz). A similar time series (TE/TR=30/4000ms) of 100 volumes was acquired. To test the abilities of the setup, the subject performed random stepwise motion between volume acquisitions, allowing comparison of motion estimated from EEG and EPI. The model was trained on the first 25 dynamics and tested on the remaining 75.
Results
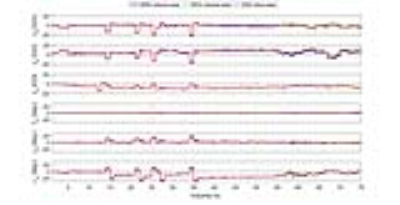
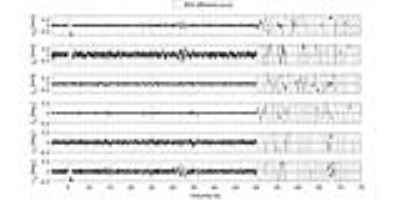
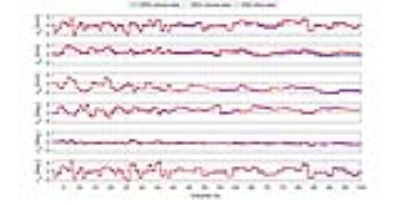
Fig. 1 shows estimated motion parameters using SPM12 volume realignment and EEG. The curves for volume-wise EEG and SPM tracking are virtually identical within the range of motion experienced during training. During free motion, the curves show expected smoothness of motion parameters while volume-wise tracking appears to provide insufficient motion compensation. Fig. 2 shows the differences between volume-wise and slice-wise tracking for step-wise motion (standard deviations $$$[x, y, z]$$$=[0.032, 0.087, 0.034] mm, $$$[\theta, \phi, \psi] $$$= [0.01407, 0.03648, 0.03589] degrees). Fig. 3 shows motion tracking for the human subject using the same methods. A high similarity between motion parameters derived from images and from EEG, is seen. The differences between slice-wise and volume-wise EEG tracking are low (standard deviations $$$[x, y, z]$$$=[0.099, 0.12, 0.082] mm, $$$[\theta, \phi, \psi] $$$= [0.075, 0.037, 0.081] degrees).Discussion & Conclusion
Volume-wise estimation of stepwise motion using EEG closely resembles motion estimates derived from SPM-realignment, thus validating the EEG model. The correspondence between slice-wise and volume-wise EEG estimates of step-wise motion confirm that slice-acquisition retain sufficient information to accurately track motion. For free motion, slice-wise detection using EEG gives expected smooth parameter estimates, contrary to volume realignment. Deviations between SPM and EEG-models occur due to model non-linearities for severe motion, and image distortions from $$$B_{0}$$$-field inhomogeneities mostly affecting y-translation. The accuracy is reduced in vivo, but can potentially reach the phantom level, if some of the EEG electrodes are interconnected with resistors to form wire loops similar to those of the phantom, i.e. loops unaffected by changes in skin conductance.Acknowledgements
Malte Laustsen is supported by the Sino-Danish Center for Education and Research.References
[1] M. B. Vestergaard, J. Schulz, R. Turner and L. G. Hanson, "Motion tracking from gradient induced signals in electrode recordings", ESMRMB Congress, 2011.
[2] M. Andersen, K. Madsen and L. G. Hanson, "Prospective motion correction for MRI using EEG-equipment", ISMRM 24th Conference, 2016.
[3] E. H. Bhuiyan, G. S. Spencer, P. M. Glover and R. Bowtell, "Tracking head movement inside an MR scanner using voltages induced in coils by time-varying gradients", ISMRM 25th Conference, 2017.
[4] M. Laustsen, M. Andersen, K. H. Madsen and L. G. Hanson, "Gradient distortions in EEG provide motion tracking during simultaneous EEG-fMRI", ISMRM Workshop on: Motion Correction in MRI & MRS, 2017.
Figures
