4058
Adaptive SAR mass-averaging to predict RF heating for B1 shimming in the presence of a hip implant for parallel transmit at 3T1School of Information Technology and Electrical Engineering, The University of Queensland, Brisbane, Australia, 2Siemens Healthcare Pty Ltd, Brisbane, Australia, 3Centre for Advanced Imaging, The University of Queensland, Brisbane, Australia
Synopsis
Parallel-transmit and B1-shimming techniques may significantly reduce radiofrequency (RF) artefacts when imaging near metal implants at 3T; however, it is known that around metal implants the 10g-averaged specific-absorption-rate (SAR10g) locations do not coincide with the location of maximum heating. In this work, we investigate the behaviour of the B1 field and RF heating around metal implants in a generic body coil at 3T as a function of shimming parameters using the adaptive SAR method we previously introduced as a fast estimate of temperature increase.
Purpose
Parallel transmit (pTx) and B1-shimming techniques can substantially improve radiofrequency (RF) homogeneity when imaging near metal implants at 3T1. However, specific B1 shim settings can focus the electric field and cause localized temperature increases2,3. Current practise uses the 10g-averaged specific absorption rate (SAR10g)4,5, which relies on electromagnetic simulations of human models to limit RF power, but it has been shown that the correlation of SAR10g with temperature cannot be guaranteed near hip prostheses. For this reason, an adaptive mass-averaging SAR6 (adSAR) was previously introduced to capture the temperature increases in tissues near a hip prosthesis at 7T. In this work, we investigate whether adSAR can be used as a fast prediction parameter for RF heating around metal implants in a generic 2-channel body coil at 3T, and use adSAR to predict heating while improving RF homogeneity around implants.Methods
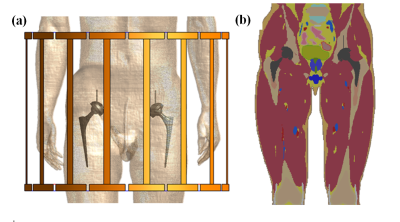
The realistic body model Duke v3.1, from the virtual family7, was modified to simulate a patient with bilateral CoCrMo hip implants. Simulations were performed in Sim4Life (ZMT, Zurich, Switzerland) with a generic 16-rung, shielded, high-pass birdcage coil8 (Fig.1). Each channel was successively driven by a 1V voltage source.
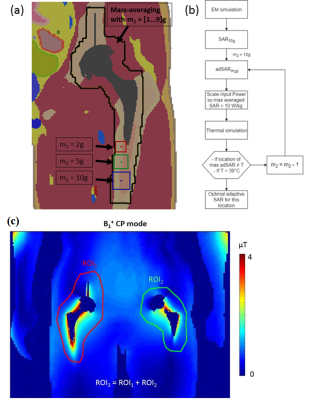
adSAR is designed to monitor RF heating by limiting the input power to ensure that the temperature will remain below 39˚C6, but requires an optimization of the averaging mass near the metal implant. For this purpose, ten shims were selected, that cause SAR10g ≥ 90% of the global maximum in tissues near the metal implants. In tissues where at least 1% of the 10g-averaging volume overlapped with one implant (region within black perimeter in Fig.2a), SAR was averaged over masses m=[1 .. 9]g to give the adSAR. For each of those 10 scenarios, input powers were calculated that lead to a 10W/kg adSAR for all the averaging masses and a 2W/kg whole-body SAR (wbSAR). Thermal simulations were performed to determine the corresponding averaging mass m that limits the temperature increase to below 39˚C applying a continuous RF signal for 30 minutes (temperature distribution was allowed to reach a steady state for 40 minutes before the pulse; temperature dependent blood perfusion9 in all tissues except constant perfusion in bones) (Fig.2b).
After
the optimization of adSAR, various shims were used to calculate a
homogeneity map1 (standard deviation in a region of interest (ROI)) and
RF heating map (peak-adSAR),
using adSAR as monitoring parameter. Shims
with the ratio of amplitudes ranging from R = 0.02 to 45, and the transmit
phase difference ranging from ΔΦ
= -180˚ to 180˚ were used
in three different regions of interest (ROI) (Fig.2c), and fields were normalized
to 1W input power.
Results
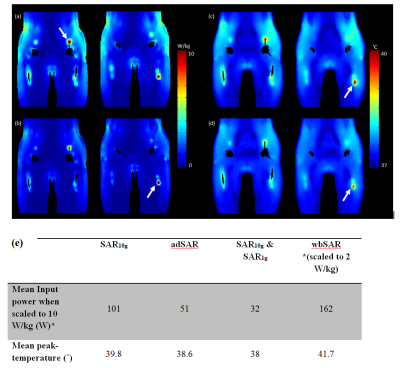
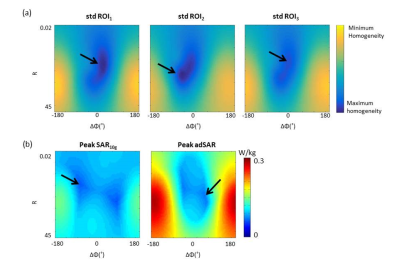
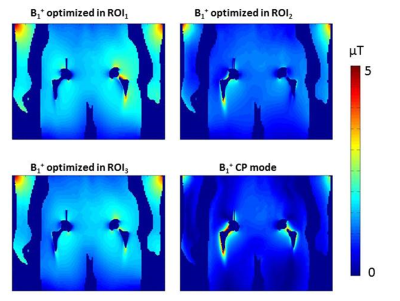
SAR10g failed to predict the location of the peak temperature and failed to keep the temperature below 39˚C. Fig.3a&3b shows a case where SAR10g and adSAR predicted different locations of the peak temperature. Optimal adSAR-averaging-masses of 3g and 5g at the tip of the screw and stem, respectively, were found to correlate with temperature distribution. Fig.3c&3d show that adSAR significantly improved the correlation between SAR and temperature profiles, and the temperature was reduced from 40 to 38.7˚C. wbSAR allowed significantly higher input powers, but resulted in temperatures up to 43.3˚C. Alternatively using SAR1g was overly restrictive in terms of the input power, as summarized in Fig.3e. Fig.4 shows the RF homogeneity and heating maps, with SAR10g and adSAR. These maps show that shims with R and ΔΦ close to 1 and 0˚, respectively, result in more homogeneous B1+ in the ROIs and lower adSAR. Figure 5 shows optimized B1+ in the ROIs compared to the CP mode. The standard deviation was improved by 63%, 51% and 48% in ROI1, ROI2 and ROI3 respectively, and would therefore significantly reduce RF artefacts.Discussion and Conclusion
Shimming in the presence of metal implants is challenging as it significantly changes the electromagnetic field and temperature distribution. Because thermal simulations are not linear when thermoregulation is considered, individual simulations are required for each shim or input power10, which is not feasible in a clinical environment. adSAR can be used as a proxy for running extensive thermal simulations by essentially ensuring that the steady-state temperature remains below 39˚C. As it can easily be scaled with input power and adapted to new shims11, it can be used on scanners for a fast prediction of RF heating. In combination with a RF homogeneity map, optimal shimming can be performed in tissues near hip prostheses. As such, our proposed method has the potential to substantially improve RF homogeneity whilst efficiently preventing RF heating in the vicinity of hip implants.Acknowledgements
Aurelien Destruel acknowledges Siemens Healthcare Pty Ltd for providing funding for a PhD scholarship and ZMT for providing the free academic license of the software Sim4Life. Markus Barth acknowledges funding from ARC Future Fellowship grant FT140100865.References
1. Bachschmidt TJ, Kohler M, Nistler J et al. Polarized multichannel transmit MRI to reduce shading near metal implants. Magn Reson Med 2015.
2. Murbach M, Zastrow E, Neufeld E et al. Heating and Safety Concerns of the Radio-Frequency Field in MRI. Current Radiology Reports 2015;3(12):1-9.
3. ASTM. Standard F2182-11a, 2011, standard test method for measurement of radio frequency induced heating on or near passive implants during magnetic resonance imaging. West Conshohocken, PA: ASTM International 2011.
4. International Electrotechnical Commission, Medical electrical equipment, Part 2: particular requirements for the basic safety and essential performance of magnetic resonance equipment for medical diagnosis. IEC 60601-2-33 2010.
5. Powell J, Papadaki A, Hand J et al. Numerical simulation of SAR induced around Co-Cr-Mo hip prostheses in situ exposed to RF fields associated with 1.5 and 3 T MRI body coils. Magn Reson Med 2012;68(3):960-968.
6. Destruel A, O'Brien K, Barth M et al. Adaptive SAR mass-averaging compared against thermal simulations in the presence of a titanium hip prosthesis in 7T pTx MRI. 2017; Hawaii, HI, USA.
7. Gosselin MC, Neufeld E, Moser H et al. Development of a new generation of high-resolution anatomical models for medical device evaluation: the Virtual Population 3.0. Physics in Medicine and Biology 2014;59(18):5287-5303.
8. Luo W, Lanagan MT, Sica CT et al. Permittivity and performance of dielectric pads with sintered ceramic beads in MRI: early experiments and simulations at 3 T. Magn Reson Med 2013;70(1):269-275.
9. Murbach M, Neufeld E, Samaras T et al. Pregnant women models analyzed for RF exposure and temperature increase in 3T RF shimmed birdcages. Magn Reson Med 2016.
10. Neufeld E, Fuetterer M, Murbach M et al. Rapid method for thermal dose-based safety supervision during MR scans. Bioelectromagnetics 2015
11. Eichfelder G, Gebhardt M. Local Specific Absorption Rate Control for Parallel Transmission by Virtual Observation Points. Magnetic Resonance in Medicine 2011;66(5):1468-1476.
Figures