3397

Optimal control based design of parallel transmission RF pulses with minimum local SAR

Armin Rund¹, Christoph Stefan Aigner², Lena Nohava³, Roberta Frass-Kriegl³, Elmar Laistler³, Karl Kunisch^1,4, and Rudolf Stollberger²

¹Institute for Mathematics and Scientific Computing, University of Graz, Graz, Austria, ²Institute of Medical Engineering, Graz University of Technology, Graz, Austria, ³Division MR Physics, Center for Medical Physics and Biomedical Engineering, Medical University of Vienna, Vienna, Austria, ⁴Johann Radon Institute for Computational and Applied Mathematics (RICAM), Austrian Academy of Sciences, Linz, Austria

Synopsis

An optimal control framework for designing parallel transmission RF pulses and gradient shapes is introduced. The optimal control model includes technical constraints and a local SAR model based on the Q-matrix formalism. Second-order optimization methods give RF pulses with enhanced homogeneity of the excitation pattern and/or decreased local SAR. The optimized results are tested in numerical experiments and validated with numerical electromagnetic simulations.

Purpose

The inclusion of B₁⁺ maps in the RF pulse design process for parallel transmit (pTx^1,2) allows increasing the homogeneity of multi-dimensional excitation patterns^3,4. Below, an optimal control method is introduced for the joint design of multi-dimensional RF, and gradient shapes with hard physical constraints for pTx with minimum local 10g-SAR using the Q-matrix formalism⁵.

Theory

Joint design of RF and gradient amplitudes for fixed pulse duration is posed as an optimal control model for the spin-domain Bloch equation with Cayley-Klein parameters ($$$\alpha,\beta$$$). The slice profile accuracy for a 2D excitation is posed as inequality constraints (in magnitude and phase). We control eight complex-valued RF pulses $$$U_k(t)$$$ and two gradient components $$$(g_1(t),g_2(t))$$$ $$B_1=\sum_{k=1}^8 S_k(x)U_k(t), \qquad G_s=g_1(t) x+g_2(t)y$$ using the RF coil sensitivities $$$S_k(x)$$$ of the RF channels. $$$(x,y)$$$ are the spatial coordinates. The task is to minimize the time averaged maximum local 10g SAR modeled by the Q-matrix⁵. Componentwise amplitude constraints on RF and gradients are included by semismooth Newton techniques. The more general bounds on the slew rate and the slice profile accuracy are treated with a $$$L^p$$$ penalization⁶. The optimization is done with second-order methods using exact adjoint-based first derivatives and quasi-Newton approximations for the second derivatives (BFGS) together with a trust-region framework for robustness⁶.

Methods

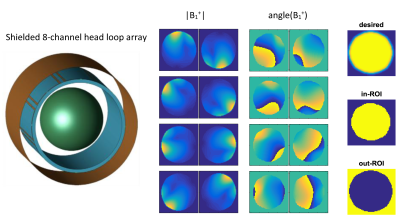
The proposed algorithm is implemented in MATLAB (The MathWorks, Inc, Natick, USA) and applied to the design of pulses for a homogeneous two-dimensional 90° slice excitation using a generic shielded 8-channel pTx loop head coil⁵ (Figure 1). We used electromagnetic simulations (XFdtd 7.4, Remcom, State College, USA) and circuit co-simulation (ADS, Keysight Technologies, Santa Clara, USA) to compute the complex B₁⁺ maps in of a homogeneous spherical phantom (d = 18cm, ε = 50.6, σ = 0.66S/m). The field of view was set to 220x220mm with a 64x64 matrix resulting in 4096 spatial points. The circular 2-dimensional target pattern (ROI) was defined in the transverse plane in the center of the sphere with a diameter of 180mm, with in-ROI and out-ROI regions (Fig. 1, right). As the initialization of the optimization, we used the spatial domain method^3,4 with excitation flip angle 90° (>83° in-ROI, <5° out-ROI), temporal discretization of 4µs, and allowed error of 10% with a pulse duration of 12.4ms. For optimization, the complex RF pulses were bound by a peak power of 1kW per channel and both gradient channels limited to a peak amplitude of 40mT/m and a slew rate of 212T/m/s. For “low SAR”-optimization, the profile accuracy was constrained by the mean squared error of the initialization (in-ROI magnitude and phase, out-ROI magnitude). A factor six smaller in-ROI magnitude error was used for “low error”-optimization.

Results and Discussion

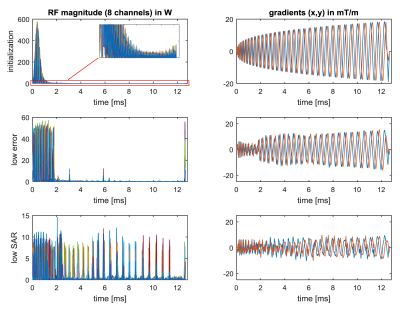
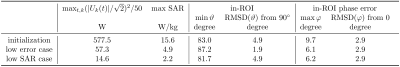
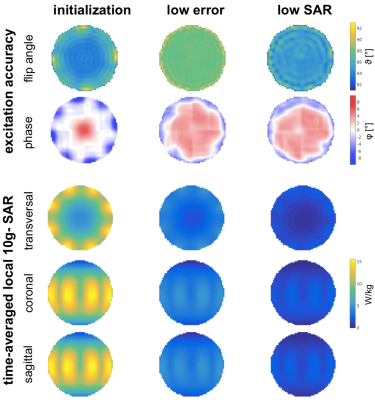
Figure 2 shows the RF and gradient shapes of the initialization and after optimization. The initial spiral-out gradient trajectory results in skewed power deposition at the center of k-space, thus leading to high peak RF power. Both optimized results show significantly reduced peak RF and a more uniform RF power deposition over time. Figure 3 compares the peak RF power and SAR of the investigated pulses. The “low error” optimum yields a higher profile accuracy in-ROI, both in the root mean square deviation (RMSD) and in the infinity-norm (higher minimum flip angle) and decreases peak RF power by 90%, and SAR by 68%. The “low SAR” optimum holds the in-ROI RMSD of the initialization. The in-ROI minimum flip angle is decreased by 1.6% which allows the optimization method to reduce peak RF power by 97% and maximum SAR by 86%. Both optima exhibit the same in-ROI phase RMSD as the initialization, but with smaller maximum deviation. Figure 4 shows the results of the Bloch and SAR simulations. The first row shows the flip angle, the second row contains the phase of the transversal magnetization ($$$2\alpha \beta^*$$$). Excitation profiles are well defined and phase dispersion is slightly lower as compared to the initial pulse. Rows 3-5 contain SAR maximum intensity projections in all three directions. Notably, SAR values decrease globally without significant changes in their spatial distribution.

Conclusion

Bloch and SAR simulations indicate the feasibility of using the proposed optimal control based design method to jointly compute complex RF pulses and gradient shapes for large flip angle pTx excitation and its ability to reduce local SAR and peak RF power.

Acknowledgements

Partly supported by Austrian Science Fund (FWF) projects SFB F3209-18 (MOBIS) and P28059-N36 (pULSE), and by BioTechMed-Graz.

References

1) Katscher U, Börnert P, Leussler C, and van den Brink JS. Transmit SENSE. Magn. Reson. Med., 49 (2003) 144-50.

2) Zhu Y. Parallel excitation with an array of transmit coils. Magn. Reson. Med., 51 (2004) 775-84.

3) Grissom W, Yip CY, Zhang Z, Stenger VA, Fessler JA, and Noll DC. Spatial domain method for the design of RF pulses in multicoil parallel excitation. Magn. Reson. Med., 56 (2006) 620–629.

4) Grissom W, Xu D, Kerr AB, Fessler JA, and Noll DC. Fast Large-Tip-Angle Multidimensional and Parallel RF Pulse Design in MRI. IEEE Trans. Med. Imaging, 28 (2009) 1548-1559.

5) Kuehne A, Goluch S, Waxmann P, Seifert F, Ittermann B, Moser E, and Laistler E. Power balance and loss mechanism analysis in RF transmit coil arrays. Magn. Reson. Med., 74 (2015), 1165–1176.

6) Rund A, Aigner CS, Kunisch K, and Stollberger R. Magnetic Resonance RF pulse design by optimal control with physical constraints. IEEE Trans. Med. Imaging, early access (02.10.2017).

Figures

Figure 1: Simulated 8 Tx-channel head coil for 7T 1H MRI loaded with a spherical phantom⁵ (left), magnitude and phase maps of each coil channel’s B₁⁺ (center), and the desired excitation pattern (right).

Figure 2: Comparison of RF magnitude and gradient shapes of the initialization and the two optimized cases. Note the different scaling on the y-axis for the RF pulse magnitude.

Figure 3: Peak RF power and time-averaged maximum local 10g-SAR; minimum flip angle θ, maximum phase φ, as well as their root mean squared deviation (RMSD).

Figure 4: Simulated 2-dimensional in-ROI excitation profiles as flip angle and phase distributions, and corresponding maximum intensity projections of the time-averaged local 10g-SAR in three directions compared for the initial pulse and the two optimized pulses.

Proc. Intl. Soc. Mag. Reson. Med. 26 (2018)

3397