Synopsis

In this work, we use an objective approach to optimize sequential and time-encoded multi-PLD protocols, and compare them to the recommended single-PLD protocol using simulations, with the aim of determining which method is capable of producing the most accurate CBF estimates across a range of ATTs.

Introduction

There are multiple pseudo-continuous Arterial Spin Labeling (PCASL)¹ methods for measuring cerebral blood flow (CBF), the main distinction being whether images are acquired at one or multiple post-labeling delays (PLDs). Acquiring multiple PLDs means that CBF can be more accurately estimated by also estimating the arterial transit time (ATT), whereas a single PLD must estimate CBF with a simplified model: a potential source of error². However, many averages may be acquired in a given scan time for single-PLD, improving the SNR at that PLD.

The multi-PLD methods are further split by whether PLDs are acquired sequentially³, each with a separate tag and control pair, or time-encoded (TE)⁴, where the PCASL pulse train is alternated between label and control according to a Hadamard encoding scheme. TE results in greater signal averaging and its benefits have been demonstrated against a matched sequential protocol⁵. However, the effective PLDs are dictated by the label duration (LD) of each TE block, which limits the method’s flexibility and ability to take advantage of long LDs and the resulting perfusion signal accumulation that PCASL can provide. These protocol differences make it extremely challenging to determine which can achieve the most accurate CBF estimates.

It has recently been demonstrated that the PLDs in sequential multi-PLD PCASL can be optimized to minimize CBF estimation variance, resulting in a ~40% decrease of CBF error in vivo, compared to a standard protocol⁶. In this work, we use this objective approach to optimize the LDs and PLDs for sequential and TE multi-PLD protocols, and compare these to the recommended single-PLD protocol⁷ with simulations, with the aim of determining which method is capable of producing the most accurate CBF estimates across a range of ATTs.

Methods

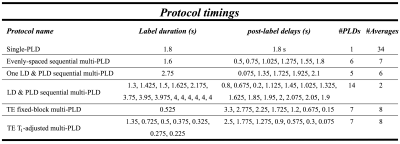
All methods were optimized for CBF measurements, using the Cramér-Rao Lower Bound (CRLB) on the parameter covariance matrix, across an expected healthy ATT range of 500 – 1800ms, and a fixed scan time of 5 minutes⁶. Sequential multi-PLD was optimized for: 1. one fixed LD with multiple PLDs and 2. multiple LD and PLD combinations, and then compared to a reference protocol with LD=1.6s, 5 evenly-distributed PLDs=0.5-1.8s. TE fixed block⁴ (TE-fixed) and the T1-adjusted variant⁸ (TE-T1adj) were optimized for total LD and final PLD. Parameters are given in Table 1.

Monte Carlo (MC) simulations were used to validate the predicted CBF and ATT variance from the optimization, across the same ATT range. Realistic noise was added to simulated data (Table 1) and fit using a variational Bayes approach⁹. The root-mean-squared-error relative to the ground truth values were used to compare protocols.

Results

The optimized protocols are shown in Table 2. The sequential protocol with multiple LD and PLD combinations was chosen for further comparison with other methods, since it had the lowest CBF errors of the sequential multi-PLD protocols (marginal improvement over the one-LD/multi-PLD protocol, results not shown).

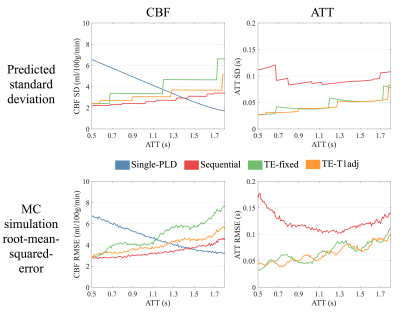
Theoretical and simulated CBF and ATT estimation errors are shown in Figure 1 as a function of ATT, showing that sequential multi-PLD is able to achieve the lowest CBF errors across almost the entire ATT range, outperforming both single-delay and time-encoded methods.

Although not explicitly included in the optimization, the time-encoded methods produced more accurate ATT estimates due to their better sampling of the inflow of labeled blood. TE T1-adj produced more accurate CBF and ATT measurements than TE fixed-block.

Discussion

We have demonstrated that, after protocol optimization, sequential multi-PLD PCASL is capable of producing more accurate CBF estimates than single-PLD or time-encoded methods across an ATT range commonly found in healthy volunteers.

TE, though more time-efficient than a matched sequential multi-PLD protocol⁵, is not as flexible with its choice of LDs and PLDs. Sequential protocols can use longer labeling durations than are available within each TE block, which outweighs the benefit gained through greater noise averaging. However, TE inherently samples the signal inflow more than the sequential multi-PLD protocols used here, resulting in more accurate ATT measurements. This agrees with previous comparison work¹⁰.

Despite the greater number of averages achievable, single-PLD only did better for CBF estimation than TE-fixed and does not provide information about blood arrival time and is very sensitive to delayed blood arrival. Some of the single-PLD CBF error at short ATTs is due to the assumed difference between T_1b and T_1t. However, when setting T_1b=T_1t, the predicted error for single-PLD in this ideal case is still larger than for sequential multi-PLD.

Future work will include a rigorous in vivo comparison to validate these results.

Acknowledgements

References

1. Dai W, Garcia D, De Bazelaire C, Alsop DC. Continuous flow-driven inversion for arterial spin labeling using pulsed radio frequency and gradient fields. Magn Reson Med 2008; 60: 1488–1497.
2. Buxton RB, Frank LR, Wong EC, Siewert B, Warach S, Edelman RR. A general kinetic model for quantitative perfusion imaging with arterial spin labeling. Magn Reson Med 1998; 40: 383–396.
3. Wang DJJ, Alger JR, Qiao JX, Gunther M, Pope WB, Saver JL et al. Multi-delay multi-parametric arterial spin-labeled perfusion MRI in acute ischemic stroke - Comparison with dynamic susceptibility contrast enhanced perfusion imaging. NeuroImage Clin 2013; 3: 1–7.
4. Günther M. Highly efficient accelerated acquisition of perfusion inflow series by Cycled Arterial Spin Labeling. Proc Intl Soc Mag Reson Med 2007; 15: 2007.
5. Dai W, Shankaranarayanan A, Alsop DC. Volumetric measurement of perfusion and arterial transit delay using hadamard encoded continuous arterial spin labeling. Magn Reson Med 2013; 69: 1014–1022.
6. Woods JG, Chappell MA, Okell TW. Optimizing Post Labeling Delays in Multiple-Delay Arterial Spin Labeling MRI for Cerebral Perfusion Imaging. Magn Reson Mater Phy 2017; 30: 233–234.
7. Alsop DC, Detre JA, Golay X, Günther M, Hendrikse J, Hernandez-Garcia L et al. Recommended implementation of arterial spin-labeled perfusion MRI for clinical applications: A consensus of the ISMRM perfusion study group and the European consortium for ASL in dementia. Magn Reson Med 2015; 73: 102–116.
8. Teeuwisse WM, Schmid S, Ghariq E, Veer IM, Van Osch MJP. Time-encoded pseudocontinuous arterial spin labeling: Basic properties and timing strategies for human applications. Magn Reson Med 2014; 72: 1712–1722.
9. Chappell MA, Groves AR, Whitcher B, Woolrich MW. Variational Bayesian Inference for a Nonlinear Forward Model. IEEE Trans Signal Process 2009; 57: 223–236.
10. Guo J, Holdsworth SJ, Fan AP, Lebel MR, Zun Z, Shankaranarayanan A et al. Comparing accuracy and reproducibility of sequential and Hadamard-encoded multidelay pseudocontinuous arterial spin labeling for measuring cerebral blood flow and arterial transit time in healthy subjects: A simulation and in vivo study. J Magn Reson Imaging 2017; : 1–14.