Synopsis

We extend diffusion-weighted acquisitions using the stimulated echo acquisition mode (STEAM) to imaginary signals. In classical diffusion encoding, only real signals arise; however, for special applications of double diffusion encoding (DDE), complex signals arise, as shown here for diffusion pore imaging, a method that allows determining the shape of arbitrary closed pores filled with an NMR-detectable medium. It is shown that the phase information of complex signals is preserved under application of stimulated echoes: We show the analytically derived signal for DDE with STEAM and use Monte Carlo simulations for validation. Consequently STEAM can be employed for diffusion pore imaging.

Introduction

Diffusion pore imaging enables the measurement of the average shape of arbitrary closed pores in an imaging volume element. Examples of such pores could in principle comprise cells in biological tissue or oil containing cavities in porous rocks. Measuring the Fourier transform of the pore space function is realized with non-conventional gradient profiles: Either by applying a long-narrow gradient profile^1-5 or by combination of q-space imaging with double diffusion encoded (DDE) measurements^6-8. Both methods enable measuring imaginary signals containing the pore shape information, which gets lost in classical diffusion encoding. While the first approach is limited in its sequence design, the second is highly flexible and allows incorporating stimulated echoes to reach the long-time limit exploiting the longer T1-relaxation time compared to T2. To the authors’ knowledge, only real signals have been acquired with stimulated echoes so far.

The present study presents the analytically derived signal for DDE-based pore imaging with stimulated echo acquisition mode (STEAM) demonstrating that the phase information is preserved for stimulated echoes. Validation using Monte Carlo simulations is presented and appropriate choice of RF-pulse phases is investigated.

Methods 1: Analytical derivation of STEAM signals

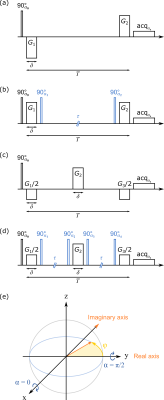
The signals arising for the sequences depicted in Fig. 1(a)-(d) are derived by sequentially applying building blocks for a) short gradient pulses and b) RF-pulse sequences generating stimulated echoes:

a) A gradient pulse $$$\boldsymbol{G}_i$$$ of duration $$$\delta$$$ induces a phase factor $$$e^{iφ_i}$$$ to spins resting at position $$${\boldsymbol{x}}_i$$$ during gradient application with $$$φ_i=-γδ{\boldsymbol{G}}_i{\boldsymbol{x}}_i$$$. If the pore’s center of mass $$$\boldsymbol{x}_{\text{cm}}$$$ is translated relative to the origin, additional phase shifts $$$ϑ$$$ occur.

b) If a pulse sequence $$$\left(90_{α_1}^°-τ-90_{α_2}^°\right)$$$ is applied to a transverse magnetization $$$e^{iφ}$$$ ($$$α_j$$$= phase of 90° pulse), the first pulse $$$90_{α_1}^°$$$ stores the real part as z-magnetization which is returned to the transversal plane by $$$90_{α_2}^°$$$ resulting in⁹ $$${\text{Re}}\left(-e^{i(φ-α_1)}\right)\,e^{iα_2}=-\frac{1}{2}\,\left(e^{i(φ-α_1+α_2)}+e^{i(-φ+α_1+α_2)}\right)$$$. Definitions of coordinate system and phases in Fig.$$$~$$$1(e).

For DDE with STEAM [Fig. 1(d)], the sequence $$90_{α_0}^°-{\boldsymbol{G}}_1/2-\left(90_{α_1}^°-τ-90_{α_2}^°\right)-{\boldsymbol{G}}_2-\left(90_{α_3}^°-τ-90_{α_4}^°\right)-{\boldsymbol{G}}_3/2-{\text{acq}}_{α_{\text{a}}}$$ gives $$\frac{1}{4}\,e^{i(α_0-α_1-α_2+α_3+α_4)}\,e^{i\left(\frac{φ_1}{2}-φ_2+\frac{φ_3}{2}\right)}+\frac{1}{4}\,e^{i(α_0-α_1+α_2-α_3+α_4)}\,e^{i\left(\frac{φ_1}{2}+φ_2+\frac{φ_3}{2}+2ϑ\right)}\\+\frac{1}{4}\,e^{i(-α_0+α_1-α_2+α_3+α_4)}\,e^{i\left(-\frac{φ_1}{2}-φ_2+\frac{φ_3}{2}-ϑ\right)}+\frac{1}{4}\,e^{i(-α_0+α_1+α_2-α_3+α_4)}\,e^{i\left(-\frac{φ_1}{2}+φ_2+\frac{φ_3}{2}+ϑ\right)},$$ with $$$ϑ=-γδ\boldsymbol{G}_2\boldsymbol{x}_{\text{cm}}$$$. The signal is calculated by averaging over all random walkers of all pores. $$$\delta\rightarrow0$$$ and diffusion time $$$T\rightarrow\infty$$$ allows averaging independently over each $$$\boldsymbol{x}_i$$$ with $$$e^{iφ_i}$$$ resulting in $$$\int_{Pore}\rho(\boldsymbol{x}_i )e^{-i\boldsymbol{qx}_i}d\boldsymbol{x}_i=\tilde{\rho}(\boldsymbol{q})$$$ with the pore space function $$$\rho(\boldsymbol{x})$$$, its Fourier transform $$$\tilde{\rho}(\boldsymbol{q})$$$ and q-space vector $$$\boldsymbol{q}=\gamma\boldsymbol{G}_2\delta$$$. Averaging over $$$\boldsymbol{x}_{\text{cm}}$$$ introduces the Fourier transform of the pore center of mass distribution $$$\tilde{P}_{\boldsymbol{x}_{\text{cm}}}(\boldsymbol{q})$$$ as prefactors (see results).

Methods 2: Monte Carlo simulations

Monte Carlo simulations were performed realizing RF-pulses with rotation matrices. Pores of equilateral triangular shape were chosen with 10$$$~$$$ µm edge length and 100,000 random walkers per pore.

Three 2D distributions of the pores centers of mass were simulated: 1) rock or tissue sample: 10,000 pores randomly distributed in 1x1 mm; 2) large unordered phantom: 1100 pores randomly distributed in 5x5$$$~$$$mm having dimensions comparable to the phantom studied in ¹⁰; 3) small grid phantom: 9 pores on a square grid, distances of 10$$$~$$$µm between centers of mass.

Additional parameters: diffusion coefficient 1$$$~$$$µm²/ms, $$$\delta$$$=2.88$$$~$$$ms, G=3.87$$$~$$$T/m, $$$T$$$=150$$$~$$$ms.

Results 1: STEAM signals

The STEAM DDE signal is composed of four terms:

$$S_{\text{DDE}}({\boldsymbol{q}})=\frac{1}{4}\,e^{i(α_0-α_1-α_2+α_3+α_4)}\,\tilde{\rho}^*({\boldsymbol{q}}/2)^2\tilde{\rho}({\boldsymbol{q}})+\frac{1}{4}\,e^{i(α_0-α_1+α_2-α_3+α_4)}\,\tilde{P}_{\boldsymbol{x}_{\text{cm}}}^*(2{\boldsymbol{q}})\,\tilde{\rho}^*({\boldsymbol{q}}/2)^2\tilde{\rho}^*({\boldsymbol{q}})\\+\frac{1}{4}\,e^{i(-α_0+α_1-α_2+α_3+α_4)}\,\tilde{P}_{\boldsymbol{x}_{\text{cm}}}({\boldsymbol{q}})\,|\tilde{\rho}({\boldsymbol{q}}/2)|^2\tilde{\rho}({\boldsymbol{q}})+\frac{1}{4}\,e^{i(-α_0+α_1+α_2-α_3+α_4)}\,\tilde{P}_{\boldsymbol{x}_{\text{cm}}}^*({\boldsymbol{q}})\,|\tilde{\rho}({\boldsymbol{q}}/2)|^2\tilde{\rho}^*({\boldsymbol{q}}).$$

Analogously for the STEAM q-space imaging signal:

$$S_{\text{QSI}}({\boldsymbol{q}})=-\frac{1}{2}\,e^{i(-α_0+α_1+α_2)}\,|\tilde{\rho}({\boldsymbol{q}})|^2-\frac{1}{2}\,e^{i(α_0-α_1+α_2)}\,\tilde{P}_{\boldsymbol{x}_{\text{cm}}}^*(2{\boldsymbol{q}})\,\tilde{\rho}^*({\boldsymbol{q}})^2.$$

The asterisk marks the complex conjugate. First terms correspond to the respective spin echo signals, corresponding to the desired pore imaging signal, reduced by a factor of 2 for each storage in the longitudinal direction, for $$$\alpha_{0,...,4}=0$$$. The additional undesired terms are modulated with the Fourier transform of the center of mass distribution $$$\tilde{P}_{\boldsymbol{x}_{\text{cm}}}(\boldsymbol{q})$$$.

Results 2: Validation with simulations

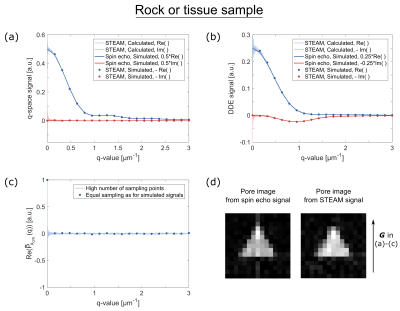
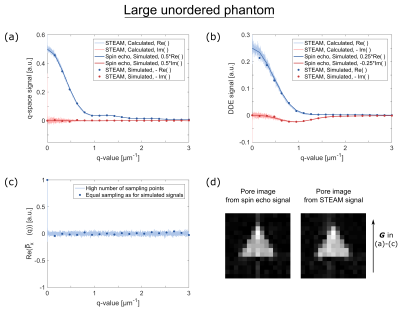
In Fig.$$$~$$$2-4, spin echo and STEAM signals were simulated for the vertical gradient direction with $$$\alpha_i=0$$$ for all 90°-pulses. For the q-space and DDE signal, analytical calculations (light-colored lines) for the respective $$$\tilde{P}_{\boldsymbol{x}_{\text{cm}}}(\boldsymbol{q})$$$ are shown and for fewer sampling points Monte-Carlo spin echo (darker-colored lines) and STEAM signals (dots). For the rock/tissue sample (Fig.$$$~$$$2), Monte Carlo simulations fit very well with the analytical derivations and pore images show clearly discernible triangles. 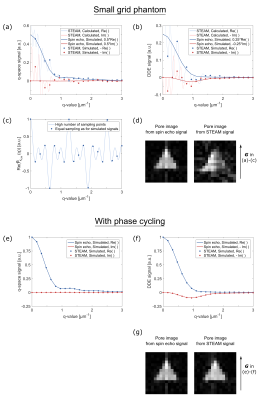For a reduced number of pores, the amplitude of $$$\tilde{P}_{\boldsymbol{x}_{\text{cm}}}(\boldsymbol{q})$$$ does not drop as low and the STEAM signal can deviate from the spin-echo result if a sampling point hits occasionally an outlier of $$$\tilde{P}_{\boldsymbol{x}_{\text{cm}}}(\boldsymbol{q})$$$, see Fig.$$$~$$$3 for a phantom similar to ^5,10-12. If the pore number is decreased further, the contribution of the three undesired terms in $$$S_{\text{DDE}}(\boldsymbol{q})$$$ gets stronger [Fig.$$$~$$$4(a)-(d)].

Discussion

For experiments, a smoothing of the STEAM signals might occur due to inhomogeneity of the gradient field thus effectively averaging over a small span of q-values so that the influence of the three undesired terms in $$$S_{\text{DDE}}(\boldsymbol{q})$$$ goes unnoticed. But if $$$\tilde{P}_{\boldsymbol{x}_{\text{cm}}}(\boldsymbol{q})$$$ does not drop to zero fast enough, phase cycling can be utilized to remove them: Following Tab.$$$~$$$1(b), the combination $$$\text{add}\,0\,(0\,0)\,(0\,0)\,0-\text{add}\,0\,\left(\frac{π}{2}\,\frac{π}{2}\right)\,\left(\frac{π}{2}\,\frac{π}{2}\right)\,0-\text{substract}\,0\,(0\,0)\,\left(\frac{π}{2}\,\frac{π}{2}\right)\,0-\text{subtract}\,0\,\left(\frac{π}{2}\,\frac{π}{2}\right)\,(0\,0)\,0$$$ results in pure $$$e^{i\left(\frac{φ_1}{2}-φ_2+\frac{φ_3}{2}\right)}$$$-term, yielding the desired spin echo signal [Fig.$$$~$$$4(e)-(g)].

Conclusion

Acknowledgements

References

1. Laun FB, Kuder TA et al., Determination of the defining boundary in nuclear magnetic resonance diffusion experiments, Phys. Rev. Lett. 107, 048102 (2011).

2. Laun FB, Kuder TA et al., NMR-based diffusion pore imaging, Phys. Rev. E 86, 021906 (2012).

3. Kuder TA, Bachert P et al., Diffusion pore imaging by hyperpolarized xenon-129 nuclear magnetic resonance, Phys. Rev. Lett. 111, 028101 (2013).

4. Hertel S, Hunter M et al., Magnetic resonance pore imaging, a tool for porous media research, Phys. Rev. E 87, 030802 (2013).

5. Hertel SA, Wang X et al., Magnetic-resonance pore imaging of nonsymmetric microscopic pore shapes, Phys. Rev. E 92, 012808 (2015).

6. Shemesh N, Westin CF et al., Magnetic resonance imaging by synergistic diffusion-diffraction patterns, Phys. Rev. Lett. 108, 058103 (2012).

7. Kuder TA and Laun FB, NMR-based diffusion pore imaging by double wave vector measurements, Magn. Reson. Med. 70, 836-841 (2013).

8. Demberg K, Laun FB et al., Nuclear magnetic resonance diffusion pore imaging: Experimental phase detection by double diffusion encoding, Phys. Rev. E 95, 022404 (2017).

9. Khrapitchev AA and Callaghan PT, Double PGSE NMR with Stimulated Echoes: Phase Cycles for the Selection of Desired Encoding, J. Magn. Reson. 152, 259-268 (2001).

10. Avram L, Assaf Y et al., The effect of rotational angle and experimental parameters on the diffraction patterns and micro-structural information obtained from q-space diffusion NMR: implication for diffusion in white matter fibers, J. Magn. Reson. 169, 30-38 (2004).

11. Bar-Shir A, Avram L et al., The effect of the diffusion time and pulse gradient duration ratio on the diffraction pattern and the structural information estimated from q-space diffusion MR: experiments and simulations, J. Magn. Reson. 194, 230-236 (2008).

12. Shemesh N, Özarslan E et al., Noninvasive bipolar double-pulsed-field-gradient NMR reveals signatures for pore size and shape in polydisperse, randomly oriented, inhomogeneous porous media, J. Chem. Phys. 133, 044705 (2010).