1073

Ultra-Fast 4D MR Elastography using Down-Stream Echoes
Christian Guenthner1, Sweta Sethi2, Ayse Sila Dokumaci3, Ralph Sinkus3, and Sebastian Kozerke1

1Institute for Biomedical Engineering, University and ETH Zurich, Zurich, Switzerland, 2Division of Research Oncology, Guy's and St Thomas' NHS Foundation Trust, London, United Kingdom, 3Division of Imaging Sciences and Biomedical Engineering, King's College London, London, United Kingdom

Synopsis

We propose the readout of the lowest order SSFP echo also referred to as down-stream echo of a phase-locked, spoiled SSFP sequence and utilize the unbalanced gradient moment as a highly efficient motion encoding gradient for ultra-fast MRE and demonstrate its feasibility for rapid breast MRE.

Introduction

Magnetic Resonance Elastography allows the in-vivo determination of the local tissue shear stiffness by time-resolved measurement of the 3D displacement field induced by an external actuator1. In conventional MRE, either SE or GRE-based phase-contrast MRE sequences are used, where a 0th moment-nulled motion encoding gradient (MEG) leads to a phase-shift proportional to the displacement field1,2. In this work, we propose a new type of fractional GRE-MRE sequence that utilizes the first order SSFP echo (down-stream echo), which is refocused by playing the spoiling gradient before instead of after the readout3,4. Here, the spoiling gradient serves as a highly efficient unipolar MEG allowing to reduce scan duration by more than 3-fold. By varying the spoiling direction in subsequent acquisitions, the full 4D MRE dataset can be acquired.

Theory

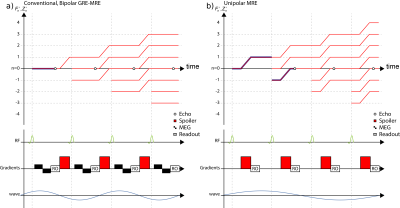
The gradient spoiling in conventional GRE sequences does not destroy transverse magnetization but only causes its dephasing, which can be refocused by reversing the dephasing before each readout4,5. The evolution of these dephased configuration states, which form the up- and down-stream echoes when refocused, can be appreciated using extended phase graphs (EPG)3,6, which is sketched for a conventional spoiled GRE-MRE sequence in Figure 1a.

Shifting the spoiling gradient before the readout, the first down-stream echo F-1 can be read out, which is depicted in Figure 1b. Since the unipolar spoiling gradient is unbalanced, its motion sensitivity can be orders of magnitude larger than a respective bipolar MEG. By changing the spoiling direction after the full 3D volume and all wave offsets are acquired, it is possible to sensitize the sequence to arbitrary motion directions allowing for the acquisition of a full 4D MRE dataset. A motion encoding scheme, e.g. Hadamard encoding7, is required to correct for phase contributions of imaging gradients.

The encoding efficiency of the proposed “Unipolar-MRE” (UP-MRE) sequence benefits two-fold: (a) The encoding efficiency of an infinite-slew unipolar spoiling gradient of strength $$$G$$$ and encoding fraction $$$q$$$ is given by$$\epsilon_f=\gamma{}G\frac{\left|\text{sin}\left(\pi{}q\right)\right|}{\pi{}f}.$$Hence, especially in the low $$$q$$$-regime, the encoding efficiency of unipolar gradients is proportional to $$$q$$$, whereas bipolar gradients scale with $$$q^2$$$, assuming the wave frequency $$$f$$$ is constant. (b) The signal of the F-1-echo is a superposition of two imaging shots allowing for constructive interference. In Figure 2, the encoding efficiency of conventional GRE-MRE is compared to the proposed UP-MRE sequence. For a typical encoding fraction $$$q$$$, the encoding efficiency of UP-MRE is more than 20x higher than the respective GRE-MRE sequence.

Methods

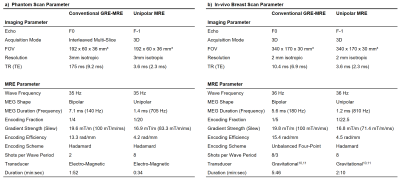
The sequence was implemented on a Philips Achieva system and wave data were acquired in a phantom (1.5T) and the breast (3T) of one volunteer. Reference measurements were performed using multi-slice, multi-shot and 3D fractional GRE-MRE sequences8. All acquisitions used sequential Cartesian readout and wave frequencies of about 35 Hz. The complete list of scan parameter is given in Table 1. A FEM-based wave inversion algorithm was used to obtain shear velocity maps9.

Results & Discussion

In Figure 3, a comparison of the Unipolar-MRE technique and conventional GRE-MRE is shown for an ultra-sound gel phantom. Acquisition duration of the GRE-MRE scan was 1:54min, compared to 0:34min for UP-MRE. Magnitude images show pronounced intra-voxel phase dispersion (IVPD) in the UP-MRE acquisition due to the use of 3D encoding. The real part of the complex displacement differs between the two techniques, as the phase accumulation in UP-MRE is given by the sum of two subsequent phase offsets, while conventional MRE only sensitizes to one time point. Simulation of the UP-MRE acquisition by summation of adjacent phase offsets of the conventional MRE acquisition yields displacement fields very similar to the UP-MRE acquisition.

In Figure 4, preliminary in-vivo breast data of the proposed UP-MRE scheme (2:10min) are shown and were compared to conventional 3D GRE-MRE (5:46min). The deviation from a perfect sinusoidal point-wise wave modulation (non-linearity) of the UP-MRE scan is especially low in the region of the axial lymph nodes (arrows), which leads to good reconstruction results in these regions. Due to partial saturation caused by the low repetition time as well as out-of-phase water-fat imaging in UP-MRE, stiffness values within the parenchymal areas are underestimated. SNR in these areas can be increased by using lower flip-angles and in-phase echo times.

Conclusions

We have demonstrated the feasibility of using unipolar gradients as highly efficient MEGs allowing to use encoding fractions surpassing significantly bipolar encoding approaches, while greatly reducing repetition times. Collectively, this reduces total acquisition time by about a factor 3 when compared to an equivalent 3D GRE sequence. While unipolar gradients cannot be employed in conventional GRE-MRE sequences, we have proposed to use them to refocus the first down-stream echo and successfully demonstrated Unipolar-MRE in both phantom and breast.

Acknowledgements

This project has received funding from the European Union’s Horizon 2020 research and innovation programme under grant agreement No 668039.

References

1. Muthupillai R, Lomas D, Rossman P, Greenleaf J, Manduca A, Ehman R. Magnetic resonance elastography by direct visualization of propagating acoustic strain waves. Science (80- ). 1995;269(5232):1854-1857. doi:10.1126/science.7569924.

2. Sinkus R, Lorenzen J, Schrader D, Lorenzen M, Dargatz M, Holz D. High-resolution tensor MR elastography for breast tumour detection. Phys Med Biol. 2000;45(6):1649-1664. doi:10.1088/0031-9155/45/6/317.

3. Hennig J. Multiecho imaging sequences with low refocusing flip angles. J Magn Reson. 1988;78(3):397-407. doi:10.1016/0022-2364(88)90128-X.

4. Mizumoto CT, Yoshitome E. Multiple echo SSFP sequences. Magn Reson Med. 1991;18(1):244-250. doi:10.1002/mrm.1910180126.

5. Heule R, Ganter C, Bieri O. Triple echo steady-state (TESS) relaxometry. Magn Reson Med. 2014;71(1):230-237. doi:10.1002/mrm.24659.

6. Weigel M. Extended phase graphs: Dephasing, RF pulses, and echoes - pure and simple. J Magn Reson Imaging. 2015;41(2):266-295. doi:10.1002/jmri.24619.

7. Guenthner C, Runge JH, Sinkus R, Kozerke S. Hadamard Encoding for Magnetic Resonance Elastography. In: Intl. Soc. Mag. Reson. Med. 25. Honolulu; 2017:1378.

8. Rump J, Klatt D, Braun J, Warmuth C, Sack I. Fractional encoding of harmonic motions in MR elastography. Magn Reson Med. 2007;57(2):388-395. doi:10.1002/mrm.21152.

9. Fovargue D, Sinkus R, Nordsletten D. Robust MR Elastography Stiffness Quantification Using a Localized Divergence Free Finite Element Reconstruction. In: Intl. Soc. Mag. Reson. Med. 25. Honolulu; 2017:1368.

10. Runge J, Hoelzl S, Sudakova J, et al. A Novel MR Elastography Transducer Concept Based on a Rotational Eccentric Mass: The Gravitational Transducer. In: Intl. Soc. Mag. Reson. Med. 25. Vol 1369. Honolulu; 2017.

11. Hoelzl S, Sethi S, Sudakova J, et al. Enabling High Resolution MRE Images of the Breast. In: Intl. Soc. Mag. Reson. Med. 25. Honolulu; 2017:4938.

Figures

Figure 1: Sequence schematics and EPG of fractional GRE-MRE and the proposed unipolar MRE. For simplicity, only the spoiling gradients are shown, thus assuming balanced imaging gradients, which are omitted. GRE-MRE is sensitized to shear motion using bipolar MEGs (black, left image). The Unipolar MRE sequence utilizes a unipolar, unbalanced gradient, which is played out before the readout, to (a) refocus the F-1 echo and (b) to sensitize the sequence to motion. As the F-1 echo is excited and then dephased and only refocused in the subsequent repetition time, UP-MRE is sensitive to the sum of two adjacent wave-phases.

Figure 2: Absolute and relative encoding efficiency of conventional, bipolar GRE-MRE and the proposed UP-MRE sequence. In the low q regime, unipolar MEGs are proportional to the encoding fraction q, whereas bipolar gradients exhibit a squared dependence. The encoding efficiency of UP-MRE is based on the acquisition of 8 wave phases within one wave period, as it is the case for both phantom and in-vivo scans presented here. For the phantom scans, UP-MRE with 705 Hz unipolar MEGs corresponding to $$$q=1/20$$$ at 35 Hz wave frequency, is 23.5-times more efficient as a comparable conventional MRE acquisition using bipolar MEGs.

Table 1: Sequence parameter table for a) the phantom comparison and b) the in-vivo breast scan.

Figure 3: Comparison of conventional multi-slice GRE-MRE with bipolar gradients and the proposed 3D Unipolar MRE (UP-MRE). Magnitude images show pronounced IVPD in the UP-MRE acquisition due to the high motion sensitivity of the F-1 echo and the use of 3D encoding. The real part of the complex displacement differs between the two techniques, as the phase accumulation in UP-MRE is given by the sum of two subsequent phase offsets, while conventional MRE only sensitizes to one time point. Simulation of the UP-MRE acquisition by summation of adjacent phase offsets of the conventional MRE acquisition yields displacement fields comparable to the UP-MRE acquisition.

Figure 4: Preliminary results at 36 Hz, comparing the conventional GRE-MRE protocol to the proposed unipolar MRE sequence. The UP-MRE displacement is a superposition of two adjacent phase offsets. UP-MRE shows low non-linearity in the domain of the axial lymph nodes, while the short TR causes partial saturation in the breast leading to low signal amplitude and hence high non-linearity. Shear wave velocity in the breast is underestimated by UP-MRE due to the low signal; however, the heightened stiffness of the axial lymph nodes is captured as opposed to conventional GRE-MRE.

Proc. Intl. Soc. Mag. Reson. Med. 26 (2018)
1073