0935
Accelerated Spiral imaging for Real-time Cardiac MRI1Department of Biomedical Engineering, University of Virginia, Charlottesville, VA, United States
Synopsis
This study proposes two spiral-based (spiral-out and spiral-in/out) bSSFP pulse sequences combined with two reconstruction methods for accelerated real-time cardiac imaging. Based on a comparison to fully-sampled data reconstructed using gridding, a low rank plus sparse (L&S) method performs better than a CG-SPIRiT method on both spiral trajectories. The spiral-in/out sequence achieved higher SNR and fewer artifacts than the spiral-out sequence. Thus, a spiral-in/out bSSFP sequence with L&S reconstruction is a promising method for real-time cardiac MRI with high image quality and excellent temporal resolution.
Introduction:
The traditional method for assessing cardiac function is to image during a breath-hold using an ECG-gated balanced steady-state free precession (bSSFP) pulse sequence with segmented Cartesian readouts. However, this method can yield poor image quality in patients who have arrhythmia or who are incapable of holding their breath. Thus, a real-time bSSFP pulse sequence would be of great value in the assessment of cardiac function without breath-holding.
Recently, a non-Cartesian bSSFP method1 was proposed for real-time cardiac imaging and spiral-based sequences have shown improved image quality in terms of SNR and reduced artifacts when compared with other techniques. However, the relatively low temporal resolution of these methods is a limitation relative to gated sequences. Hence, in this study spiral-based bSSFP sequences combined with parallel imaging and model-based temporal sparsity reconstruction2 were proposed to achieve a high acceleration.
Method:
Interleaved spiral-out and spiral-in/out bSSFP with linearly decreasing sampling density were both evaluated in this study. Both fully-sampled spiral trajectories contain 32 arms with 1.6 ms readout per arm, and four interleaves 90° apart from each other are utilized to reconstruct each frame with an undersampling rate of 8. Among frames the interleaves are rotated in a bit-reversed order to reduce temporal correlation. FOV was set to 340 × 340 mm2 for all of the experiments. Other sequence parameters and the resulting spatial and temporal resolutions are given in Table 1. A model-based k-space trajectory method3 was used to estimate the actual spiral trajectory for both sequences. A low resolution field map was acquired using two single-shot spirals before bSSFP acquisitions.
Two acceleration methods were used for reconstruction: CG-SPIRiT4 and low rank plus sparse (L&S)5. The field map data was used for the calibration kernel of CG-SPIRiT. For the L&S method, the low rank constraint was enforced for all time points with the same static cardiac structure and a joint sparsity constraint was used because the sparsity of different phases of cardiac circle between interleaves is assumed to be highly correlated. As multiple receiver coils were used in this study, the coil sensitivity was estimated from center k-space data of the field map using ESPIRiT6 and then used for L&S reconstruction. Low resolution linear field map and Chebyshev-based off-resonance correction7 methods were used for the gridding reconstruction without acceleration, CG-SPIRiT and L&S methods.
All the experiments were performed on a 1.5T scanner (MAGNETOM Avanto, Siemens Healthcare, Erlangen, Germany) with a 32-channel surface coil array. For each healthy volunteer, a midventricular short-axis view and a horizontal long-axis view were imaged under free-breathing conditions. For each set of experiments, the spiral-out and spiral-in/out bSSFP sequences were run consecutively at the same image plane with 30 fully-sampled frames per sequence collection.
Results and Discussion:
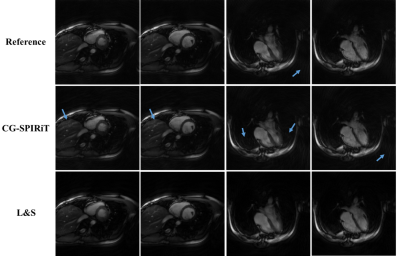
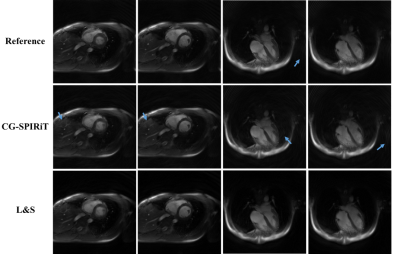
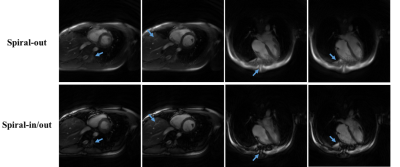
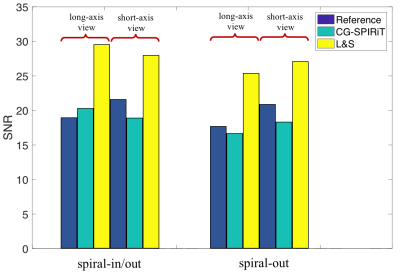
The reconstructed dynamic image series from spiral-in/out and spiral-out bSSFP sequence are shown in Figs. 1 and 2. For each spiral sequence, 30 fully-sampled frames from the gridding reconstruction were set to be the reference images. For the CG-SPIRiT and L&S methods, 8-fold acceleration was achieved and a total of 240 frames were reconstructed. Fig. 1 shows the systolic and diastolic frames from short-axis and long-axis free-breathing experiments using the spiral-in/out based bSSFP sequence. From top to bottom row are the gridding reconstruction without any acceleration, CG-SPIRiT and L&S method, respectively. The blue arrows point to structures from the CG-SPIRiT method that show obvious noise-like aliasing artifacts, and the L&S method shows clear image quality improvement. Fig. 2 shows similar results when using the spiral-out trajectory, demonstrating the fidelity of the L&S method. Similar frames from these two spiral trajectories using L&S reconstruction are compared in Fig. 3. The blue arrows point to fine structures from the spiral-in/out image that show details which are blurred in the spiral-out image. Fig. 4 shows the apparent SNR increases when using the L&S reconstruction method or the spiral-in/out trajectory, and the highest SNR was achieved when the spiral-in/out trajectory was combined with L&S reconstruction.Conclusion:
In this study accelerated real-time spiral-based bSSFP sequences were implemented and tested in cardiac imaging. The results show that a spiral-in/out bSSFP sequence combined with low rank plus sparse reconstruction had the best image quality for highly undersampled data, with minimal temporal blurring and reduced artifacts. The resulting method yields ungated spiral cardiac movies at 68 frames per second.Acknowledgements
No acknowledgement found.References
[1] Feng X, Salerno M, Kramer C M, Meyer CH. Non-Cartesian balanced steady-state free precession pulse sequences for real-time cardiac MRI[J]. Magn Reson Med. 2016, 75(4):1546.
[2] Kim YC, Narayanan SS, Nayak KS. Flexible retrospective selection of temporal resolution in real-time speech MRI using a golden-angle spiral view order. Magn Reson Med. 2011. 65(5):1365-1371.
[3] Tan H, Meyer CH. Estimation of k-space trajectories in spiral MRI. Magn Reson Med 2009;61:1396–1404.
[4] Lustig M, Pauly JM. SPIRiT: iterative self-consistent parallel imaging reconstruction from arbitrary k-space. Magn Reson Med 2010;64:457– 471.
[5] Otazo R, Candes E, Sodickson DK. Low-rank plus sparse matrix decomposition for accelerated dynamic MRI with separation of background and dynamic components. Magn Reson Med. 2014. 73(3): 1125-1136.
[6] Uecker M, Lai P, Mruphy MJ, et al. ESPIRiT--an eigenvalue approach to autocalibrating parallel MRI: where SENSE meets GRAPPA. Magn Reson Med. 2014. 71(3): 990-1001.
[7] Chen W, Sica CT, Meyer CH. Fast conjugate phase image reconstruction based on a Chebyshev approximation to correct for B0 field inhomogeneity and concomitant gradients. Magn Reson Med. 2008. 60(5): 1103-1111.
Figures