0888
On the vanishing of the t-term in the short-time expansion of the diffusion coefficient for oscillating gradients in diffusion NMR1Institute of Radiology, University Hospital Erlangen, Friedrich-Alexander-Universität Erlangen-Nürnberg (FAU), Erlangen, Germany, 2Medical Physics in Radiology, German Cancer Research Center, Heidelberg, Germany
Synopsis
The short-time expansion of the diffusion coefficient in powers of t1/2 is universally connected to structural parameters of the boundaries restricting the diffusive motion. The t1/2-term is proportional to the surface-to-volume ratio. The t-term is related to permeability and curvature. The short-time expansion can be measured by application of oscillating gradients of long total duration. For oscillating gradients, the inverse of the oscillation frequency becomes the relevant time scale. The purpose of this work is to show that the oscillating gradient approach is blind to the t-term. Thus, the t-term does not bias the determination of the t1/2-term in experiments.
INTRODUCTION
The short-time expansion of the diffusion coefficient in powers of t1/2, where t is the diffusion time, is universally connected to structural parameters of the boundaries restricting the diffusive motion1. The t1/2-term is proportional to the surface to volume ratio. The t-term is related to permeability and curvature. The short-time expansion can be measured by the use of diffusion encodings of short total duration or by application of oscillating gradients of long total duration2. For oscillating gradients, the inverse of the oscillation frequency becomes the relevant time scale. The purpose of this work is to show that the oscillating gradient approach is blind to the t-term.
Theory
The effective diffusion coefficient in the short-time limit is
D(t)=<(x(t)−x(0))2>2t=<x(t)2+x(0)2−2x(t)x(0)>2t=∑n=0Mncntn/2with the t0-term M0=D0, the t1/2-termM_1=-\frac{4}{3d\sqrt{π}}\frac{S}{V}\sqrt{D_0},and the t-termM_2=\frac{S}{V}(\frac{1}{d}\kappa-\frac{1}{12}D_0\overline{R^{-1}}).Here, D_0 is the free diffusion coefficient, S/V is the surface-to-volume ratio, d is the dimension, \kappa is the membrane permeability, and \overline{R^{-1}} is a term related to the membrane curvature. In diffusion MR experiments, <x(t)^2> and <x(0)^2> do not contribute to the signal attenuation due to the rephasing condition. Hence the diffusion spectrum \mathcal{D}(\omega) may be written as\frac{\mathcal{D}(\omega)}{\omega^2}=\frac{1}{2}\int_{-\infty}^\infty⟨x(t_2 )x(t_1 )⟩ e^{-i\omega t_{21}} dt_{21}=-\frac{1}{2}\sum_{n=0}\int_{-\infty}^\infty M_n |t_{21}|^{1+n/2}e^{-i\omega t_{21}}dt_{21}.with t_{21}=t_2-t_1. The last term is a Fourier transform: \frac{1}{2}\int_{-\infty}^\infty |t_{21}|^{1+n/2}e^{-i\omega t_{21}}dt_{21}=-\omega^{-2-n/2} cos(n\pi/4)\Gamma(2+n/2) for \omega>0 and n≥0. Hence one finds:\mathcal{D}(\omega)=M_0+M_1 \sqrt{\frac{π}{2}}\frac{3}{4\omega^{1⁄2}}+0\cdot M_2+O(\omega^{-3⁄2} )and\mathcal{D}_{app}(\tau):=\mathcal{D}(2\pi/\tau)=D_0+M_1\frac{3}{8}\tau^{1⁄2}+0\cdot M_2+O(\tau^{3⁄2}). Thus the t-term is not visible in oscillating gradient diffusion MR diffusion experiments, which build on the experimental detection of D(\omega). Note that the value of coefficient accompanying the t^{1/2}-term was reported previously (e.g. in 3).
Methods
The diffusion spectrum was computed using the multiple correlation function (MCF) approach using Eq. 114 in 4. The following closed domains were considered: slab, cylinder, sphere, “bi-slab”. The bi-slab domain consists of three parallel planes. The inner plane is permeable (\kappa= 50 µm/s), while the two outer ones are impermeable. The radii of cylinder and sphere were 5 µm. For the slab domain and the bi-slab domain, the separation of the planes was 10 µm (thus the bi-slab was in total 20 µm wide). D_0 was set to 1 µm²/ms. Oscillating cosine gradients were simulated with a total duration of 0.5 s. For bipolar gradients, simulations were performed with a gradient pulse duration of 0.001 ms.Results
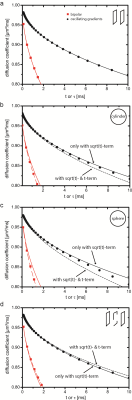
Figure 1 displays D(t) and \mathcal{D}_{app}(\tau). Markers indicate simulation results using the MCF approach and lines represent the short-time expansion. Solid lines represent the short time expansion to order t^{1/2} and dotted lines represent it to order t. For \mathcal{D}_{app}(\tau), the dotted line shall represent a reasonable “guess” for the t-term, i.e. M_2\cdot1\cdot\tau\cdot3^2/8^2 with the effective diffusion time \tau\cdot3^2/8^2. The intention is to visualize this line although this term does not occur in reality. For the slab domain (Fig. 1a), the t-term is zero. Hence, Fig. 1a does not display a dotted line and markers stay close to the solid lines. In Fig. 1b,c (cylinder, sphere) and 1d (bi-slab), it is clearly visible that the markers for the bipolar gradients stay close to the dotted lines indicating the importance of the t-term. The markers of the oscillating cosine gradients stay close to the solid line indicating that the t-term does not influence \mathcal{D}_{app}(\tau) . Owing to higher order terms, deviations between the short-time expansion and markers are present at larger t.Discussion
As oscillating gradients are blind to the t-term, estimates of S/V as in 5 are not biased by this term, but, obviously, the membrane permeability, for example, cannot be estimated using the t-term. This is in line with the findings by Li et al. 6, who reported that the membrane permeability has little effect on oscillating gradient derived diffusion coefficients at high frequencies. This is presumably not a major limitation given the smallness of the t-term that is visible in Fig. 1, which makes a fit challenging. Interestingly, the disappearance of the t-term in the short-time expansion using oscillating gradients is due to its disappearance in \mathcal{D}_{app}(\tau). Thus optimizing oscillating gradient profiles instead of using, for example, just cosine gradients, which was a successful approach in other regards 7,8, does not help to make the t-term reappear in the signal attenuation.Conclusion
As we have also recently outlined in 9 in more detail, oscillating gradients are blind to the t-term and hence no bias in fits of the surface-to-volume ratio arises from it.Acknowledgements
Financial support by the DFG (grant numbers LA 2804/6-1, SFB TRR 125/2 R01 and KU 3362/1-1) is gratefully acknowledged.References
1. Mitra PP, Sen PN, Schwartz LM, Ledoussal, P. Diffusion Propagator as a Probe of the Structure of Porous-Media. Phys Rev Lett 1992;68:3555-3558.
2. Stepišnik, J. Analysis of Nmr Self-Diffusion Measurements by a Density-Matrix Calculation. Physica B & C 1981;104:350-364.
3. Novikov, DS, Kiselev, VG. Surface-to-volume ratio with oscillating gradients. J Magn Reson 2011;210: 141-145.
4. Grebenkov, DS. NMR survey of reflected Brownian motion. Reviews of Modern Physics 2007;79: 1077-1137.
5. Reynaud O, Winters KV, Hoang DM, et al. Surface-to-volume ratio mapping of tumor microstructure using oscillating gradient diffusion weighted imaging. Magn Reson Med 2016;76:237-247.
6. Li H, Jiang XY, Xie JP, et al. Time-Dependent Influence of Cell Membrane Permeability on MR Diffusion Measurements. Magn Res Med 2016;75:1927-1934.
7. Drobnjak I, Siow
B, Alexander DC. Optimizing gradient waveforms for
microstructure sensitivity in diffusion-weighted MR. J. Magn. Reson. 2010;206:41-51
8.
Siow
B, Drobnjak I, Chatterjee A, et al.
Estimation of pore size in a microstructure phantom using the optimised
gradient waveform diffusion weighted NMR sequence. J Magn Reson 2012;214:51-60.
9. Laun FB, Demberg K, Nagel AM, et al.
On the
vanishing of the t-term in the
short-time expansion of the diffusion coefficient for oscillating gradients in
diffusion NMR. Front. Physics. Accepted.
Figures