0885

Diffusion Time Dependence of NODDI in in vivo Human White Matter
Masaaki Hori1, Kouhei Kamiya2, Katsutoshi Murata3, Thorsten Feiweier4, Issei Fukunaga1, Akifumi Hagiwara1,2, Ryusuke Irie1,2, Christina Andica1, Tomoko Maekawa1,2, Saori Koshino1,2, Koji Kamagata1, Kanako Kunishima Kumamaru1, Michimasa Suzuki1, Akihiko Wada1, and Shigeki Aoki1

1Radiology, Juntendo University School of Medicine, Tokyo, Japan, 2Radiology, The University of Tokyo Graduate School of Medicine, Tokyo, Japan, 3Siemens Japan K.K., Tokyo, Japan, 4Siemens Healthcare GmbH, Erlangen, Germany

Synopsis

We investigated the diffusion time dependency of diffusion metrics, especially ICVF and INVF, compared with ADC using an oscillating gradient spin-echo sequence in in vivo human white matter at 3T. Our results show that the change ratio of both ICVF and INVF indicate similar tendency in the same white matter locations, with or without diffusivity calculation. Moreover, with higher oscillating frequency, ICVF decreased but ISOVF stayed almost unchanged, indicating that the rate of ICVF and the extra-cellular component may change with oscillating frequency beside the unchanged isotropic water component.

Introduction

Neurite orientation dispersion and density imaging (NODDI) is a model-based diffusion-weighted (DW) MRI technique that can estimate specific microstructural features of neuronal tissue morphology1. The key to this technique is a 3-compartment tissue model, including restricted intracellular compartment including axons (ICVF), hindered extracellular compartment, and cerebrospinal fluid (free water molecules, ISOVF) to analyze data from diffusion MRI. Moreover, NODDI can provide information of orientation dispersion as an index (ODI) to show the axon distribution. Thus, this model may overcome the limitation of conventional diffusion analysis, such as diffusion tensor imaging2, 3. NODDI calculations rely on two basic assumptions: the intrinsic water diffusivity: = λint || = λext = 1.7 μm2 /ms and the tortuosity effect: λext⊥= (1 − vint)λ. Kaden et al. pointed out that assuming a fixed diffusivity is a limitation of NODDI and they proposed multi-compartment spherical mean technique ( SMT) to calculate the intra-neurite volume fraction, which is similar to ICVF, but without fixed intrinsic diffusivity values4. Recently, time-dependent diffusion changes have become a topic for diffusion MRI analysis because quantitative diffusion metrics change with different diffusion time Δin tissue microstructures5-7. Observations of time-dependent diffusion parameters have been reported in in vivo brain of human subjects at times ranging from 40 to 800 ms8. It is known that oscillating gradient spin echo (OGSE) diffusion-weighted sequences are able to probe shorter diffusion times compared to the pulsed gradient spin echo (PGSE). In humans, Baron et al. combined OGSE and PGSE for a total diffusion time range from 4 ms to 40 ms, and found brain to exhibit time-dependent diffusion9. The purpose of this study is to investigate diffusion time dependency of diffusion metrics, especially ICVF and INVF (intra-neurite volume fraction), compared with apparent diffusion coefficient changes with different diffusion time in vivo.

Methods

Twenty-three normal volunteers (mean 73 y.o., 13 women and 10 men) were scanned on a 3T MR scanner (MAGNETOM Prisma, Siemens Healthcare, Erlangen, Germany) with a 64-channel head/neck coil. Imaging parameters for trapezoid cosine-modulated OGSE dMRI10 (employing a prototype sequence) were as follows: repetition time/echo time, 7800ms/168ms; section thickness, 4 mm; 30 slices; field of view, 200 x 200 mm2; matrix, 164 x 164; imaging time, approximately 7 min for each; 2 b values (800 s/mm2 and 1655 s/mm2) with a b=0 image and diffusion encoding in 12 direction for every b value; frequency=0Hz, 20Hz, 30Hz (corresponding effective Δ＝57.5ms, 9.3ms, 6.5ms, respectively).

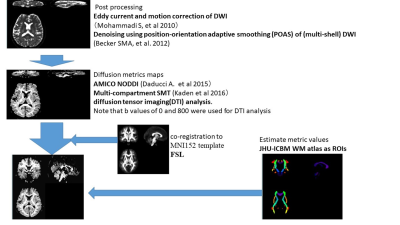
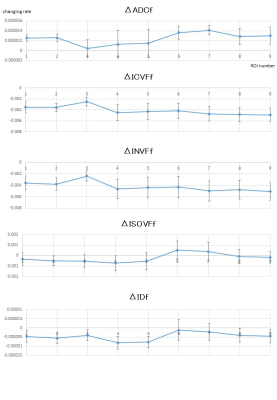
After eddy current11 and motion correction12 of DWI data, diffusion metrics maps were generated. The following diffusion metrics are considered; apparent diffusion coefficient (ADC), ICVF and ISOVF from Accelerated Microstructure Imaging via Convex Optimization NODDI analysis and INVF, and intrinsic diffusivity (ID) from multi-compartment SMT. We used the FMRIB Software Library (Oxford, UK.) linear image registration tool to register all diffusion metrics maps to the MNI152 template. We used Johns Hopkins University (JHU) ICBM-DTI-81 white-matter (WM) labels atlas for specifying WM ROIs and 9 ROIs were selected for evaluation. These procedures are summarized in Figure 1. Moreover, the rate of ADC, ICVF, INVF, ISOVF and ID change with oscillating frequency (e.g. ΔADCf ) was calculated by the linear fitting in Matlab (www.mathworks.com). Correlations between the ratio of changes in ICVF and ADC and in INVF and ADC were calculated on a voxel-by-voxel basis.

Results

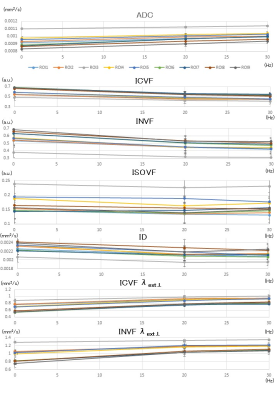
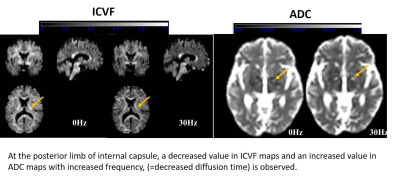
The results are shown in the graphs (Figures 2 and 3). Briefly, ADC increased, ICVF, INVF and ID decreased, and ISOVF stayed constant with the decrease of diffusion time (= increase of frequency). The change rates of each diffusion metric with oscillating frequency depend on the location of white matter. Moreover, there were weak correlations between the ratio of changes in ICVF and ADC (r=0.32, Pearson correlation coefficient) and in INVF and ADC (r=0.24). Representative ICVF and ADC maps are shown in Figure 4.

Discussion and Conclusion

The results show the diffusion time dependency of ICVF and INVF, in addition to ADC. It is interesting that the change rates of both ICVF and INVF indicate similar tendency in the same white matter location, with or without intrinsic diffusivity calculation. Moreover, with higher oscillating frequency, ICVF decreased but ISOVF stayed almost unchanged, indicating that the rate of ICVF and extra-cellular component may change with oscillating frequency beside the unchanged isotropic water component. In conclusion, diffusion time dependence is confirmed on NODDI metrics in in vivo white matter and we should pay attention to the diffusion times for interpretation of the ICVF and other diffusion metrics for clinical use.

Acknowledgements

This work was supported by JSPS KAKENHI Grant Number 16K10328.

References

  1. Zhang H, et al. NODDI: practical in vivo neurite orientation dispersion and density imaging of the human brain. Neuroimage. 2012;61(4):1000-16.
  2. Grussu F, et al. Neurite orientation dispersion and density imaging of the healthy cervical spinal cord in vivo. Neuroimage. 2015;111:590-601.
  3. Deligianni F, et al. NODDI and Tensor-Based Microstructural Indices as Predictors of Functional Connectivity. PloS one. 2016;11(4):e0153404
  4. Kaden E, et al. Multi-compartment microscopic diffusion imaging. Neuroimage. 2016 Oct 1;139:346-359.
  5. Fieremans E, et al. In vivo observation and biophysical interpretation of time-dependent diffusion in human white matter. Neuroimage. 2016 Apr 1;129:414-427.
  6. Novikov, D. S., Jensen, J. H., Helpern, J. A., & Fieremans,E. Revealing mesoscopic structural universality with diffusion. Proceedings of the National Academy of Sciences, 2014;111(14), 5088-5093.
  7. Novikov, D. S., & Kiselev, V. G. Effective medium theory of a diffusion-weighted signal. NMR in Biomedicine, 2010 23(7), 682-697.
  8. Horsfield MA, et al. Self-diffusion in CNS tissue by volume-selective proton NMR. Magn Reson Med. 1994 Jun;31(6):637-44.
  9. Baron CA, Beaulieu C. Oscillating gradient spin-echo (OGSE) diffusion tensor imaging of the human brain. Magn Reson Med. 2014 Sep;72(3):726-36.
  10. Van AT, et al. In vivo investigation of restricted diffusion in the human brain with optimized oscillating diffusion gradient encoding. Magn Reson Med. 2014 Jan;71(1):83-94.
  11. Mohammadi S, et al. Correcting eddy current and motion effects by affine whole-brain registrations: Evaluation of three-dimensional distortions and comparison with slicewise correction. Magn Reson Med 2010 64:1047-1056.
  12. Becker SMA, et al. Position-orientation adaptive smoothing of diffusion weighted magnetic resonance data (POAS). Med Image Anal 2012 16:1142-1155.

Figures

Image post processing summary

Changing diffusion metrics with OGSE frequency. ROI list:01 Anterior corona radiate, right, 02 Anterior corona radiata, left, 03 Genu of corpus callosum, 04 Posterior corona radiata, right, 05 Posterior corona radiata, left, 06 Posterior limb of internal capsule, right, 07 Posterior limb of internal capsule, left, 08 Superior corona radiata, right, 09 Superior corona radiata, left ( JHU ICBM-DTI-81 white-matter labels atlas )

Changing rate of each diffusion metrics.

Representative ICVF and ADC maps of 0Hz and 30Hz.

Proc. Intl. Soc. Mag. Reson. Med. 26 (2018)
0885