0761

Geometrical Characterization of Marfan and Bicuspid Aortic Valve Patients Using Finite Element Methods
Julio Sotelo1,2, Andrea Guala3, Lydia Dux-Santoy3, Aroa Ruiz-Muñoz3, Arturo Evangelista3, Joaquín Mura1, Cristian Tejos1,2,4, Daniel E Hurtado4,5, José Rodríguez-Palomares3, and Sergio Uribe1,4,6

1Biomedical Imaging Center, Pontificia Universidad Católica de Chile, Santiago, Chile, 2Department of Electrical Engineering, Pontificia Universidad Católica de Chile, Santiago, Chile, 3Department of Cardiology, Hospital Universitari Vall d´Hebron, Vall d´Hebron Institut de Recerca (VHIR), Universitat Autònoma de Barcelona, Barcelona, Spain, 4Institute for Biological and Medical Engineering, Schools of Engineering, Medicine and Biological Sciences, Pontificia Universidad Católica de Chile, Santiago, Chile, 5Department of Structural and Geotechnical Engineering, Pontificia Universidad Católica de Chile, Santiago, Chile, 6Department of Radiology, School of Medicine, Pontificia Universidad Católica de Chile, Santiago, Chile

Synopsis

BAV patients and patients with MFS are predisposed to develop geometrical changes in the aorta. The geometrical assessment of the aorta using MRI in these patients generates an operator dependency and variability given by the 2D planer reformatting of the data. In this work, we propose finite element Laplace formulation, which allows us to obtain 3D maps of geometrical parameters in the aorta, avoiding the 2D reformatting process of the MRI data. We apply our method on volunteers, BAV and MFS patients. Our method allows applying it to any type of volumetric segmentation from MR or CT.

Introduction

Bicuspid aortic valve (BAV) is known as the most common congenital defect1. This anomaly is associated with typical manifestations of aortic dilatation and an increased risk of dissection and rupture2. Similar manifestations are also a key feature in patients with Marfan syndrome (MFS). Marfan patients exhibit a mutation in the fibrillin-1 gene, that affect markedly the elastic properties of the aorta, decreasing the compliance which generate the dilatation of the aorta3. To evaluate the progression and provide a better treatment to the BAV and MFS patients, the study of the morphometry of the aorta is evaluated as a clinical routine, and is normally assessed by echocardiography, computed tomography angiography, or magnetic resonance angiography (MRA)4. Some studies evaluate the aortic geometry of BAV and MFS patients with MRI5-7, but the principal limitation of these studies is the operator dependency and variability that exist in 2D planer reformatting of the MRI data that lead to regional variations when measuring aortic geometry. Furthermore, the diameter is only measure in some region of the aorta. For this reason, there is a need to generate new methods and markers that avoid these problems. In this work, we propose a methodology based on the Laplace formulation using finite elements (FE) methods that allows us to obtain three-dimensional geometrical parameters (diameter, curvature8, tortuosity8, torsion8, width/high ratio of the aortic arch6) of the aorta of patients with BAV from the angiographic image9 obtained by 4D flow MRI data.

Methods

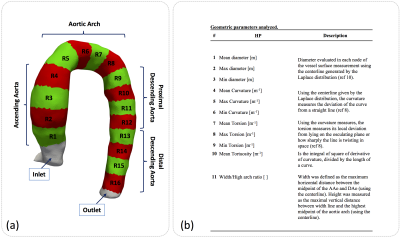
We developed a standard Galerkin FE problem10 to obtain the Laplace distribution from the angiographic image generated by 4D-flow MR data (see figure1). The algorithm used to calculate the geometrical parameters and Laplace distribution was performed on python. A total of 14 healthy volunteer, 10 patients with Marfan syndrome, 19 patients with BAV right / non-coronary (RN) cups fusion and 30 patients with BAV right / left (RL) coronary cusp fusion were included in the study. The 4D flow data was acquired at Hospital Universitari Vall d´Hebron in a 1.5T GE-MR Sigma scanner using the vastly undersampled isotropic projection reconstruction technique11. The patient demographic and MR acquisition parameters are shown in the figure 2. Sixteen different regions were analyzed between volunteer and BAV-patient figure3(a). In each region, each geometrical parameter was analyzed figure3(b). In all these regions, we analyze these geometrical parameters to find differences between volunteers and patients. The normal distribution of each data was studied using the Lilliefors test. Results between patients and volunteers were compared using a t-student test (for normal distribution data) and a wilcoxon test (for non-normal distribution data). Also, we analyze the R2 value of a linear regression between all geometrical parameters to find out if there was any correlation between them.

Results

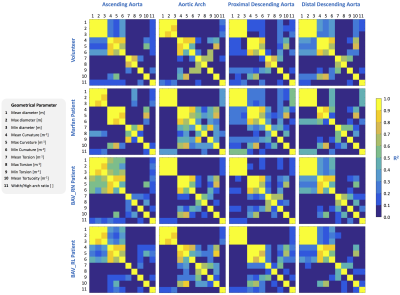
In the Figure4(a)-(d) we show the results of geometrical parameters with significant differences (p<0.05) between volunteers and patients, the yellow box indicates a significant difference between the data. In the subfigures Figure4(a), (b), (c) and (d) we compare volunteers-Marfan, volunteers-BAV, volunteers-BAV_RN and volunteers-BAV_RL respectively. In the Figure4(e), we show the 3D maps of each geometrical parameters, for one; representative subject pertaining to each group. We observed that the geometrical parameters in the regions of the ascending aorta and part of the aortic arch (regions 1 to 8) show more significant differences between volunteers and BAV-patients with RL phenotype, in comparison with RN. Between volunteers and Marfan patients only the mean curvature of the ascending aorta shows more differences that the other parameters. In Figure5, we show the correlation matrix between all geometrical parameters generated for each region (see figure3a), for volunteer and patients (Marfan, BAV_RN, BAV_RL). We observe that the geometrical parameters as diameter and curvature are correlated in the ascending aorta of BAV patients (RN or RL) with a R2 value bigger than 0.5. In Marfan patients we observe that the related parameters are curvature, torsion and tortuosity in the aortic arch.

Discussion and conclusion

We have presented a novel method that allow us the semiautomatic analysis of geometric parameters of the entire aorta in 3D from the angiographic data obtained by 4D flow. The main advance of the propose technique is that it avoids reformatting of the data and reduces the problem of operator dependency and variability. The methodology presented here represents a great advance to fully characterize anatomy of any vessels. We have shown that our method is able to characterize the geometrical values of the aorta in different types of patients.

Acknowledgements

Thank to grant, CONICYT - PIA - Anillo ACT1416, CONICYT FONDEF/I Concurso IDeA en dos etapas ID15|10284, and FONDECYT #1141036. Guala A. has received funding from the European Union Seventh Framework Programme FP7/People under grant agreement n° 267128. Sotelo J. acknowledges to FONDECYT Postdoctorado 2017 #3170737.

References

1. Ward C. Clinical significance of the bicuspid aortic valve. Heart 2000;83:81–5.

2. Fedak PW, David TE, Leask RL, et al. Bicuspid aortic valve disease: recent insights in patho- physiology and treatment. Expert Rev Cardiovasc Ther 2005;3: 295 – 308.

3. Jeremy RW, Huang H, Hwa J, et al. Relation between age, arterial distensibility, and aortic dilatation in the Marfan syndrome. Am J Cardiol 1994;74:369–373

4. Erbel R, Aboyans V, Boileau C, et al. ESC Guidelines on the diagnosis and treatment of aortic diseases. Eur Heart J 2014;35: 2873 – 926.

5. Garcia J, Barker AJ, Murphy I, et al. Four-dimensional flow magnetic resonance imaging-based characterization of aortic morphometry and haemodynamics: impact of age, aortic diameter, and valve morphology. Eur Heart J Cardiovasc Imaging 2016;17(8):877-84.

6. Schnell S, Smith DA, Barker AJ, et al. Altered aortic shape in bicuspid aortic valve relatives influences blood flow patterns. Eur Heart J Cardiovasc Imaging 2016;17(11):1239-1247.

7. van der Palen RL, Barker AJ, Bollache E, et al. Altered aortic 3D hemodynamics and geometry in pediatric Marfan syndrome patients. J Cardiovasc Magn Reson 2017;17;19(1):30

8. Piccinelli M, Veneziani A, Steinman DA, Remuzzi A, Antiga L. A framework for geometric analysis of vascular structures: application to cerebral aneurysms. IEEE Trans Med Imaging. 2009;28(8):1141-55.

9. Johnson KM, Lum DP, Turski PA, et al. Improved 3D phase contrast MRI with off-resonance corrected dual echo VIPR. Magn Reson Med 2008;60(6):1329-36.

10. Sotelo J, Dux-Santoy L, Guala A, et al. 3D axial and circumferential wall shear stress from 4D flow MRI data using a finite element method and a laplacian approach. Magn Reson Med 2017;0:0-0

11. Gu T, Korosec FR, Block WF, et al. PC VIPR: a high-speed 3D phase-contrast method for flow quantification and high-resolution angiography. AJNR Am J Neuroradiol. 2005;26(4):743-9.

Figures

Figure 1.- Steps of the Laplace and centerline quantification process. Is important to note that the 2D planes generated automatically from the Laplace formulation are not overlapping as in the centerline method (ref 10).

Figure 2.- Table1: Patient demographics and MR parameters used to acquire in-vivo data.

Figure 3.- In (a) we show the sixteen regions used to compare the geometrical parameters between volunteer and patient. In (b) we show the description of each geometrical parameters used in this study (b).

Figure 4.- From (a) to (d) we show the results of geometrical parameters with significant differences (p<0.05) between; volunteer/Marfan patients, volunteer/BAV patients, volunteer/BAV_RN patients and volunteer/BAV_RL patients respectively. The yellow box in (a) to (d) represent that exist significant differences between them. In the figure4(e) we show the 3D maps of each geometrical parameters, for one; representative volunteer, patient with Marfan syndrome, patient with BAV-RL and patient with BAV-RN respectively.

Figure 5.- Show the correlation matrix (R2 values), obtained by the linear regression between all geometrical parameters of volunteers, patients with Marfan syndrome, patients with BAV_RN and patients with BAV_RL.

Proc. Intl. Soc. Mag. Reson. Med. 26 (2018)
0761