Purpose

To introduce the ultimate intrinsic transmit efficiency (UITXE) as a performance upper bound for any RF shimming experiment, and propose it as a reference to assess the absolute performance of transmit (Tx) arrays.

Theory and Methods

The transmit efficiency metric η, which was proposed for multiple channel transmission^1,2, is defined as $$$η=\frac{\mathbf{w}^{H}\mathbf{\Gamma}\mathbf{w}}{\mathbf{w}^{H}\mathbf{\Phi}\mathbf{w}}$$$ where $$$\mathbf{w}$$$ is a complex-valued vector of RF shimming coefficients (amplitude and phase modulations) for each Tx channel. The matrix $$$\mathbf{\Gamma=\mathbf{C}^{H}\mathbf{C}}/M$$$ contains the B₁+ squared per unit current for each Tx channel, averaged over the M voxels of the region of interest (ROI) for which Tx efficiency is being maximized. The matrix $$$\mathbf{\Phi}$$$ represents RF power dissipation over the volume (V) of the sample, and its elements are calculated as $$$\mathbf{\Phi}_{n,m}=\frac{1}{2}\intop_{V}\sigma(\mathbf{r})\mathbf{e}^{(n)}(\mathbf{r})^{*}\cdot\mathbf{e}^{(m)}(\mathbf{r})dV $$$ , where $$$\mathbf{e}^{(n)}$$$ is the electric field of the n^th Tx channel per unit current. Note that the matrices $$$\mathbf{\Phi},\mathbf{\Gamma}$$$ are Hermitian by definition and $$$\mathbf{\Phi}$$$ is also a positive definite matrix, in the case of passive media. Hence the maximum Tx efficiency $$$\tilde{\mathbf{\eta}} $$$ is given by the largest eigenvalue of the associated generalized eigenvalue problem $$$\mathbf{\Gamma w}=\lambda\mathbf{\Phi w} $$$. The corresponding eigenvector $$$\tilde{\mathbf{w}} $$$ contains the optimal RF shimming coefficients that achieve maximum efficiency. In this work, we constructed a complete basis of electromagnetic fields using vector spherical harmonic modes (4232 in total), generated by electric currents flowing on a spherical shell (18cm radius), enclosing a homogeneous sphere (15cm radius) with average brain electrical properties³. Since the operator that maps currents to fields is compact, $$$\tilde{\mathbf{\eta}} $$$ converges to the UITXE as the number of modes increases. We investigated UITXE as a function of main magnetic field strength (B₀) and for different target ROIs. We assessed maximum Tx efficiency with respect to UITXE for various arrays of loop coils at 7T. Coils’ electromagnetic fields were calculated with a volume integral equation solver⁴ using piecewise linear basis functions.

Results

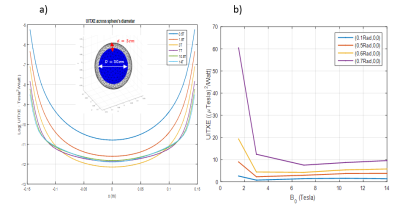
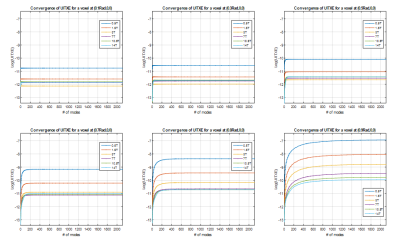
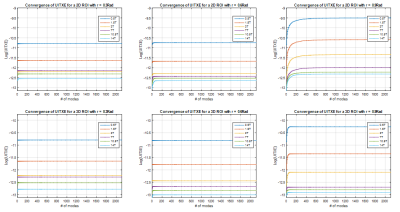
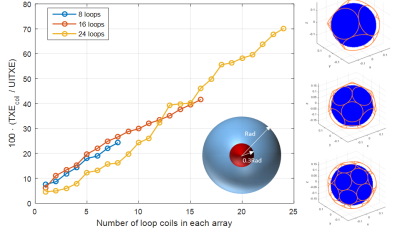
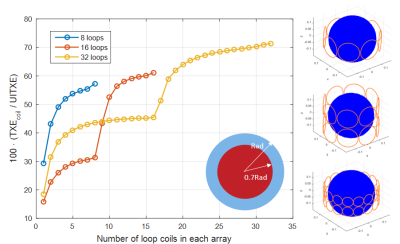
Fig. 1a shows UITXE for single voxels across the sphere’s diameter for different B₀ values, whereas Fig. 1b shows UITXE at particular voxel locations for increasing B₀. As for the UISNR^5,6, UITXE increases as the target voxel approaches the surface of the sphere. Interestingly, UITXE decreases with B₀ up to 3T and then starts to slowly increase for higher B₀ values. Fig. 2 shows convergence of the UITXE calculations vs. number of modes for different voxel positions and B₀. The closer the voxel is to the sphere surface, the more modes are required for convergence. Fig. 3 illustrates the convergence of UITXE when maximizing Tx efficiency in 2D and 3D ROIs of different sizes, rather than at single voxels. UITXE required more modes to converge for 2D than 3D ROIs. Figs. 4 and 5 show the maximum Tx efficiency achievable at 7T with finite arrays as a percentage of the UITXE, for a 3D and a 2D ROI, respectively. In both cases, UITXE was approached more closely by increasing the number of loops. For the 3D ROI (an inner sphere with radius equal to 30% the object radius), enclosing Tx arrays with 8, 16, and 24 loops yielded 24%, 41%, and 70% of the UITXE, respectively. For the 2D ROI (a circle covering 70% the central transverse section), belt shaped Tx arrays with 8, 16, and 32 loops yielded 57%, 61%, and 71% of the UITXE, respectively.

Discussion and Conclusions

UITXE represents a theoretical upper bound for coil performance, since it depends only on the size and electrical properties of the sample and on the target ROI, but it’s independent of any particular coil design. Although the optimization algorithm does not enforce any particular flip angle distribution, as in the case of UISAR³, one can expect that maximizing Tx efficiency would naturally prevent B₁+ nulls within the target ROI². UITXE could be used as an absolute reference to assess Tx arrays and RF shimming techniques, since η is independent of any scale factor in the shimming weights (therefore independent of any overall change in transmit voltage) and, furthermore, can be practically evaluated in experiments². Ongoing future work includes studying UITXE for a realistic human head and combining the current modes using the corresponding optimal shimming coefficients $$$ \tilde{\mathbf{w}} $$$ to derive ideal current patterns⁷ resulting in UITXE, which could provide physical insight and a concrete design target to RF engineers. We also plan to evaluate absolute performance of actual Tx arrays with respect to UITEX in phantom experiments.

Acknowledgements

This work was supported in part by NIH R01 EB024536 and was performed under the rubric of the Center for Advanced Imaging Innovation and Research (CAI2R, www.cai2r.net), a NIBIB Biomedical Technology Resource Center (NIH P41 EB017183).