5558
Motion-corrected high-resolution intra-cardiac imaging using MR-Tracking coils: reducing the effect of noise on motion estimation1Radiology, Brigham and Women's Hospital, Boston, MA, United States, 2Cardiology, Brigham and Women's Hospital, Boston, MA, United States, 3Siemens Healthcare, Boston, MA, United States, 4St. Jude Medical, Minnesota, MN, United States
Synopsis
We developed an intra-cardiac MRI (ICMRI) catheter to monitor heating during MRI-guided electro-physiological ablative procedures. ICMRI includes an imaging coil that expands within the cardiac chambers and a tetrahedral-shaped array of MR-tracking coils, intended to compensate for cardiac motion during the imaging process. In this study, we used real swine cardiac MR-tracking data, which may contain varying levels of positional uncertainty (noise), to develop algorithms that filter this noise, and thus do not distort the motional (translational/rotational) estimates, required for delivering non-blurred high-resolution intra-cardiac images. Our results show that submillimiter-error motion reconstruction is feasible under realistic levels of noise.
Purpose
We developed an advanced generation intra-cardiac MRI (ICMRI)1 [Fig.1] providing higher-Signal-to-Noise-Ratio (SNR) imaging, relative to surface MR coils, of cardiac walls during MRI-guided Electrophysiology radio-frequency ablative (RFA) procedures. The ICMRI imaging coil, which expands from 5 to 35mm diameter, is inserted folded into cardiac chambers through the vascular tree, and then expanded to 35mm at a distance of 30mm from the desired cardiac-wall to enable sensitive (~10x SNR) imaging, which allows faster or higher-spatial-resolution imaging. Since imaging is performed within the moving heart and scan-duration spans multiple cardiac and respiratory cycles, it is imperative to perform motion correction. As a result, ICMRI is equipped with a 15mm diagonal tetrahedron-shaped array of MR-tracking (MRT) coils to estimate rigid-body motion (translations and rotations) and then perform prospective motion correction (PMC) using an interleaved tracking and imaging sequence. We demonstrated2 that when MRT-based motion estimation is accurate, PMC can be successfully performed. However, we have found that when MRT values are noisy, wrong values of translations/rotations are returned, and PMC delivers artifactual images, such as blurred images. The goal of this project was to estimate the effects of such noise on intra-cardiac images, and find methods of MRT data filtration that would improve image quality, in advance of swine experiments to visualize RF ablation of the left ventricle (LV) and left atrium in real-time, which will be performed in November 2016.Methods
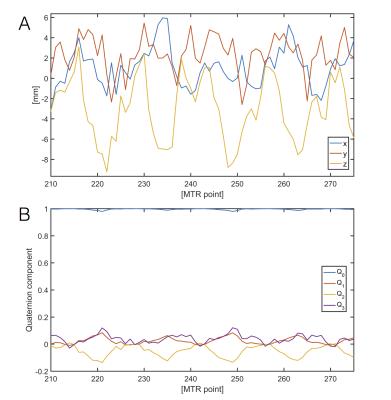
Noisy MRT-based positional-tracking within the heart of a swine. We previously3 measured LV cardiac motion at 40msec increments (during breath-holds) over multiple cardiac cycles by inserting 1-mm diameter catheters with 3 MRT coils into the Left Anterior Descending and Right Coronary Artery vessels in multiple swine. We used data from 2 MRT coils to manufacture an artificial 4-coil tetrahedron array, representing motion of the ICMRI catheter, and followed displacement and rotations over 30 cardiac cycles [Fig.2]. The noise in each MRT coil was estimated to be ~1.2mm using a high-pass filter. Simulations with increasing (0-4mm) uniform distributed noise were used to study the performance of the reconstruction algorithm under varying levels of noise [Fig.3].
Application of filters to improve rotational and translational estimation. We made use of a learning time-period (200 MTR points,~40sec) to measure the dimensions of the extended tetrahedron and the noise level of each coil, so that we could adaptively select rotational and translational noise thresholds. For a system with n coils, our adaptive algorithm provided a set of (n3) rotational estimations and n translational estimations, which were then filtered to reduce the noise effect on the reconstruction. Various filtering strategies (mean, outlier rejection and 1σ filter) were analyzed so that the optimal filter, for a given level of noise, was automatically chosen after the learning period.
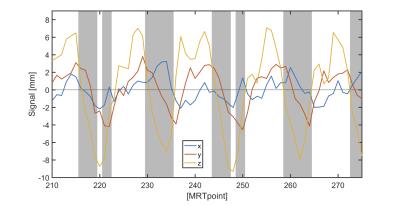
Filter different cardiac phases according to the reconstruction performance. After the optimal rotational and translational estimations were calculated [Fig.4], we compared the MRT input data with the reconstructed position in real time. Time points with a discrepancy in position larger than 1mm could be disregarded, entailing skipping imaging of cardiac-cycle-segments with potentially large artifacts [Fig.5].
Results
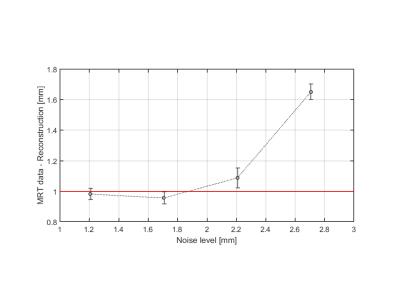
The algorithm was able to reconstruct positions with sub-millimeter accuracy for noise levels up to ~2mm. The ratio of reconstruction error to noise remained below 45% up to ~3mm noise levels. Further increments in noise levels make the error increase in a polynomic fashion.
Using the real (~1.2mm noise) cardiac swine data, we found, in <33% of the cardiac cycle, reconstructed position estimations with more than 1 mm discrepancy from the MRT data.
Discussion and Conclusion
MRT-based motional estimation inside the heart can be improved dramatically by application of noise filtering. Such filters reduced errors in translation estimation and in rotational estimation by 30-40% for noise levels up to 3 mm. We observed that when all coils had the same level of noise (σ), averaging the estimators (mean filter) was a better strategy than rejecting outliers (1σ filter). If one coil was particularly noisy (>2σ), rejecting its data was the most advantageous choice.
Residual errors in the reconstruction remained mainly in the systolic region of the cardiac cycle. Thus, the diastolic phase was a more resilient area, well suited for image acquisition with reduced motion artifacts. The algorithm was robust to errors up to 1mm if the noise is under ~2mm. The larger the noise, the more data samples that needed to be rejected to avoid motion artifacts, increasing the scan time. For a realistic scenario, less than 33% of the cardiac cycle needed to be excluded, so image acquisition could be performed over most of the cardiac cycle.
Acknowledgements
NIH P41EB015898, NIH U54HL119145References
1. Schmidt EJ, Qin L, Santos J, Michaud G, Kwong R, Stevenson W.G, Dumoulin CL. Intra-Cardiac MRI Catheter for EP Ablation Monitoring: Preliminary Studies. The International Society for Magnetic Resonance in Medicine 19th Scientific Meeting & Exhibition, 2011 May, Montréal, Québec, Canada.
2. Qin L, Schmidt EJ, Tse ZT, Santos J, Hoge WS, Tempany-Afdhal C, Butts-Pauly K, Dumoulin CL. Prospective motion correction using tracking coils Magn Reson Med. 2013; 69: 749–759.
3. Schmidt EJ, Yoneyama R, Dumoulin CL, Darrow RD, Klein E, Hayase M. Detailed 3D Coronary Motion Tracking in swine models using MR Tracking catheters. J. Magnetic Resonance Imaging 2009; 29:86-98.
Figures
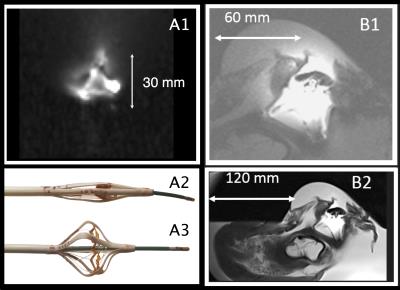
Figure 1: 3T ICMRI imaging and MR-tracking catheter. A) (A1) Tetrahedron 4-channel integrated MRT array on ICMRI shaft, shown in saline phantom. It detects ICMRI motion (translation/rotation) and is used for motion-compensated imaging. (A) Distal portion (A2) closed during vascular navigation, and (A3) expanded to 35 mm diameter during imaging, with an EP RFA catheter protruding out its end. B) ICMRI performance in ex-vivo swine left atrium. ICMRI alone (B1) provides enhanced view of left atrium, with (B2) ~10x higher imaging SNR than provided by a 32-channel surface cardiac array.