4876
Evaluation of the impact of strain correction on the secondary eigenvector of diffusion with in vivo and ex vivo porcine hearts1Cardiovascular BRU, Royal Brompton Hospital, London, United Kingdom, 2NHLBI, National Institutes of Health, MD, United States, 3Department of Radiological Sciences, UCLA, CA, United States, 4Biomedical Engineering, University of Virginia, VA, United States, 5Geisinger Medical Center, PA, United States, 6Siemens Healthcare, GA, United States, 7Siemens Healthcare, TN, United States
Synopsis
Myocytes have a laminar organization, where sheets of myocytes interleave with collagen-lined shear layers. Cardiac diffusion tensor imaging is capable of probing sheet dynamics with secondary diffusion directions, although questions remain about cardiac strain being a possible confounder. Here we study the validity of strain-correcting cardiac diffusion tensor data by directly comparing in vivo DTI data without and with strain correction, to ex vivo DTI data of the same porcine hearts arrested in a diastolic or systolic conformation. Results show that the current strain correction model exaggerates the contribution of microscopic strain to diffusion resulting in an over-correction.
Purpose
To analyse the validity of strain-correcting cardiac diffusion tensor data by directly comparing in vivo and ex vivo data from the same porcine hearts arrested in a diastolic or systolic conformation.Background
Myocytes have a laminar organization, where sheets of myocytes are separated by collagen-lined shear layers. The rotation of these myolaminae, also named sheetlets, plays a major role in explaining wall thickening during cardiac contraction1. Cardiac diffusion tensor imaging (cDTI) with a STEAM sequence is capable of probing sheetlet orientation with the secondary eigenvector of diffusion2, although questions remain about the possible confounding effects of tissue strain throughout the cardiac cycle3 4.
Recent work has shown that strain correction greatly affects the secondary diffusion eigenvector, but no validation of the correction was possible5. Herein, we evaluate the validity of strain-correcting cDTI data by directly comparing in vivo cDTI data without and with strain correction, to ex vivo cDTI data of the same porcine hearts arrested in a diastolic or systolic conformation. The ex vivo hearts provide cDTI data free of any possible cardiac tissue strain during diffusion encoding.
Methods
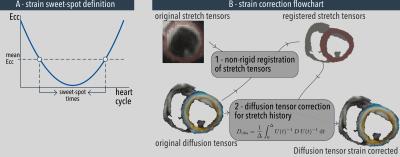
All imaging was performed at 3T (MAGNETOM Skyra, Siemens, Germany) using investigational prototype sequences. 11 pigs were successfully scanned with a multi-slice spiral cine DENSE sequence with displacement encoded in 3D6 (2.5x2.5x8 mm3), and with a STEAM-EPI DTI sequence (6 directions, b=500smm-2, diffusion weighting time 1 cardiac cycle, 2x2x8 mm3) during diastasis, at end systole, and at the strain sweet-spots (see figure 1A for definition) in a mid-ventricular slice. The same cDTI sequence was repeated after the hearts were excised with a diastolic (KCl) arrest induced in n=6 swine and systolic-like (BaCl2) arrest in n=5 swine.
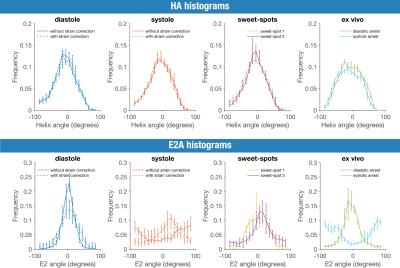
The multi-slice DENSE data was used to calculate the two sweet-spot times of the cardiac cycle where strain effects are minimized (figure 1A), and to strain correct the in vivo cDTI data acquired at end systole and during diastasis on a pixelwise basis (figure 1B). The orientation of the primary (E1A or helix-angle, HA) and secondary (E2A) eigenvectors was compared in diastole (without/with strain correction), in systole (without/with strain correction), at both strain sweet-spots, and in the strain-free ex vivo data arrested in either systole or diastole.
Results
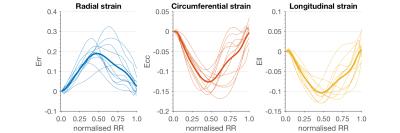
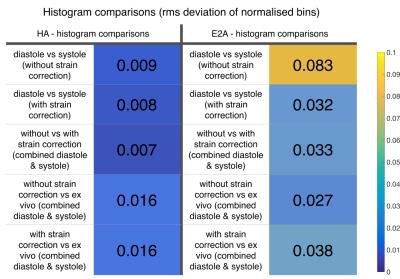
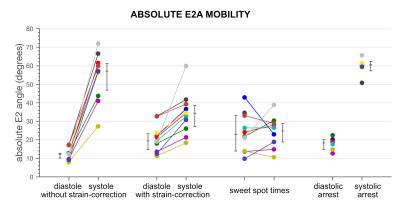
The mean(SD) peak radial, circumferential and longitudinal strains were: Err=0.21(0.06), Ecc=-0.14(0.02), Ell=-0.11(0.01) (figure 2). The sweet-spots given by the Ecc curves were located at 24%(7%) and 74%(12%) of the RR interval time. The inter-subject median [IQR] histograms for HA and E2A are shown in figure 3. The root mean square deviation when comparing the histogram bins of both HA and E2A are shown in figure 4. E2A histogram differences are lower without strain correction (0.027) than with strain correction (0.038) when comparing to ex vivo E2A. The in vivo E2A histograms without strain correction are therefore closer to the ex vivo. The median absolute E2A for each pig is shown in figure 5.Discussion & conclusions
In vivo HA distribution remains relatively unchanged throughout the cardiac cycle, with little impact from strain correction. The ex vivo distribution is slightly broader, but with little difference between the diastolic and the systolic arrests. In contrast, E2A changes across the cardiac cycle and E2A changes with strain correction. E2A differences between diastole and systole are reduced with strain correction. However, ex vivo arrested strain-free E2A conformation is closer to in vivo values without strain correction. These results suggest that the currently proposed strain correction may need to be revisited.Acknowledgements
This work was supported by the following: the National Heart, Lung and Blood Institute, National Institutes of Health by the Division of Intramural Research, NHLBI, NIH, DHHS (HL00460714CPB); the British Heart Foundation; and the National Institute of Health Research Cardiovascular Biomedical Research Unit at the Royal Brompton Hospital and Imperial College, London.References
1 – LeGrice, I. J., Takayama, Y., and Covell, J. W. Transverse shear along myocardial cleavage planes provides a mechanism for normal systolic wall thickening. Circ Res (1995): 182-93.
2 - Kung, G. L., Nguyen, T. C., Itoh, A., et al. The presence of two local myocardial sheet populations confirmed by diffusion tensor MRI and histological validation. J Magn Reson Imaging (2011): 1080-91.
3 - Reese, Wedeen, and Weisskoff Measuring Diffusion in the Presence of Material Strain. J Magn Reson B (1996): 253-8.
4 - Axel, L., Wedeen, V. J., and Ennis, D. B. Probing dynamic myocardial microstructure with cardiac magnetic resonance diffusion tensor imaging. J Cardiovasc Magn Reson (2014): 89.
5 - Stoeck, C. T., Kalinowska, A., von Deuster, C., et al. Dual-phase cardiac diffusion tensor imaging with strain correction. PLoS One (2014): e107159.
6 - Zhong, X., Spottiswoode, B. S., Meyer, C. H., et al. Imaging three-dimensional myocardial mechanics using navigator-gated volumetric spiral cine DENSE MRI. Magn Reson Med (2010): 1089-97.
Figures