3921
Magnetic Field Monitoring of Spiral Echo Train Imaging1Biomedical Engineering, University of Virginia, Charlottesville, VA, United States, 2Physics, Siemens Healthineers GmbH, Erlangen, Germany, 3Radiology & Medical Imaging, University of Virginia, Charlottesville, VA, United States, 4Geisinger Health System, Lewisburg, PA, United States, 5Application Development, Siemens Healthineers GmbH, Erlangen, Germany
Synopsis
The goal of this study was to characterize the k-space trajectories of a spiral echo train pulse sequence using a dynamic field camera. The spiral trajectories were quite repeatable, with typical measured error less than 0.01% of the maximum k-space magnitude. This was true for constant-density, dual-density, and spiral-in/out trajectories. A somewhat larger error was typically observed in the first echo. Overall, this study demonstrates that 3D spiral echo train imaging using the same k-space estimation method for all echoes was sufficient to achieve high image quality.
Introduction:
Echo train imaging (RARE, turbo/fast spin echo) can be combined with spiral readouts for even faster imaging that is robust in the presence of motion. For spiral imaging, it is important to have an accurate estimate of the k-space trajectory, and a number of methods have been developed for estimating the distortion of a prescribed spiral gradient waveform by eddy currents and other hardware factors. Many of these methods focus on spiral gradient echo acquisitions, where the phase introduced by the spiral gradients is relatively isolated between readouts. Spiral echo train imaging is a more complicated case, because errors introduced by the spiral gradients have the potential to build up during the echo train. It is difficult to independently measure the spiral k-space trajectories for each echo using conventional k-space measurement techniques, but an alternative approach is to use magnetic field monitoring with a dynamic field camera. The purpose of this work was to characterize the distortion of spiral trajectories along a spin echo train using a dynamic field camera.Methods:
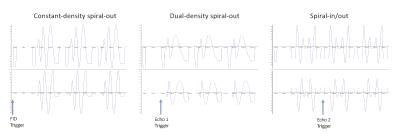
The 3D spiral spin-echo pulse sequence that was characterized was based on a commercial version of a Cartesian 3D echo train pulse sequence (SPACE, Siemens), modified to repetitively acquire spiral gradients in-plane with through-plane phase encoding. The sequence was adapted for use with the field camera by eliminating the RF, ADC and the z-axis gradient waveforms. The experiments were performed on a 3T MAGNETOM Prisma (Siemens) scanner with a dynamic field camera (Skope). The field camera had 16 1H NMR field probes and a 16-channel spectrometer for recording field dynamics. Because of signal decay, the fields associated with only one echo were measured during each TR, and the pulse sequence sent a trigger to the field camera before the relevant echo, which shifted on subsequent TRs (TR=2.5s, 96 echoes plus 1 FID). Three different spiral designs were studied: constant-density spiral-out, dual-density spiral-out, and constant-density spiral-in/out; the first three echoes of each sequence shown in Fig. 1. Each pulse sequence was measured in four orientations: transverse; transverse with X/Y gradients swapped; coronal; and coronal with X/Z gradients swapped.
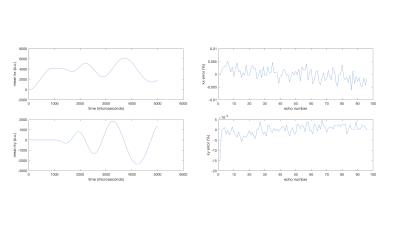
The dynamic field camera measurements were processed in Matlab. The phase of the raw data from each probe was extracted, fit to spherical harmonics, and smoothed to yield (kx, ky, kz) values every microsecond. The mean of the k-space trajectories over all of the echoes was calculated, and average signed and RMS deviation of the k-space trajectory from the mean trajectory was calculated for each echo. This is a measure of the variability over the echo train of the spiral trajectory during the readout. Also, a simulation was performed in which the k-space position was inverted at each refocusing pulse to simulate the evolution of signal phase with ideal refocusing pulses.
Results:
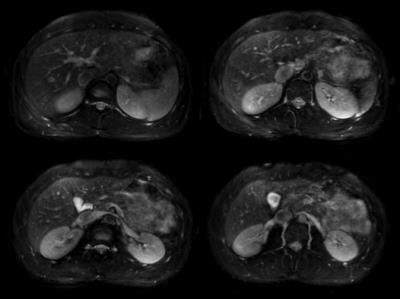
Each type of spiral trajectory was highly repeatable. The largest time-averaged k-space deviation for any echo (other than the first) was less than 0.01% of the maximum k-space magnitude. There were no significant differences between orientations. A typical measured trajectory and the corresponding signed error plot is given in Fig. 2. The errors were larger for many of the first echoes, with the largest error 0.05% of the maximum k-space magnitude. The errors were larger in the simulated echo train, but still on the order of 0.05% and similar to that of a control channel with no gradients applied, so the buildup of errors may be due to the lower SNR at the end of the echo. Figure 3 shows a 3D free-breathing abdominal image with the same k-space estimation method applied for each echo, demonstrating that good image quality can be achieved assuming the same spiral trajectory at each echo.Conclusions:
This study characterized the k-space trajectories of a long spiral echo train pulse sequence using a dynamic field camera. The spiral trajectories were quite repeatable, with typical measured error less than 0.01% of the maximum k-space magnitude. This was true for constant-density, dual-density, and spiral-in/out trajectories. A larger error was typically observed in the first echo, which likely corresponded to an eddy current transient. These errors are probably still small enough to be neglected, but the first echo could be discarded to reduce error further. Future work could involve comparing these errors to those predicted by a gradient impulse response function. Overall, this study demonstrated that this implementation of 3D spiral echo train imaging using the same k-space estimation method for all echoes is sufficient to achieve high image quality.
Acknowledgements
Grant support: NIH R21EB022309References
No reference found.Figures