3845
SOMS: Simultaneous Orthogonal Multi-Slice Imaging1Diagnostische und Interventionelle Radiologie, University Medical Center Göttingen, Göttingen, Germany, 2Partner site Göttingen, German Centre for Cardiovascular Research (DZHK), Göttingen, Germany
Synopsis
Simultaneous multi-slice (SMS) MRI has become a popular technique in both research and clinical practice, as it provides information about multiple slices at the time of a conventional single-slice measurement. For technical reasons, SMS is commonly limited to the acquisition of parallel slices. Here, we propose a new Cartesian sequence for the simultaneous excitation of orthogonal slices. We dub this technique simultaneous orthogonal multi-slice (SOMS). In this proof of concept phantom study, we reconstruct two 2-fold undersampled, simultaneously acquired orthogonal slices.
Introduction and Purpose
The reduction of image acquisition time is crucial for many applications in MRI - especially in clinical practice. Since the turn of the century, simultaneous multi-slice (SMS) has attracted increased interest among researchers as it allows for significantly shorter scan times without essential loss of image quality by simultaneously acquiring data from multiple slices.1,2 However, conventional SMS can only be used to acquire parallel slices. With the newly developed simultaneous orthogonal multi-slice (SOMS) sequence we overcome this limitation and demonstrate the simultaneous acquisition of orthogonal slices which could be interesting for e.g. interventional real-time MRI. The main idea of SOMS is to use the slice-selection gradient of the second slice to phase-encode the first slice and to use the phase-encoding gradient of the second slice as the slice-selection rewinder of the first slice.Methods
Sequence
The
SOMS sequence is an adapted 2D FLASH sequence where two excitation
pulses are used within one TR.
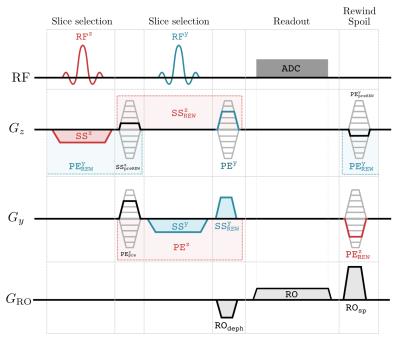
A schematic of the SOMS sequence for orthogonal slices is depicted in Fig. 1. We excite spins orthogonally to the z-axis using a sinc-pulse (RFz) and a slice-select gradient (SSz). To phase-encode (PEz) this slice we use a fixed slice-select (SSy) and slice-select-rewinder (SSyREW) gradient in y-direction, with a variable preceding gradient (PEzpre) which determines the momentum of PEz. The second sinc-pulse (RFy) is applied at the same time as the SSy gradient. This excites a slice perpendicular to the y-axis. To phase-encode this slice, we use the gradient PEy. This gradient, together with the preceding z-gradient SSzpreREW also rewinds the slice-select gradient SSz. Only one readout-dephase (ROdeph) and readout gradient (RO) is necessary. Finally, a readout spoiler (ROsp) and phase rewinders (PEzREW, PEyREW) are used. Note that the momentum of PEyREW is distributed between PEypreREW and the subsequent SSz and SSzpreREW. We make use of the CAIPIRINHA technique by modifying the RF pulse phase.2
Reconstruction
We use a Cartesian SMS-NLINV reconstruction.³ This reconstruction technique jointly estimates the image content and the coil sensitivities for all slices and works particularly well for undersampled SMS data.
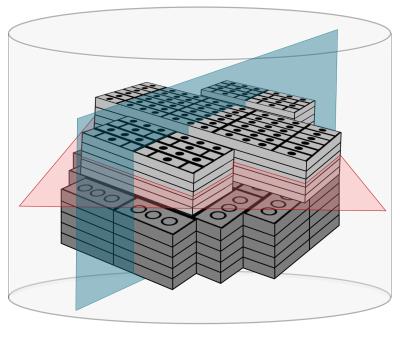
Phantom
The
phantom (Fig.
2) is custom-made using pure water and
Acrylonitrile-Butadiene-Styrene (ABS) bricks known from LEGO. The
proton densities
of the orthogonal slices
apparently differ which
allows for a visual
validation
of the sequence and image
reconstruction.
Experiment
We performed a SOMS measurement of two orthogonal slices. All experiments were conducted on a SIEMENS Skyra 3T scanner using a 20 channel head coil.
Results
Both simultaneously excited orthogonal slices could be reconstructed without significant artifacts (Fig. 3).Discussion
Since we need to apply two RF pulses and corresponding slice-selection gradients, the TR of the SOMS sequence is inherently larger than the TR for a conventional single-slice sequence. However, SOMS only requires one readout and one rephase/spoil event and thus can spare time compared to conventional multi-slice measurements. Moreover, two independent measurements of orthogonal slices can only exploit sensitivity-encoding in one dimension. With SOMS, sensitivity differences between the simultaneously excited slices can be exploited in addition to in-plane variations which enables higher reduction factors, similar to two-dimensional acceleration.4Conclusion and Outlook
In this proof of concept study, we introduced a new sequence for the simultaneous acquisition of orthogonal slices (SOMS). We successfully demonstrated the technique for orthogonal slices with phantom measurement.
Possible next steps are the extension to oblique slices and the combination of SOMS with traditional multi-band excitation pulses from parallel SMS MRI to excite orthogonal sets of multiple parallel slices.
Acknowledgements
No acknowledgement found.References
1. D. Larkman et al. “Use of multicoil arrays for separation of signal from multiple slices simultaneously excited”. J. Magn. Reson. Imaging 13 (2001), pp. 313–317.
2. F. Breuer et al. “Controlled aliasing in parallel imaging results in higher acceleration (CAIPIRINHA) for multi-slice imaging”. Magn. Reson. Med. 53 (2005), pp. 684–691.
3. S. Rosenzweig and M. Uecker. “Reconstruction of multiband MRI data using Regularized Nonlinear Inversion”. In: Proc. Intl. Soc. Mag. Reson. Med 24 (2016), p. 3259.
4. M. Weiger et al. “2D sense for faster 3D MRI”. Magn. Reson. Mater. Phys., Biol. Med. 14 (2002), pp. 10–19.
Figures