3389
Analytical solution for restricted diffusion in multilayered cylinders using the extended Multiple Correlation Function approach.1I2BM / Neurospin / UNIRS, CEA, Gif-sur-Yvette, France, 2Université Paris-Saclay, Orsay, France, 3I2BM / Neurospin / UNATI, CEA, Gif-sur-Yvette, France
Synopsis
In this work, we used the extended Multiple Correlation Function (MCF) method to derive analytical expressions of the NMR signal in multilayered cylinder geometries for an arbitrary direction of the magnetic field gradient. Each layer of the cylinder is characterized by a diffusion coefficient and a relaxation time and each boundary between adjacent layers is characterized by a value of permeability in order to allow the modeling of the multilayered structure of axons surrounded by its myelin sheat.
Purpose
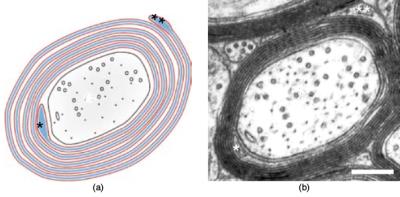
Diffusion MR microscopy has become an alternative to histology to probe in vivo quantitative features of the white matter microstructure, such as the local mean axon diameter and density. Identifying the appropriate model is critical to providing reliable and quantitative information. Many degrees of freedom are needed in brain white matter multi-compartmental models in order to capture diffusion effects1. However, in state-of-the-art models2,3, a simple cylinder geometry is used to represent the restricted compartment of water trapped within axons. Multilayered cylinders would better represent the axon structure (Fig2). Analytical solutions for restricted diffusion in multilayered cylinders have been provided using the powerful MCF formalism4. However, those solutions are only valid for a fixed direction of the magnetic gradient waveform, which does not allow to incorporate the effects of imaging gradients in diffusion-weighted NMR scans. To alleviate this limitation, the MCF technique was extended by allowing for variations in the direction of the gradient5, but no analytical solution was provided for permeable multilayered cylinders. The aim of this theoretical work is thus to compute this analytical solution using the extended MCF approach.Methods
Laplace operator eigenbasis
The solution to the Bloch-Torrey equations in multilayered cylinders (Fig1) in terms of eigenfunctions $$$ u_{nk}( r, \phi ) $$$ of the Laplace operator is calculated using polar coordinates, enabling the separation between radial and angular components : $$ u_{ nk }( r, \Phi ) = C_{ nk }R_{ nk }( r )\Phi_{ nk }( \phi ) $$ The computation of Laplace operator eigenfunctions and of their corresponding eigenvalues does not depend on the gradient direction. The computation of the eigenfunctions radial part $$$ R_{ nk }( r ) $$$ and of the corresponding eigenvalues was provided with specific diffusion coefficient, relaxation times $$$T_2$$$ per layer and allowing different permeabilities at each interface4. However, the angular part is different and takes the form $$$ \Phi_{nk}( \phi ) = e^{i n\phi }$$$ as we need to express the generalized case of an arbitrary gradient orientation. The normalization constant $$$C_{ nk }$$$ is set to fulfill $$ C_{ nk }^2\int_{r_0}^R\int_{0}^{2\pi}{ | u_{ nk }( r, \phi ) |^2 r dr d\phi } = 1$$ We thus obtain $$ u_{ nk }( r, \phi ) = \frac{1}{\sqrt{ 2\pi(R^2-r_0^2)I_{nk} }} R_{nk}(r)e^{i n \phi}$$ where the integral $$$I_{nk} = \frac{1}{(R^2-r_0^2)}\int_{r_0}^R{R_{nk}(r)^2 r dr}$$$ has already been computed4.
Magnetic field matrix
The reconstruction of the macroscopic signal using MCF necessitates the computation of the magnetic field matrix $$$A $$$ corresponding to a linear gradient $$$ \textbf{ r } $$$. In standard MCF approach, the linear gradient is oriented along the $$$x$$$-axis. Thus, only $$$\mathcal{ A }^x$$$ is computed . Following Bar and Sochen 6, we computed the $$$y$$$-component $$$\mathcal{ A }^y$$$ which generalizes the multilayered cylinder solution of Bloch-Torrey equation to any gradient direction.
Results & Discussion
The $$$\mathcal{ A }^x$$$ component is given by $$\mathcal{ A }^x_{nk, n'k'} = \int_{r_0}^R\int_{0}^{2\pi}{ u_{nk}( r )u_{n'k'}^*( r ) r^2 \cos{\phi} dr d\phi }$$ and the $$$\mathcal{ A }^y$$$ component is given by $$\mathcal{ A }^y_{nk, n'k'} = \int_{r_0}^R\int_{0}^{2\pi}{ u_{nk}( r )u_{n'k'}^*( r ) r^2 \sin{\phi} dr d\phi }$$ For both $$$x$$$ and $$$y$$$ components, the radial integration writes $$ \int_{r_0}^R{ r^2 R_{nk}( r )R_{n'k'}(r) dr } = R( R^2 - r_0^2 )K_{nk, n'k'} $$ where the expression of $$$K_{nk, n'k'} $$$ has already been derived 4. For the $$$x$$$ component, integration over $$$\phi$$$ yields $$\int_0^{2\pi}{ e^{i (n – n' ) \phi} \cos{\phi} d\phi } = \pi\delta_{n', n \pm 1}$$ while for the $$$y$$$ component, $$ \int_0^{2\pi}{ e^{i (n – n' ) \phi} \sin{\phi} d\phi} = i\pi[\delta_{n', n – 1} - \delta_{n', n + 1}] $$ We finally get $$\mathcal{ A }^x_{nk, n'k'} = \pi \delta_{n', n \pm 1}R( R^2 - r_0^2 )K_{nk, n'k'}$$ and $$\mathcal{ A }^y_{nk, n'k'} = i\pi[\delta_{n', n – 1} - \delta_{n', n + 1}]R( R^2 - r_0^2 )K_{nk, n'k'}$$ We have thus computed the magnetic field matrix $$$A = (\mathcal{ A }^x, \mathcal{ A }^y, 0) $$$. The present work shows that it is possible to generalize solutions of Bloch-Torrey equations for multilayered geometries to the case of arbitrary gradient directions. The same framework could be used in other multilayered geometries to obtain more accurate analytical solution of the diffusion inside cellular structures in white matter.Conclusion
We established solutions of the Bloch-Torrey equations for a multilayered cylinder structure with specific diffusion coefficient, relaxation times $$$T_2$$$ per layer and allowing different permeabilities at each interface. This model is particularly adequate to represent the microstructure of white matter fibers made up of an axon surrounded by its myelin sheat, and could be used to integrate the two components at the same time and to allow different permeabilities at their interfaces.Acknowledgements
This work was partially funded by the European FET Flagship ‘Human Brain Project’ (SP2) FP7-ICT-2013-FET-F/604102.References
1. Panagiotaki et al. Compartment models of the diffusion MR signal in brain white matter: A taxonomy and comparison. NeuroImage 2012; 59: 2241-54.
2. Alexander et al. Orientationally invariant indices of axon diameter and density from diffusion MRI. Neuroimage 2010; 52(4): 1374-89.
3. Assaf et al. Axcaliber: A method for measuring axon diameter distribution from diffusion MRI. Magn. Res. in Med. 2008; 59: 1347-54.
4. Grebenkov. Pulsed-gradient spin-echo monitoring of restricted diffusion in multilayered structures. Journal of Magn. Res. 2010; 205: 181-195.
5. Özarslan, Shemesh, Basser. A general framework to quantify the effect of restricted diffusion on the NMR signal with applications to double pulsed field gradient NMR experiments. The Journal of Chem. Phys. 2009; 130 104702.
6. Bar, Sochen. A Spectral Framework for NMR Signal with Restricted Diffusion. Concepts in Magn. Res. 2015; 44A(1) 16-53.
7. Johansen-Berg, E.J. Behrens. Diffusion-MRI: From quantitative measurement to in-vivo neuroanatomy. Elsevier 2009.
Figures
