3353
Eddy current artifact reduction in diffusion-weighted single-refocused spin-echo EPI1Brain Imaging Center (BIC), Goethe University Frankfurt, Frankfurt am Main, Germany, 2Palacky University Olomouc, University Hospital Olomouc, Czech Republic
Synopsis
Diffusion-weighted (DW) MRI with single-refocused spin-echo preparation suffers from eddy-current induced image distortions. In this study, a method for substantial reduction of eddy-current artifacts is proposed. Dummy scans comprising DW gradients prior to the acquisition of each multi-slice data volume yield a steady state of eddy-currents and thus comparable distortions across the volume which subsequently can be corrected via an advanced setting of the eddy-current correction FMRIB software library tool. In comparison to the commonly used twice-refocused spin-echo sequence for eddy-current compensation, the proposed method is less sensitive to radiofrequency inhomogeneities and offers higher signal-to-noise ratio due to shorter echo-time.
Purpose
Diffusion-weighted (DW) MRI with single-refocused spin-echo (srSE) preparation is based on the Stejskal-Tanner concept [1] (Fig.1a, top gradient scheme). Eddy currents induced by the DW gradients may yield image distortions that depend on the DW gradient direction [2]. A compensation method has been proposed, employing bipolar DW gradients between the radiofrequency (RF) pulses [3-4] (Fig.1a, bottom gradient scheme). However, this method yields a reduced diffusion time (Δ) and signal-to-noise ratio (SNR) losses due to a prolonged echo-time (TE), especially for short echo-train-lengths. Similarly, a twice-refocused spin-echo (trSE) module [5] with bipolar DW gradients was proposed (Fig.1b) which also prolongs TE and is more sensitive to RF inhomogeneities (B1) due to the additional refocusing pulse. These issues become more severe at higher magnetic field strengths. The current study proposes an improved method for a substantial reduction of eddy-current artifacts. It is based on srSE-EPI with monopolar DW gradients (Fig.1a, top gradient scheme), thus achieving short TE. Eddy-current artifact compensation comprises (1) dummy scans and (2) an advanced setting for the eddy-current correction FMRIB software library (FSL) tool [6-7]. The method is compared to DW-trSE.Methods
The proposed method employs standard srSE-EPI. Dummy scans (no RF but full DW gradients) of duration Tds are played out prior to the acquisition of each multi-slice data volume, using the same DW gradient directions in the dummy scans and the acquisition. This drives eddy currents into a steady state prior to acquisition, thus reducing artifacts (Nyquist ghosting, blurring) and avoiding inter-slice differences between eddy-current induced distortions which therefore can be corrected via postprocessing with an advanced FSL-toolbox.
Experiments: On a healthy volunteer, diffusion tensor imaging (DTI) with b = 1000 s/mm2 and 60 different DW-directions was performed, using the optimized DW-srSE-EPI and DW-trSE-EPI sequences on a 3T MRI-scanner with an 8-channel phased array head receive coil. Parameters were: Field-of-view = 192×192 mm2, in-plane spatial resolution = 2×2 mm2, 60 interleaved axial slices, thickness 2 mm without gap, partial-Fourier = 6/8, GRAPPA-acceleration-factor = 2, echo-spacing Tes = 0.86 ms, Bandwidth (BW) = 1302 Hz/pixel, TR/volume = 9 s, and TE = 81/95 ms (srSE/ trSE). For Tds, the values 0/0.75/1.5/3 s were tested in srSE.
Image analysis: Preprocessing and subsequent analysis were performed using FMRIB's diffusion toolbox [6-7]. Initially, geometrical distortion caused by off-resonance effects was corrected with additional reversed phase-blip data using TOPUP [7-8]. DW-raw-images were corrected for eddy-current and head-motion using either (1) affine registration to a reference magnetic field B0 volume [9] or (2) iterative registration to a model-free prediction of an undistorted image calculated via a Gaussian process implemented in EDDY [10]. In order to achieve optimal eddy-current corrections, the second pipeline was employed with the first-level cubic model, the second-level linear model and 20 iterations. Diffusion tensor fitting was performed using linear regression implemented in the “dtifit” FSL-tool for computing fractional anisotropy (FA) maps and principal eigenvectors.
Results and Discussion
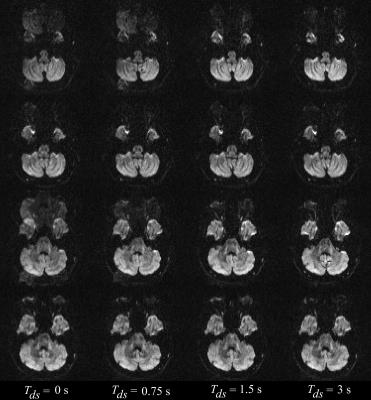
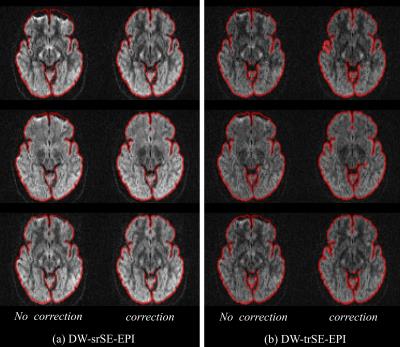
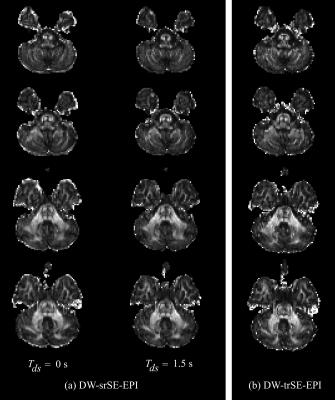
For Tds=0, the proposed sequence yielded eddy-current induced artifacts such as misalignment, signal discrepancy, blurring and Nyquist ghosting, in particular for even-numbered slices (Fig.2, left column: rows 1&3). This is due to interleaved sampling as even-numbered slices are acquired first, i.e. before eddy currents have attained a steady state, so distortions are variable across these slices and differ from those of odd-numbered slices (Fig.2, left column: rows 2&4). DW-srSE preparation reduced eddy-current generated Nyquist ghosting, blurring, and signal discrepancy with increasing Tds (Fig.2), being sufficient for Tds=1.5s (Fig.2, 3rd column: rows 1&3). Image distortions due to residual eddy-currents and additional B0 inhomogeneities differ in different diffusion-directions (Fig.3a, left). In contrast, eddy-current compensated DW-trSE-EPI suffers only from B0 inhomogeneities (Fig.3b, left). After post-processing, both methods yield undistorted images (Fig.3a,b, right), albeit with reduced SNR for DW-trSE-EPI due to the longer TE. Eddy-current artifacts as shown in Fig.2 also influence FA maps and cannot be removed during postprocessing. Fig.4 shows FA maps acquired with the DW-srSE sequence (Tds of 0 and 1.5 s) and the DW-trSE sequence. For Tds=0, eddy-current induced artifacts, e.g., bright edges, blurring and misalignment, are clearly visible in the temporal lobe of the brain (Fig.4a, left). For the other data sets, FA maps provide better quality (Fig.4, center&right).Conclusion
An improved method for substantial reduction of eddy-current induced artifacts is proposed, using an optimized Stejskal-Tanner preparation with a sufficient number of dummy scans and an advanced setting of the eddy-current FSL tool (i.e. EDDY). In comparison to DW-trSE, the proposed method is less sensitive to B1 inhomogeneities and offers higher SNR due to shorter TE.Acknowledgements
No acknowledgement found.References
[1] Stejskal, E. O., & Tanner, J. E. (1965). Spin diffusion measurements: spin echoes in the presence of a time-dependent field gradient. The journal of chemical physics, 42(1), 288-292.
[2] Le Bihan, D., Poupon, C., Amadon, A., & Lethimonnier, F. (2006). Artifacts and pitfalls in diffusion MRI. Journal of magnetic resonance imaging, 24(3), 478-488.
[3] Alexander, A. L., Tsuruda, J. S., & Parker, D. L. (1997). Elimination of eddy current artifacts in diffusion-weighted echo-planar images: the use of bipolar gradients. Magnetic Resonance in Medicine, 38(6), 1016-1021.
[4] Finsterbusch, J. (2009). Eddy-current compensated diffusion weighting with a single refocusing RF pulse. Magnetic resonance in medicine, 61(3), 748-754.
[5] Reese, T. G., Heid, O., Weisskoff, R. M., & Wedeen, V. J. (2003). Reduction of eddy-current-induced distortion in diffusion MRI using a twice-refocused spin echo. Magnetic Resonance in Medicine, 49(1), 177-182.
[6] Jenkinson, M., Beckmann, C.F., Behrens, T.E.J., Woolrich, M.W., Smith, S.M. (2012). FSL. NeuroImage 62, 782–790. doi:10.1016/j.neuroimage.2011.09.015.
[7] Smith, S. M., Jenkinson, M., Woolrich, M. W., Beckmann, C. F., Behrens, T. E., Johansen-Berg, H., ... & Niazy, R. K. (2004). Advances in functional and structural MR image analysis and implementation as FSL. Neuroimage, 23, S208-S219.
[8] Andersson, J.L.R., Skare, S., Ashburner, J. (2003). How to correct susceptibility distortions in spin-echo echo-planar images: application to diffusion tensor imaging. NeuroImage 20, 870–888. doi:10.1016/S1053-8119(03)00336-7.
[9] Jenkinson, M., Bannister, P., Brady, M., Smith, S. (2002). Improved Optimization for the Robust and Accurate Linear Registration and Motion Correction of Brain Images. NeuroImage 17, 825–841. doi:10.1006/nimg.2002.1132.
[10] Andersson, J.L.R., Sotiropoulos, S.N. (2016). An integrated approach to correction for off-resonance effects and subject movement in diffusion MR imaging. NeuroImage 125, 1063–1078. doi:10.1016/j.neuroimage.2015.10.019.
Figures