1434

Inversion quality independent robust $$$T_1$$$-quantification of MOLLI sequence data

Thomas Kampf¹, Theresa Reiter², and Wolfgang Rudolf Bauer³

¹Experimental Physics V, Universität Wuerzburg, Wuerzburg, Germany, ²University Hospital Wuerzburg, ³University Hosptial Wuerzburg

Synopsis

Quantitative mapping of the longitudinal relaxation time has gained increasing interest as it allows monitoring of important structural and functional information of the myocardium. The MOLLI sequence commonly used in clinical research requires a high quality of the inversion pulses for unbiased quantification which is non-trivial especially at high fields. In this work we present a simple modification of the MOLLI sequence which in combination with the recently introduced IGF post processing solves the problem of insufficient inversion quality as demonstrated in phantom experiments.

Introduction

Quantification of functional and structural properties, e.g. perfusion or extra cellular volume, has gained increasing interest in clinical research. Especially,research focuses on quantification of the longitudinal relaxation $$$T_1$$$ time.On clinical scanners usually MOLLI, a multi shot version of an inversion recovery Look-Locker sequence, is used. However, with this sequence the magnetization only recovers with an apparent relaxation time $$$T_1^*$$$. To estimate $$$T_1$$$ usually the correction$$$^1$$$ $$$T_1 = T_1^*\, M_0/M_{ss}$$$ ($$$M_0$$$-equilibrium magnetization, $$$M_{ss}$$$-steady state magnetization) is applied. While the quality of $$$T_1^*$$$ and $$$M_{ss}$$$ is usually of no concern, the estimate of $$$M_0$$$ depends strongly on the quality of the inversion pulse which can cause problems especially at higher fields$$$^2$$$. To circumvent this problem the quality of the inversion pulse is calculated from signal models or measured in a pre-experiment. Recently the Inversion Group Fitting (IGF) method for $$$T_1$$$ quantification from MOLLI data was introduced$$$^{3,4}$$$. It allows to determine $$$M_0$$$, $$$M_{ss}$$$ and $$$T_1^*$$$ from MOLLI sequences with arbitrary waiting times between the inversions. This can be used to incorporate the M0measurement directly in the MOLLI sequence and hence obtain a more robust quantification of $$$T_1$$$.

Methods

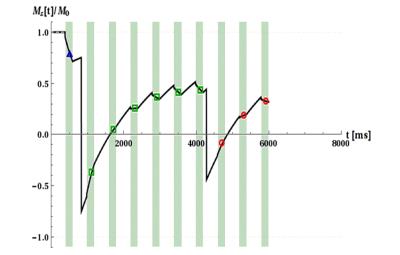
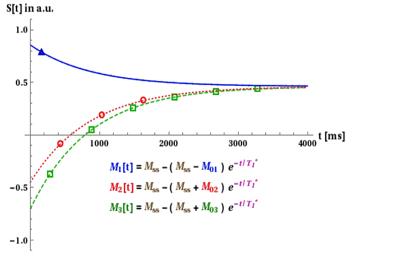
Recently the IGF was introduced to overcome the dependence of MOLLI based $$$T_1$$$-data from heart rate and waiting time. It bases on the insight that for a constant heart rate the apparent relaxation time $$$T_1^*$$$ as well as the steady state magnetization $$$M_{ss}$$$ is equal for all inversions. Only the initial magnetization differs for the different inversions. Furthermore, before the first inversion pulse the magnetization is in equilibrium. Hence, $$$M_0$$$ can be determined from the first inversion. However, the quality of this value depends on the quality of the inversion pulse. In this work it is proposed to acquire an additional image before the first inversion to determine $$$M_0$$$. In the next heart beat without additional waiting time a usual MOLLI sequence with multiple inversions can be used (see Fig. 1). From the inversion data the IGF method is used to obtain $$$T_1^*$$$ and $$$M_{ss}$$$. As the first image is acquired without inversion pulse the magnetization $$$M_1(t_1)$$$ at the echo time $$$t_1$$$ is independent from its quality. With the assumed monoexponential signal evolution $$$M_0$$$ is extrapolated (see Fig. 2) and the relaxation time can be estimated via

$$T_1=\frac{M_1(t_1)}{M_{ss}} T_1^* - t_1 + \frac{M_1(t_1) t_1}{M_{ss}}\,.$$

All measurements were performed at a 3 T clinical MR scanner with vendor provided sequences. A saturation recovery spin echo with multiple delay times was used as reference. Different MOLLI schemes were tested. The notation $$$x_1(y_1)x_2(y_2)…$$$ where $$$x_n$$$ gives the number of images in the $$$n$$$-th inversion and $$$y_n$$$ is the waiting time in heart beats between $$$n$$$-th and $$$(n+1)$$$-th inversion. The Phantom consists of multiple agarose gel filled tubes (2.5% agarose) doped with Gd-based contrast agent of different concentration. The corresponding $$$T_1$$$-times ranged from approximately 220ms to 2700 ms.

Results

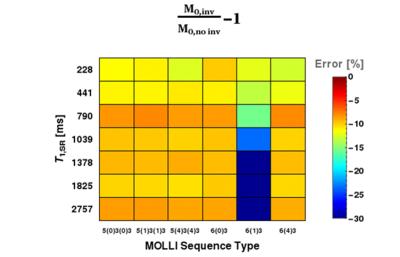
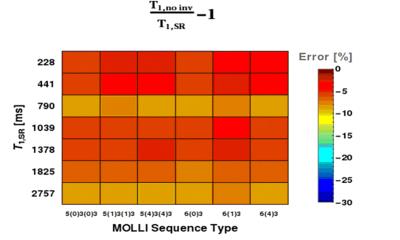
The experimental data show a strong underestimation of the $$$T_1$$$ quantified from the MOLLI data of approximately 15-17% (see Fig. 3). Furthermore for the 6(1)3 sequence even larger errors are found. Fig.4 shows that a large part of this bias (approximately 10% of the underestimation) comes from an underestimation of $$$M_0$$$ from the inversion data compared to the $$$M_0$$$ value determined from non-inversion data of the first image. The increased error in the 6(1)3 sequence is caused by especially large underestimation of $$$M_0$$$. Using the proposed correction and the non-inversion data a significant reduction in the in the $$$T_1$$$ bias is achieved (approximately 5-7%) (see Fig.5). Furthermore the larger deviations for 6(1)3 are removed.

Discussion and Conclusion

In this work a simple strategy for the reduction of $$$T_1$$$-quantification dependency on the quality of the inversion pulse is demonstrated. It circumvents the problem by calculating the equilibrium magnetization from a single image before the first inversion pulse. Hence, the bias due to imperfect inversions could be significantly reduced. This comes to the expense of an additional image which increases the sequence length by a single heartbeat. Compared to usual sequence lengths of 11-17 heart beats this is only a minor drawback. Additionally the $M_0$ value is not determined from a curve fit but calculated from a single image. Hence, high SNR in the initial image is necessary to prevent increased noise in $$$T_1$$$ which might exceed the bias introduced by insufficient inversion. However, one has to keep in mind that this image has the highest SNR as it is acquired starting with equilibrium magnetization and does not suffer from insufficient recovery times which reduce SNR.

Acknowledgements

None

References

1. Deichmann R, Haase A, Quantification of $$$T_1$$$ values by SNAPSHOTFLASHNMR imaging. J MagnReson, 1992;96: 608-612.

2. Kellman P,HerzkaDA, Hansen MS,Adiabatic inversion pulses for myocardial $$$T_1$$$ mapping.MagnReson Med,2014: 71 (4): 1428-1434

3. Reiter T, Kampf T. Bauer WR, Myocardial Fibrosis and extracellular Volume in DCM -clinical application of an improved post processing strategy of MOLLI based $$$T_1$$$ quantification, 80-th Annual meeting of the German Cardiac Society, 2014: V847

4. Sussman MS, Yang IY, Fok K et al., Inversion group (IG) fitting: A new $$$T_1$$$ mapping method for modified look-locker inversion recovery (MOLLI) that allows arbitrary inversion groupings and rest periods (including no rest period). MagnReson Med, 2016: 75 (6) 2332-2340

Figures

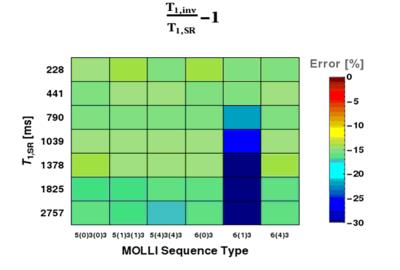
Scheme of the proposed method. Starting before the first inversion pulse an additional initial image is acquired (blue triangle). Afterwards, without additional waiting time a standard MOLLI sequence can be applied (here 6(0)3). Where the first inversion train (green boxes) and the second inversion train (red circles) both do not start from $$$M_0$$$ but will have the same $$$M_{ss}$$$ and $$$T_1$$$

Visualization of the adapted post processing. Using the IGF fitting procedure one can obtain $$$T_1^*$$$ as well as $$$M_{ss}$$$ from the second (green boxes) and third (red circles). Using these estimates $$$M_0$$$ can be calculated from the first pre inversion image (blue triangle).

in the quantified longitudinal relaxation times form MOLLI sequences without initial image before the first inversion $$$T_{1,inv}$$$ compared to the reference $$$T_{1,SR}$$$. A large underestimation nearly constant of 15-17% is observable. For 6(1)3 MOLLI an increased deviation of $$$T_{1,inv}$$$ is observed.

Deviation of the equilibrium magnetization value between fitted value from the MOLLI data without initial pre-inversion image $$$M_{0,inv}$$$ and the equilibrium magnetization calculated using the proposed method $$$M_{0,no inv}$$$. A nearly constant underestimation of the equilibrium magnetization calculated from the data without initial pre-inversion image is observable. Furthermore, an increased underestimation of $$$M_0$$$ is observed for 6(1)3 explaining the increased $$$T_1$$$-bias.

Error in the quantified $$$T_{1,no inv}$$$ values from the MOLLI sequence with initial pre inversion image. A significant reduced $$$T_1$$$ bias (5-7%) compared to the standard post processing is observable. No increased bias of the quantified $$$T_{1,no inv}$$$ value is observed for 6(1)3 MOLLI.

Proc. Intl. Soc. Mag. Reson. Med. 25 (2017)

1434