0717
Pore size estimation using the mixing time dependence of a double diffusion encoding experiment: experimental validation on a clinical MR system1University of Luebeck, Luebeck, Germany
Synopsis
Diffusion MRI provides information about microstructure, but is limited in complex situations. Double diffusion encoding makes assessment of shape and size possible in these contexts. The time delay (mixing time) between diffusion encodings has not been studied closely outside of the short and long regimes. We present here an experimental study of the mixing time dependence. In spherical pores, the parallel-antiparallel signal difference can be approximately described as an exponential decay with a rate related to diameter. This decay was obtained on a clinical scanner in a water-in-oil emulsion.
Purpose
To explore the mixing time dependence of the double diffusion experiment as a way to estimate microscopic pore size.Introduction
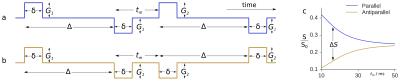
Conventional diffusion MRI single diffusion encoding (SDE), has proved helpful in measuring restricted diffusion in voxels containing aligned structures like white matter. Double diffusion encoding (DDE) is better adapted to voxels containing randomly oriented structures.1-2 DDE offers more possibilities in experiment design than SDE (see parameters in Figure 1). By varying the angle between the two wave-vectors,3-4 their amplitude5 or both,6-7 the diameter of differently shaped pores can be estimated, especially when using clinical systems with limited gradient strength.8
Here, we introduce the mixing time (tm) dependence, as measured in both parallel and anti-parallel cases (Figure 1a and 1b, respectively), as a tool to estimate spherical pore size.9 We present here first experimental results using a clinical MR system and a water-in-oil emulsion.10
Methods
The emulsion consists of water droplets colored with methylene blue and surrounded by oil.10 Its mean droplet diameter, as measured by light microscopy and weighted for volume contribution, was approximately 8 µm. A 3 T MR whole-body system (Ingenia, Philips, Amsterdam, Netherlands) was used with 45 mT/m maximum gradient strength and an 8-channel head coil. SPIR fat saturation was used in conjunction with a spin-echo EPI implementation of DDE.11 A 27x27x9 mm³ region of interest centered in the phantom was averaged for analysis. Table 1 shows experimental parameters. All wave-vectors were constrained in the x-y plane (perpendicular to the main magnetic field). φ is the angle formed by the first wave-vector with the x axis. ψ is the angle between the two wave-vectors. The phantom, a 250 ml plastic bottle, was aligned along the horizontal z axis. Total acquisition time was 4 hours 44 minutes.
To correct for background gradients, eight evenly distributed directions were used for the first wave-vector. Signals from opposite directions were then geometrically averaged.12 Pore diameter, d, was extracted from the normalized parallel-antiparallel signal difference (ΔS, see Figure 1c) dependence on tm using:9
ΔS∝ e−4α2D0tm/d2
, with α ≈ 2.08, a geometrical factor. A value of 2.3 µm²/ms was used for the diffusion coefficient, D0, at 300 K.13
Results
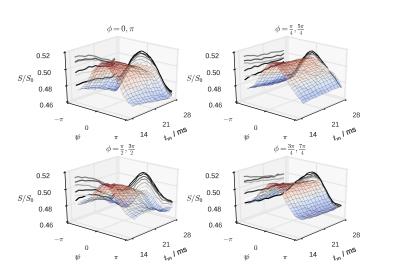
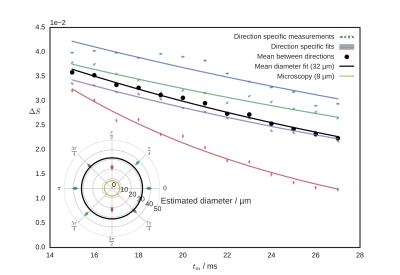
Figure 2 shows the signal modulation with Ψ for increasing tm. A clear difference is present across values of φ. Figure 3 shows the decay of ΔS with increasing tm for all directions and for their arithmetic mean. The inset shows diameter estimate for individual directions compared with their mean (averaged before fit) and with the light microscopy measurements.Discussion
Since the water droplets in the emulsion phantom are spherical, the results should be independent of the direction of the first wave-vector. This is not the case, as can be seen on Figure 2 and can originate from eddy current effects.14 The general decay with tm is nonetheless present for every assessed direction of the first wave-vector.
The large deviation between the pore diameter estimate from microscopy (8 µm) and the tm fit (32 µm) may be due to:
- The experiment does not respect the long diffusion wavelength condition (γδGd/2)2≪1 because of the presence of big pores.
- The emulsion requires the use of surfactants, this may modify the diffusion coefficient.
- Eddy current or susceptibility effects.
- Signal modulation from other sources. Cross term gradients may originate from eddy currents and susceptibility effects.
Even if DDE has been demonstrated not to contain, under many circumstances, new information compared to the set of SDE experiments,15 it may provide a more elegant and clinically feasible way to estimate pore size.
Conclusion
The present work describes the use of the mixing time dependence of DDE MRI as a source of spherical pore size information. A decay of the parallel-antiparallel signal difference with increasing tm was observed. In future development, it is hypothesized that this decay contains information about the complete pore size distribution, which could then be retrieve by means of an inverse Laplace transform analogue.9
The data presented here span 8x32 direction pairs as a way to identify artifacts, when only 2x2 should be necessary for diameter estimate. Such a subset would require around five minutes of acquisition time.
Acknowledgements
We thank the German Research Foundation [grant DFG KO3389/2-1] for financial support.References
1. Mitra, P. P. (1995). Multiple wave vector extension of the NMR pulsed gradient spin echo diffusion measurement. Phys. Rev. B 51, 15074–15078. http://doi.org/10.1103/PhysRevB.51.15074
2. Özarslan, E., & Basser, P. J. (2008). Microscopic anisotropy revealed by NMR double pulsed field gradient experiments with arbitrary timing parameters. J. Chem. Phys. 128, 154511. http://doi.org/10.1063/1.2905765
3. Koch, M. A., & Finsterbusch, J. (2008). Compartment size estimation with double wave vector diffusion-weighted imaging. Magn. Reson. Med. 60, 90–101. http://doi.org/10.1002/mrm.21514
4. Morozov, D., Bar, L., Sochen, N., & Cohen, Y. (2013). Measuring small compartments with relatively weak gradients by angular double-pulsed-field-gradient NMR. Magn. Reson. Imaging 31, 401–407. http://dx.doi.org/10.1016/j.mri.2012.08.007
5. Shemesh, N., Ozarslan, E., Basser, P. J., & Cohen, Y. (2010). Detecting diffusion-diffraction patterns in size distribution phantoms using double-pulsed field gradient NMR: Theory and experiments. J. Chem. Phys. 132, 034703. http://doi.org/10.1063/1.3285299
6. Benjamini, D., Katz, Y., & Nevo, U. (2012). A proposed 2D framework for estimation of pore size distribution by double pulsed field gradient NMR. J. Chem. Phys., 137, 224201. http://doi.org/10.1063/1.4769792
7. Benjamini, D., & Nevo, U. (2013). Estimation of pore size distribution using concentric double pulsed-field gradient NMR. J. Magn. Reson. 230, 198–204. http://doi.org/10.1016/j.jmr.2013.03.001
8. Koch, M. A., & Finsterbusch, J. (2011). Towards compartment size estimation in vivo based on double wave vector diffusion weighting. NMR Biomed. 24, 1422–1432. http://doi.org/10.1002/nbm.1711
9. Methot, V. & Koch, M. A. (2016). Pore size distribution estimation using the mixing time dependency of a double diffusion encoding experiment: a proof of concept from Monte Carlo simulated data. Magn. Reson. Mater. Phy. 29, S165–S167. http://doi.org/10.1007/s10334-016-0569-9
10. Ulloa, P., Benedyk, A., Landmesser, R. & Koch, M. A. (2016). Water-in-oil Emulsion as a Simple Phantom for Validation of Double Diffusion Encoding MRI Sequences. Magn. Reson. Mater. Phy. 29, S48–S49, http://doi.org/10.1007/s10334-016-0568-x
11. Ulloa, P., Wottschel, V., & Koch, M. A. (2015). Studying the extracellular contribution to the double wave vector diffusion-weighted signal. Curr. Dir. Biomed. Engin. 1, 240–244. http://doi.org/10.1515/cdbme-2015-0060
12. Neeman, M., Freyer, J. P., & Sillerud, L. O. (1991). A simple method for obtaining cross-term-free images for diffusion anisotropy studies. NMR microimaging. Magn. Res. Med. 21, 138–143. http://doi.org/10.1002/mrm.1910210117
13. Mills, R. (1973). Self-diffusion in normal and heavy water in the range 1-45 deg. J. Phys. Chem. 77, 685–688. http://doi.org/10.1021/j100624a025
14. Lawrenz, M., & Finsterbusch, J. (2011). Detection of microscopic diffusion anisotropy on a whole-body MR system with double wave vector imaging. Magn. Reson. Med. 66, 1405–1415. http://doi.org/10.1002/mrm.22934
15. Jespersen, S. N. (2012). Equivalence of double and single wave vector diffusion contrast at low diffusion weighting. NMR in Biomed. 25, 813–818. http://doi.org/10.1002/nbm.1808
Figures