0199

B1+ inhomogeneity mitigation in CEST using parallel transmission: pTxCEST
Nuno André da Silva1, Desmond H. Y. Tse2, Benedikt A Poser2, and N Jon Shah1,3,4

1Institute of Neuroscience and Medicine - 4, Forschungszentrum Jülich, Jülich, Germany, 2Faculty of Psychology and Neuroscience, Maastricht University, Maastricht, Netherlands, 3Department of Neurology, Faculty of Medicine, RWTH Aachen University, JARA, Aachen, Germany, 4Department of Electrical and Computer Systems Engineering, and Monash Biomedical Imaging, School of Psychological Sciences, Monash University, Melbourne, Australia

Synopsis

To demonstrate the benefits from the increased spectral bandwidth at ultra-high field (UHF) by using parallel transmission (pTx) to mitigate flip-angle inhomogeneity in chemical exchange saturation transfer (CEST) imaging. A pTx basis pulse is homogenised by magnitude least-squares (MLS) optimisation and expanded to form a frequency-selective saturation pulse for CEST. The pTx saturation pulse was validated by both Bloch-McConnell simulation and in vivo imaging at 7T. Improved homogeneity in contrasts and relaxation-compensated CEST metrics were observed in our in vivo data when the pTx saturation pulse was used instead of the standard CP-mode Gaussian pulse.

Purpose

To demonstrate the benefits from the increased spectral bandwidth at ultra-high field (UHF) by using parallel transmission (pTx) to mitigate flip-angle inhomogeneity in chemical exchange saturation transfer (CEST) imaging.

Methods

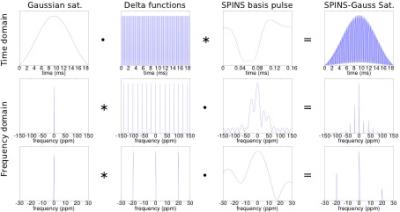
Flip-angle homogenised pTx basis pulse designed by magnitude least-squares (MLS) optimisation1,2,3 was expanded to form a frequency-selective pulse for CEST in a two-step process (Fig. 1). The saturation pulse was simulated with a three-pool Bloch-McConnell equation to evaluate its CEST contrast properties4. Experiments were performed on a 7T whole-body MR scanner (MAGNETOM, Siemens Healthineers, Erlangen, Germany) with an 8-channel transmit/32-channel receive head coil (Nova Medical, Wilmington, MA, USA). B0 and B1+ maps for pulse optimisation were obtained from a dual-echo 3D GRE and a transmit phase-encoded5, T2 and T2* compensated version of DREAM6, respectively. PreSat-TFL7 was used to map the flip-angle of the designed pulses8. In vivo CEST imaging performance of the pTx saturation pulse (pTxCEST) and the standard Gaussian saturation in CP-mode were compared. 2-spokes pTx homogeneous excitation was used to ensure fair comparison of the two saturation pulses9. A 2D GRE (single-shot centric-acquisition, TE=4.39ms, TR=9.2ms, matrix size = 80×80, flip-angle=10˚, slice-thickness=6.5mm) with a train of 120 saturation pulses (50% duty-cycle) was used as the CEST imaging sequence. Z-spectra were sampled from -300 to 300ppm at 69 unevenly distributed points using an effective power of 0.6µT 10. Magnetisation transfer ratio and inverse Z-spectrum analyses were used as metrics in evaluating the data from three healthy volunteers11.

Results

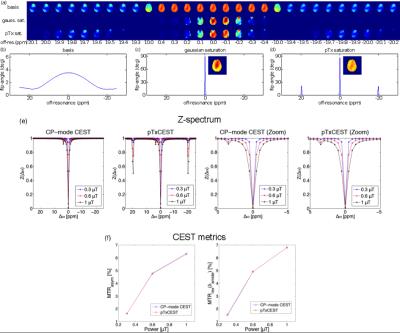
The results of Bloch simulations of the two saturation pulses, CP-mode CEST and pTxCEST, as well as the pTx basis pulse are presented in Fig. 2(a-d). Small side bands at ± 20 ppm can be seen in the pTxCEST saturation. The inserts in Fig. 2 (c) and (d) are the spatial distribution of the normalised flip-angle integrated over the central peak (± 0.5 ppm), which show a more spatially homogeneous saturation provided by the pTxCEST (NRMSE 0.042) in comparison to the CP-mode CEST pulse (NRMSE 0.122). Bloch-McConnell simulations (Fig. 2e-f) showed that side bands of the pTx saturation pulse at ± 20 ppm did not affect any CEST contrast. 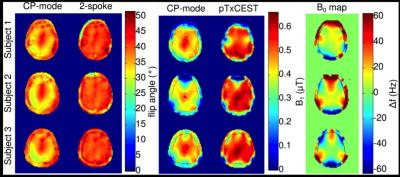The B0 off-resonance maps, the PreSat-TFL flip-angle maps of the CP-mode and 2-spokes excitations, as well as the CP-mode CEST and pTxCEST saturations at the position of the CEST imaging slice from three healthy volunteers are presented in Fig. 3. The average excitation NRMSE across the three volunteers reduced from 0.11 (CP-mode) to 0.05 (2-spokes). The CP-mode CEST saturation pulse only reached the desired 0.6 μT effective B1+ power in the central brightening spot, while the pTxCEST pulse managed to homogeneously deliver the desired saturation power across the entire brain region within the imaging slice. Fig. 4 shows the Z-spectra from both CP-mode CEST and pTxCEST saturations from two different locations in white matter. 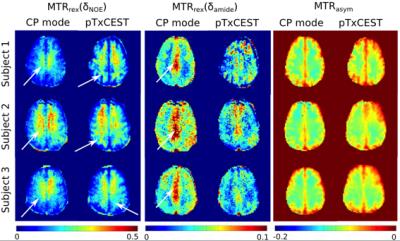The spectra from CP-mode CEST saturation display noticeable differences, caused by the B1+ inhomogeneity. CEST parametric maps are presented in Fig. 5. Undesired contrast variations, e.g. due to B1+ inhomogeneity rather than tissue difference, can be observed in the maps acquired with the CP-mode CEST saturation, but not in those with the pTxCEST saturation.

Discussion

This work presents a method to design a parallel-transmit saturation pulse for CEST to mitigate undesired contrast variations caused by the B1+ inhomogeneity at UHF. The homogeneous pTxCEST saturation pulse was evaluated by simulations as well as in vivo human brain imaging at 7T. The pTxCEST saturation was designed in two steps which combine the benefits of the spatially homogenised flip from the pTx basis pulse with the frequency selectivity of a Gaussian pulse, a typical choice for saturation in CEST experiments. While the pTx saturation preserves the bandwidth of the Gaussian envelope, it creates side bands as shown in Figs. 1-2. This leaves a usable range of ± 10 ppm, which exceeds the typical saturation range in CEST experiments using MTRasym and hence poses no practical constraints. Furthermore, no influence in CEST contrasts was found in the Bloch-McConnell simulations (Fig. 2). Both Bloch simulations (Fig. 2) and in vivo mapping (Fig. 3) show that pTxCEST provided spatially more homogeneous saturation than CP-mode, which translated into more reliable CEST contrast. This is indicated by the reduced variability in the white matter Z-spectra between ± 5 ppm (Fig. 4) when pTxCEST saturation was used. Also, the same improvements can be seen in the CEST parametric maps (Fig. 5).

Conclusion

A pTx-based pulsed CEST pre-saturation scheme is proposed and validated by simulations and 7T in vivo imaging.

Acknowledgements

We thank Christopher Wiggins for the helps on various technical issues at the scanner. Scan hours were funded under Scannexus/Brains Unlimited development project dev_b0_b1 and intramural MBIC funding projects (F8000C09 and F8015). NJS is funded in part by the Helmholtz Alliance ICEMED - Imaging and Curing Environmental Metabolic Diseases, through the Initiative and Network Fund of the Helmholtz Association. Further, NJS is supported in part by TRIMAGE, an EU FP7 project (grant agreement n° 602621)

References

1. Setsompop K, Wald LL, Alagappan V, Gagoski BA, Adalsteinsson E. Magnitude least squares optimization for parallel radio frequency excitation design demonstrated at 7 Tesla with eight channels. Magn Reson Med 2008; 59 (4):908-915

2. Grissom W, Yip C-y, Zhang Z, Stenger VA, Fessler JA, Noll DC. Spatial domain method for the design of RF pulses in multicoil parallel excitation. Magn Reson Med 2006; 56 (3):620-629

3. Sbrizzi A, Hoogduin H, Lagendijk JJ, Luijten P, Sleijpen GLG, van den Berg CAT. Time efficient design of multi dimensional RF pulses: Application of a multi shift CGLS algorithm. Magn Reson Med 2011; 66 (3):879-885

4. Jones CK, Polders D, Hua J, Zhu H, Hoogduin HJ, Zhou J, Luijten P, van Zijl PCM. In Vivo 3D Whole-Brain Pulsed Steady State Chemical Exchange Saturation Transfer at 7T. Magn Reson Med 2012; 67 (6):1579-1589

5. Tse DHY, Poole MS, Magill AW, Felder J, Brenner D, Shah NJ. Encoding methods for mapping in parallel transmit systems at ultra high field. J Magn Reson 2014; 245:125-132

6. Nehrke K, Versluis MJ, Webb A, Bornert P. Volumetric B1 (+) mapping of the brain at 7T using DREAM. Magn Reson Med 2014; 71 (1):246-256

7. Chung S, Kim D, Breton E, Axel L.Rapid B1+ mapping using a preconditioning RF pulse with TurboFLASH readout. Magn Reson Med 2010; 64 (2):439-446

8. Tse DHY, Wiggins CJ, Ivanov D, Brenner D, Hoffmann J, Mirkes C, Shajan G, Scheffler K, Uludag K, Poser BA. Volumetric imaging with homogenised excitation and static field at 9.4 T. Magn Reson Mater Phy 2016; 29 (3):333-345

9. Tse DHY, Wiggins CJ, Poser BA. High-resolution gradient-recalled echo imaging at 9.4T using 16-channel parallel transmit simultaneous multislice spokes excitations with slice-by-slice flip angle homogenization. Magn Reson Med 2016; 10.1002/mrm.26501

10. Zu Z, Li K, Janve VA, Does MD, Gochberg DF. Optimizing pulsed-chemical exchange saturation transfer imaging sequences. Magn Reson Med 2011; 66 (4):1100-1108

11. Windschuh J, Zaiss M, Meissner JE, Paech D, Radbruch A, Ladd ME, Bachert P. Correction of B1-inhomogeneities for relaxation-compensated CEST imaging at 7T. NMR Biomed 2015; 28:529-537

12. Malik SJ, Keihaninejad S, Hammers A, Hajnal JV. Tailored excitation in 3D with spiral nonselective (SPINS) RF pulses. Magn Reson Med 67 2012; (5):1303-1315

13. Zhou J, Lal B, Wilson DA, Laterra J, Zijl PCMv. Amide proton transfer (APT) contrast for imaging of brain tumors. Magn Reson Med 2003; 50:1120-1126

14. Xu J, Zaiss M, Zu Z, Li H, Xie J, Gochberg DF, Bachert P, Gore JC. On the origins of chemical exchange saturation transfer (CEST) contrast in tumors at 9.4T. NMR Biomed 2014; 27 (4):406-416

15. Zaiss M, Bachert P. Exchange-dependent relaxation in the rotating frame for slow and intermediate exchange modelling off-resonant spin-lock and chemical exchange saturation transfer. NMR Biomed 2013; 26 (5):507-518


Figures

Fig.1: Schematic of the two-step pTx saturation pulse formation in the time and frequency domains. The bottom row is the same pulse plotted in a frequency range of ± 30 ppm. First, a basis pulse using the spiral non-selective (SPINS)12 trajectory is designed by the MLS optimisation to yield a spatially homogeneous flip-angle. Second, the frequency selectivity of a long-duration Gaussian pulse and the spatial homogeneity of the basis pulse are combined by repeating the basis pulse for the same period of time as the Gaussian pulse and imposing the profile of the Gaussian pulse onto the resulting composite pulse.

Fig.2:(a-d) Saturation pulse Bloch simulations: (a) Flip-angle maps of the pTx basis pulse, the CP-mode CEST and the pTxCEST saturation pulses at different frequencies. (b-d) Flip-angle averaged over the volume-of-interest in a frequency range of ± 25 ppm for the basis pTx pulse, the CP-mode CEST and the pTxCEST saturation pulse. The inserts in (c) and (d) show the flip-angle maps of the central peak (± 0.5 ppm). (e) Bloch-McConnell simulations of Z-spectra for both CP-mode CEST and pTxCEST saturations at different effective B1+ powers. (f) CEST contrasts evaluated in metrics MTRasym and MTRrex over the effective B1+ amplitude range.

Fig. 3: PreSat-TFL flip-angle maps of the excitations (left) and saturations (centre), as well as B0 off-resonance maps (right) acquired in 3 healthy volunteers. The slice location corresponds to the CEST imaging slice. Flip-angle maps of the excitation pulses demonstrate the homogeneous excitations provided by the 2-spoke pulse, which successfully eliminates the central brightening that is seen in the CP-mode excitation. The pTxCEST pulse managed to homogeneously deliver the desired saturation power across the entire brain region within the imaging slice. As a property of the frequency-selective Gaussian pulse, regions with B­0 off-resonance over ± 0.5 ppm were not saturated

Fig. 4: Z-spectra obtained with CP-mode CEST and pTxCEST saturation pulses, at the two different white matter locations indicated in the brain image on the left. As predicted by Bloch-McConnell simulations (Fig. 2d-e), reductions in signal can be seen at ± 20 ppm for the pTxCEST saturation due to its side bands. Within the typical frequency range for evaluating MTRasym, i.e. ± 5 ppm, no effect of the side bands can be observed. B1+ inhomogeneity in the CP-mode saturation led to different effective B1+ saturation powers in the two voxels, resulting in differences between the two white matter CP-mode spectra

Fig. 5: CEST parametric maps from the CP-mode CEST and pTxCEST saturation schemes. White arrows highlight the areas where pTxCEST showed improvements over CP-mode CEST saturation. MTRasym is given by the difference of the signals at the amide frequency (3.5 ppm) and at the opposite side of the water frequency (-3.5 ppm)13. The metric MTRrex is defined as MTRrex(δi)= 1/Zlab(δi) - 1/Zref(δi), where δi = 3.5 ppm for amide MTRrex, δi = -3.5 ppm for NOE MTRrex, and the reference 1/Zref is the sum of all fitted Lorentzian functions except the labelled species14,15.

Proc. Intl. Soc. Mag. Reson. Med. 25 (2017)
0199