Introduction

Safety limits based on the specific absorption rate (SAR) in MRI are typically derived from numerical electromagnetic (EM) simulations of a set of human body models. Most available models are discretized voxel-based geometries that are simulated by EM time-domain solvers, which do not ensure electrical contact between anatomically connected parts of tissues. This problem can be solved if an anatomically correct surface-based human model is simulated using a tetrahedral-mesh-based solver. However, it is a challenge to convert voxel-based geometries (as obtained, e.g., from MRI scans) to high-quality surface-based objects with proper matching in contact regions. Another actual restriction is that progress in 3D EM tetrahedral-mesh-based solver development got ahead of geometry import, pre-processing, and mesh generation capabilities. Thus, the surface-based model should be limited to about 2,000,000 faces. Only a few surface-based human models are available¹. Our goals in this study were: (a) to build a prototype of a high resolution surface-based human head model that can be simulated in a reasonable time; (b) to evaluate the influence of cerebrospinal fluid (CSF) on field propagation estimates of traveling wave excitation at 297.2 and 400 MHz.

Method

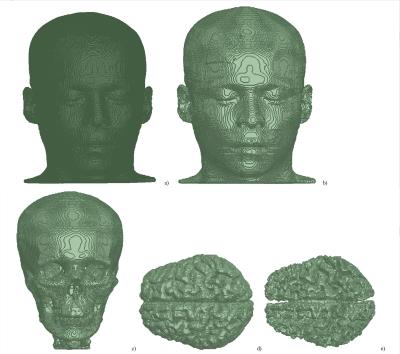
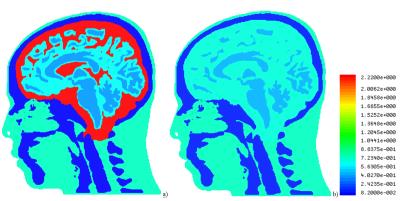
Five objects—primary intracranial tissues (grey matter, GM, white matter, WM, and CSF; Fig. 1) as well as skull and skin—were obtained from a manually segmented anatomical dataset with 1×1×1 mm³ spatial resolution. The anatomical input data were taken from previous work^2,3 and consisted of a CT scan to derive skin and skull and an MRI scan to derive the other tissues. The convex hull of each tissue boundary within the segmented dataset was calculated. The model is an assembly of the resulting surfaces nested into each other. The skin object’s electrical properties were assumed to equal those from muscle. Extracranial tissues have comparable electrical properties and were not segmented separately. The surface meshes describing the geometry of each object were then converted into stl format and imported into ANSYS HFSS 2014 as solid 3D objects. To reduce the amount of faces, all neighboring triangular faces located at the same geometrical plane were combined into one face (Fig. 1), which reduced the number of faces eightfold to approx. 1,000,000 faces. To study RF transmission, traveling-wave excitations at 297.2 MHz and 400 MHz were applied to the top of the head. Electrical properties of tissues were adopted from the IT’IS database⁴. The CSF electrical properties were modified to either e=72.73 and s=2.22 S/m, or e=60 and s=0.69 S/m. The latter values mimic a model without inclusion of a CSF object in the numerical domain by setting its electrical properties to those from GM (Fig. 2). All results were scaled to 1W transmit power.

Results and Discussion

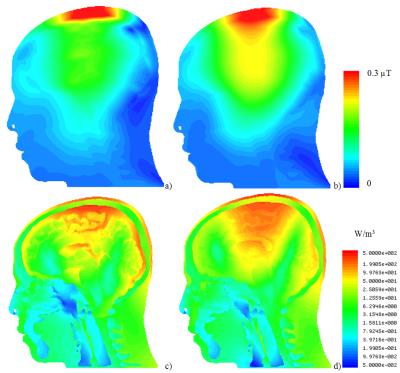
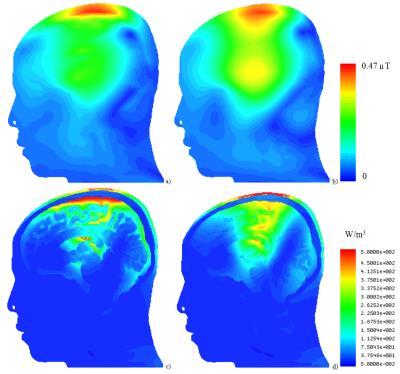
For both excitation frequencies, CSF acted as a weak RF screen (Figs. 3 and 4), resulting in (i) a substantial drop of B1+ in GM and WM and (ii) in a significant redistribution of volume loss density. Concomitantly, power deposition increased in the top part of the scalp. Because blood perfusion in the scalp is relatively low, local overheating becomes a safety concern, especially at 400 MHz. The current model includes high-resolution objects only in the intracranial volume whereas no separation of different tissues was attempted in other regions. For simulation of traveling-wave excitation, this is not a relevant simplification; however, for simulations of head arrays it will be necessary to include the upper body parts and to segment cerebellum, brain stem, and air cavities. The time required for geometry import, pre-processing, and mesh generation was tenfold longer than the solver time of approx. 1 hour on an up-to-date Dell workstation. This is too long for real-time safety assessment, however, it is reasonable for investigating SAR dependences on intracranial geometry variation (e.g. variation of CSF spaces with age).

Conclusion

Combining neighboring triangular faces located in the same plane into a single one is an approach that achieves simulations of high-resolution human models previously not accessible to tetrahedral-mesh-based solvers. If electrical contact between anatomically connected parts of CSF is correctly considered, CSF was found to partially shield brain tissues from the incident RF field. This results in a decrease of B₁+ inside the brain and an increase in the power deposited in the scalp. Although the particular results of this study cannot be readily generalized to the wider human population, the use of individual MRI scans as input data can be readily extended to studying other realistic anatomical variations.

Acknowledgements

We thank Don Tucker, Sergei Turovets, Phan Luu, and Chelsea Mattson from Electrical Geodesics, Inc. for proving the multimodal imaging data.