4578
Accelerating Three-Dimension Balanced Steady-State Free Precession Imaging with Modified Wave-CAIPI Technique1Lauterbur Research Center for Biomedical Imaging, Shenzhen Institutes of Advanced Technology, Chinese Academy of Sciences, Shenzhen, China, 2Research Center for Medical AI, Shenzhen Institutes of Advanced Technology, Chinese Academy of Sciences, Shenzhen, China
Synopsis
Balanced steady-state free precession (bSSFP) has merits such as high signal-to-noise ratio, T2/T1 contrast and rapid acquisition speed. However, bSSFP requires further acceleration in 3D imaging due to massive data collected. The acceleration of conventional parallel imaging techniques is limited. In this study, we propose wave-bSSFP by using a modified wave-CAIPI technique to highly accelerate bSSFP. Wave gradients were truncated to further reduce g-factor noise penalty with high wave amplitudes. The simulation and in vivo experiment indicate that wave-bSSFP is effective in decreasing g-factor. Here, an acceleration factor of 9 was achieved in brain scan with 0.8 mm isotropic resolution.
Introduction
Balanced steady-state free precession (bSSFP) [1, 2] has been widely used in various clinical applications of magnetic resonance imaging (MRI). Due to its balanced gradient along all axes within each repetition time, bSSFP intrinsically provides a high signal-to-noise ratio (SNR) and a unique T2/T1 tissue contrast, and rapid acquisition speed. In 3D imaging where massive data are acquired, conventional parallel techniques are used to accelerate bSSFP, while the acceleration factors are limited. To achieve further accelerations, we develope wave-bSSFP by using the wave-CAIPI technique [3] to highly accelerate bSSFP. Instead of conventional wave-CAIPI technique that uses extended wave gradients [3], the beginning and end of wave gradients were truncated in our work. This may facilitate the application of large-amplitude wave gradient without overstepping the gradient slew-rate limitation of the system. Therefore, further reduction in g-factor noise penalty [4] can be achieved without elongating both TE and TR due to gradients extension. The simulation and in vivo experiment indicate that the proposed method could effectively decrease g-factor at high acceleration factors.Methods
Pulse Sequence: The proposed sequence diagram of wave-bSSFP is developed as seen as Figure 1. Wave gradients of sinusoidal waveforms with phase shift of π/2 are applied in phase and slice direction. To obtain maximal wave amplitude as well as not exceed the gradient slew-rate limitation, the sinusoidal wave gradient in slice direction with initial phase of π/2 is truncated by one cycle. This allows gradients to moderately ramp.
Simulation: The simulation was conducted to evaluate the effectiveness of modified wave gradients in g-factor reduction. Wave-CAIPI reconstructions with truncated and extended wave gradients were simulated based on fully sampled phantom data (acceleration factor of 3×3). And, simulation data was acquired on Siemens 3T MR system (MAGNETOM Trio, Siemens AG, Erlangen, Germany) using bSSFP sequence with a 32-channel head coil. The scan parameters were: TE = 3.43 ms, TR = 6.86 ms, flip angle = 30°, bandwidth = 298 Hz/pixel, matrix size = 192×192×192, voxel size = 1×1×1 mm3. With respect to the limitation of our MR system on gradient slew-rate (maximal 200 mT/m·ms), a small wave amplitude of 2 mT/m with wave cycles of 7 were used in the simulation.
In Vivo Experiment: Whole brain was also scanned on the same Siemens 3T MR system. IRB-approved healthy subjects were enrolled in the experiments. The 32-channel head coil was used in the brain experiment, and point spread functions were estimated using two-dimension projection data [5]. Wave amplitude was chosen as 12 mT/m with wave cycles of 7, and an acceleration factor of 3×3 was used. Other scan parameters were: TE = 3.43 ms, TR = 6.86 ms, flip angle = 30°, bandwidth = 298 Hz/pixel, matrix size = 240×240×240, voxel size = 0.8×0.8×0.8 mm3.
Image Reconstruction: Reconstructions were implemented using MATLAB (Mathworks, Natick, MA, USA) for both simulation and in vivo experiments, and retrospective accelerations were applied. The sensitivity maps were estimated using the center 48×48 ACS lines with the ESPIRiT software package [6].
Results
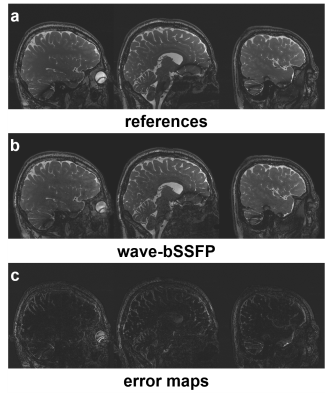
The experimental results show that the truncated wave gradients of the proposed wave-bSSFP are also able to effectively decrease g-factor penalty (Figure 2b). Moreover, its reconstructed image quality and 1/g-factor maps are similar to that of wave-CAIPI technique using conventional extended wave gradients (Figure 2b&c). With truncated wave gradients, wave-bSSFP achieves an acceleration factor of 9 and 0.8 mm isotropic resolution with small g-factor noise penalty (Figure 3b). Reconstructed images also exhibit comparable quality to that of fully sampled bSSFP results (Figure 3a).Discussion
BSSFP usually has large readout amplitudes due to short TE and TR used to avoid banding artifacts. This leads to limited g-factor reduction for conventional wave-CAIPI technique [3], because of reducing g-factor with the increased ratio of wave amplitude to readout amplitude [4]. Previous studies also used extended sinusoidal wave gradient to obtain large wave gradients. However, this method would elongate both TE and TR. For bSSFP sequence in which short TE and TR are required, previous method of extending sinusoidal wave gradients is not suitable. In order to obtain large gradient amplitude to increase acceleration factor, we proposed truncated wave gradients to be used in wave-bSSFP. It had been demonstrated to allow large wave gradients under the system limitation.Conclusion
Highly accelerated three-dimension bSSFP has been achieved by the proposed wave-bSSFP technique. The truncated wave gradient allows application with larger amplitudes than the conventional wave gradients, which can benefit g-factor reduction as well as SNR compensation. In the future, the proposed wave-bSSFP will be applied into the clinical applications of bSSFP sequence.Acknowledgements
This work was supported in part by the grant from the National Science Foundation of China (61871373, 61471350, 81729003), Guangdong Provincial Key Laboratory of Medical Image Processing (2017A050501026), and the National Science Foundation of Guangdong Province (2018A0303130132).References
[1] Carr, H., Steady-state free precession in nuclear magnetic resonance[J]. Physical Review, 1958. 112(5): p. 1693.
[2] Oppelt, A., R. Graumann, H. Barfuss, H. Fischer, W. Hartl, and W. Schajor, FISP—a new fast MRI sequence[J]. Electromedica, 1986. 54(1): p. 15-18.
[3] Bilgic, B., B.A. Gagoski, S.F. Cauley, A.P. Fan, J.R. Polimeni, P.E. Grant, L.L. Wald, and K. Setsompop, Wave‐CAIPI for highly accelerated 3D imaging[J]. Magnetic resonance in medicine, 2015. 73(6): p. 2152-2162.
[4] Zhilang Qiu, Haifeng Wang, Leslie Ying, Xin Liu, Dong Liang, Parameter Optimization of Wave-CAIPI Based on Theoretical Analysis[C]. Proc. Intl. Soc. Mag. Reson. Med. 26 (2018).
[5] Duyn J H, Yang Y, Frank J A, et al. Simple correction method for k-space trajectory deviations in MRI[J]. Journal of Magnetic Resonance, 1998, 132(1): 150-153.
[6] Uecker, M., P. Lai, M.J. Murphy, P. Virtue, M. Elad, J.M. Pauly, S.S. Vasanawala, and M. Lustig, ESPIRiT—an eigenvalue approach to autocalibrating parallel MRI: where SENSE meets GRAPPA[J]. Magnetic resonance in medicine, 2014. 71(3): p. 990-1001.
Figures