0943
HYFI: Hybrid filling of the dead-time gap for faster zero echo time imaging1ETH Zurich and University of Zurich, Zürich, Switzerland
Synopsis
MRI of tissues with short transverse relaxation times can be performed efficiently with zero echo time sequences. However, in situation involving large dead time gaps, current established techniques such as algebraic ZTE, PETRA or WASPI are not optimal because of either point spread function-related artifacts or low SNR efficiency. In this work, a novel ZTE-based MRI technique is proposed. It employs a hybrid encoding scheme with both Cartesian and radial acquisitions. In this way, SNR efficiency is improved while preserving image quality as compared to existing methods.
Introduction
MRI of tissues with short transverse relaxation times can be performed efficiently with zero echo time (ZTE) sequences1. The characteristics of these sequences is that the readout gradient is switched on before spin excitation and that data is acquired as soon as possible after the end of the radiofrequency pulse. The delay separating spin excitation and acquisition is referred to as dead time and includes half the pulse duration, the transmit-receive switch time of the MRI scanner and the filter group delay. Since the dead time prevents acquisition of data in central k-space, missing information must be recovered subsequently. To do so, several established sequences are commonly used: algebraic ZTE1, PETRA2 and WASPI3. They all give satisfactory results when only a few Nyquist dwells are missed4. However, the dead time may reach several tens of microseconds leading to relatively large gaps (tens of Nyquist dwells), especially at high bandwidths as required for high-resolution imaging of fast relaxing spins5. In such situations, the algebraic approach is not feasible because of ill-conditioned reconstruction, WASPI suffers from serious artifacts arising from oscillatory point spread functions, and the efficiency with respect to signal-to-noise ratio (SNR) of PETRA decreases due to many single-point imaging (SPI) acquisitions4.
The idea of the presented method is to increase the SNR efficiency as compared to PETRA while preserving image quality. To do so, the k-space gap is filled in a hybrid manner with a Cartesian core surrounded by radial shells.
In this work we present the HYFI principle and demonstrate its capability by imaging of a rubber phantom, a sample of ovine bone and the head of a health human subject.
Methods
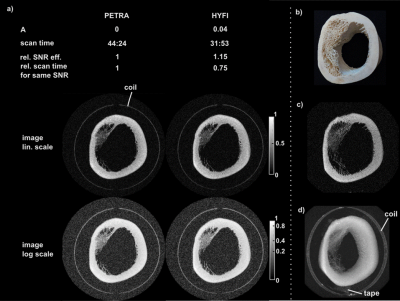
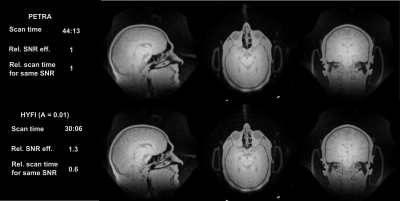
Figure 1 illustrates the basics of ZTE-based sequences. PETRA, WASPI, and HYFI differ in the way they recover or complement the data missed during the dead-time gap . In PETRA (Fig. 1c), the inner k-space is acquired single-point-wise in a Cartesian fashion leading to constant T2* weighting. In WASPI (Fig. 1d), a second set of radial acquisitions is performed at lower gradient strength, giving rise to potentially strong exponential T2* weighting. In HYFI (Fig. 1e), a combination of Cartesian SPI and radial acquisitions is used to limit the T2* decay inside the gap to a selected range. This range is defined by the amplitude factor A which describes the relative signal loss occurring during the readout following an excitation. It assumes a targeted T2* which corresponds to the transverse relaxation time of the tissue of interest and is chosen such as to maximize SNR efficiency with minimum loss of image quality. The targeted T2 was set to 100 us for rubber imaging and to 200 us for both bone and head respectively. Experiments were performed on 3T (bone and human head) and 7T (rubber) Philips Achieva MRI systems (Philips Healthcare, Best, Netherlands), using 1H-free surface coils6 and symmetrically biased transmit-receive switches7. Data was acquired with a custom-made spectrometer8. Images were reconstructed with a conjugate gradient algorithm9.
The SNR, the SNR efficiency (SNReff), the relative SNR efficiency (relSNReff) and the scan time for same SNR (tSNR) are calculated as follow:
SNR=Sσ,SNReff=SNR√t,relSNR=SNReffSNReffPETRA,tSNR=(1relSNReff)2
with S being the average signal over a region of interest (ROI), σ the standard deviation of a simulated noise acquisition in the same ROI, t the scan time and SNReffPETRA the SNR efficiency of PETRA.
Results
Figure 2 shows the number of excitations (N) that needs to be performed to fill a k-space sphere of radius = gap according to Nyquist criterion. The advantage of radial versus Cartesian geometry increases with gap size.
Figure 3 illustrates the HYFI principle and in particular the influence of the amplitude factor A. When A increases from 0 to 1, the relative number of required excitations, N/NPETRA, decreases substantially, allowing faster imaging. Moreover, the image quality is preserved at low amplitude factor (A <= 0.2) but artifacts start to appear if a too large A is chosen.
Figure 4 and 5 display comparisons between PETRA and HYFI for high-bandwidth imaging. For the same SNR and comparable image quality, HYFI is about 25-40% faster than PETRA.
Discussion and conclusion
HYFI, a novel ZTE-based MRI technique was proposed employing a hybrid encoding scheme with both Cartesian and radial acquisitions. In this way, SNR efficiency is improved while preserving image quality as compared to existing related methods. Its SNR advantage increases with gap size which makes it particularly suitable for imaging situations involving large dead times, as associated with high-bandwidth or introduced to achieve T2* selectivity or reduce image blurring. Moreover, patient comfort is improved by largely replacing SPI by radial acquisitions as reduced gradient switching significantly lowers vibrations and acoustic noise.Acknowledgements
No acknowledgement found.References
1. Weiger M, Pruessmann KP. MRI with Zero Echo Time. eMagRes. 2012;1:311–322.
2. Grodzki DM, Jakob PM, Heismann B. Ultrashort echo time imaging using pointwise encoding time reduction with radial acquisition (PETRA). Magnetic Resonance in Medicine. 2012;67:510–518.
3. Wu Y, Dai G, Ackerman JL, Hrovat MI, Glimcher MJ, Snyder BD, Nazarian A, Chesler D a. Water- and fat-suppressed proton projection MRI (WASPI) of rat femur bone. Magnetic resonance in medicine. 2007;57:554–67.
4. Froidevaux R, Weiger M, Brunner DO, Dietrich BE, Wilm BJ, Pruessmann KP. Filling the dead-time gap in zero echo time MRI: Principles compared. Magnetic Resonance in Medicine. 2018;79:2036–2045.
5. Froidevaux R, Weiger M, Rösler MB, Brunner DO, Dietrich BE, Reber J, Pruessmann KP. Pushing the limits of short-T2 MRI: 200 mT/m gradient strength and 2 MHz bandwidth. Proceedings of the 27th Annual Meeting ISMRM, Paris. 2018.
6. Rösler MB, Weiger M, Brunner DO, Schmid T, Pruessmann KP. An RF birdcage coil designed for an insert gradient coil dedicated to short-T2 MRI. In Proceedings of the 26th Annual Meeting of ISMRM, Honolulu. 2017:2668.
7. Brunner DO, Furrer L, Weiger M, Baumberger W, Schmid T, Reber J, Dietrich BE, Wilm BJ, Froidevaux R, Pruessmann KP. Symmetrically Biased T/R Switches for NMR and MRI with Microsecond Dead Time. Journal of Magnetic Resonance. 2016;263:147–155.
8. Dietrich BE, Brunner DO, Wilm BJ, Barmet C, Gross S, Kasper L, Haeberlin M, Schmid T, Vannesjo SJ, Pruessmann KP. A field camera for MR sequence monitoring and system analysis. Magnetic Resonance in Medicine. 2015;00:n/a-n/a.
9. Pruessmann KP, Weiger M, Bo P, Boesiger P. Advances in Sensitivity Encoding With Arbitrary k -Space Trajectories. 2001;651:638–651.
Figures